Longest uncrossed knight's path
The longest uncrossed (or nonintersecting) knight's path is a mathematical problem involving a knight on the standard 8×8 chessboard or, more generally, on a square n×n board. The problem is to find the longest path the knight can take on the given board, such that the path does not intersect itself. A further distinction can be made between a closed path, which ends on the same field as where it begins, and an open path, which ends on a different field from where it begins.
Known solutions
The longest open paths on an n×n board are known only for n ≤ 9. Their lengths for n = 1, 2, …, 9 are:
The longest closed paths are known only for n ≤ 10. Their lengths for n = 1, 2, …, 10 are:

|
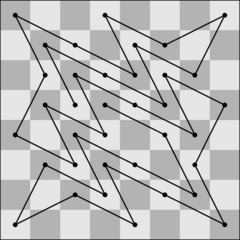
|
| The longest closed path for n = 7 of length 24. |
The longest open path for n = 8 of length 35. |
Generalizations
The problem can be further generalized to rectangular n×m boards, or even to boards in the shape of any polyomino. The problem for n×m boards, where n doesn't exceed 8 and m might be very large was given at 2018 ICPC World Finals. The solution used dynamic programming and uses the fact that the solution should exhibit a cyclic behavior.
Other standard chess pieces than the knight are less interesting, but fairy chess pieces like the camel ((3,1)-leaper), giraffe ((4,1)-leaper) and zebra ((3,2)-leaper) lead to problems of comparable complexity.
See also
- A knight's tour is a self-intersecting knight's path visiting all fields of the board.
- TwixT, a board game based on uncrossed knight's paths.
References
- L. D. Yarbrough (1968). "Uncrossed knight's tours". Journal of Recreational Mathematics 1 (3): 140–142.
- George Jelliss, Non-Intersecting Paths
- Non-crossing knight tours
- 2018 ICPC World Finals solutions (Problem J)
External links
 |

