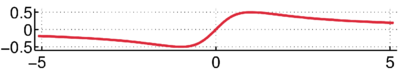
Serpentine curve
From HandWiki
A serpentine curve is a curve whose equation is of the form
- [math]\displaystyle{ x^2y+a^2y-abx=0, \quad ab \gt 0. }[/math]
Equivalently, it has a parametric representation
- [math]\displaystyle{ x=a\cot(t) }[/math], [math]\displaystyle{ y=b\sin (t)\cos(t), }[/math]
or functional representation
- [math]\displaystyle{ y=\frac{abx}{x^2+a^2}. }[/math]
The curve has an inflection point at the origin. It has local extrema at [math]\displaystyle{ x = \pm a }[/math], with a maximum value of [math]\displaystyle{ y=b/2 }[/math] and a minimum value of [math]\displaystyle{ y=-b/2 }[/math].
History
Serpentine curves were studied by L'Hôpital and Huygens, and named and classified by Newton.
Visual appearance
External links
 |