Ailles rectangle
The Ailles rectangle is a rectangle constructed from four right-angled triangles which is commonly used in geometry classes to find the values of trigonometric functions of 15° and 75°.[1] It is named after Douglas S. Ailles who was a high school teacher at Kipling Collegiate Institute in Toronto.[2][3]
Construction
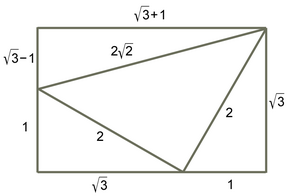
A 30°–60°–90° triangle has sides of length 1, 2, and [math]\displaystyle{ \sqrt{3} }[/math]. When two such triangles are placed in the positions shown in the illustration, the smallest rectangle that can enclose them has width [math]\displaystyle{ 1+\sqrt{3} }[/math] and height [math]\displaystyle{ \sqrt{3} }[/math]. Drawing a line connecting the original triangles' top corners creates a 45°–45°–90° triangle between the two, with sides of lengths 2, 2, and (by the Pythagorean theorem) [math]\displaystyle{ 2\sqrt{2} }[/math]. The remaining space at the top of the rectangle is a right triangle with acute angles of 15° and 75° and sides of [math]\displaystyle{ \sqrt{3}-1 }[/math], [math]\displaystyle{ \sqrt{3}+1 }[/math], and [math]\displaystyle{ 2\sqrt{2} }[/math].
Derived trigonometric formulas
From the construction of the rectangle, it follows that
- [math]\displaystyle{ \sin 15^\circ = \cos 75^\circ = \frac{\sqrt3 - 1}{2\sqrt2} = \frac{\sqrt6 - \sqrt2} 4, }[/math]
- [math]\displaystyle{ \sin 75^\circ = \cos 15^\circ = \frac{\sqrt3 + 1}{2\sqrt2} = \frac{\sqrt6 + \sqrt2} 4, }[/math]
- [math]\displaystyle{ \tan 15^\circ = \cot 75^\circ = \frac{\sqrt3 - 1}{\sqrt3 + 1} = \frac{(\sqrt3 - 1)^2}{3 - 1} = 2 - \sqrt3, }[/math]
and
- [math]\displaystyle{ \tan 75^\circ = \cot 15^\circ = \frac{\sqrt3 + 1}{\sqrt3 - 1} = \frac{(\sqrt3 + 1)^2}{3 - 1} = 2 + \sqrt3. }[/math]
Variant
An alternative construction (also by Ailles) places a 30°–60°–90° triangle in the middle with sidelengths of [math]\displaystyle{ \sqrt{2} }[/math], [math]\displaystyle{ \sqrt{6} }[/math], and [math]\displaystyle{ 2\sqrt{2} }[/math]. Its legs are each the hypotenuse of a 45°–45°–90° triangle, one with legs of length [math]\displaystyle{ 1 }[/math] and one with legs of length [math]\displaystyle{ \sqrt{3} }[/math].[4][5] The 15°–75°–90° triangle is the same as above.
See also
References
- ↑ Ravi Vakil (January 1996). A Mathematical Mosaic: Patterns & Problem Solving. Brendan Kelly Publishing Inc.. pp. 87–. ISBN 978-1-895997-04-0. https://archive.org/details/mathematicalmosa0000vaki. "ailles rectangle."
- ↑ Charles P. McKeague; Mark D. Turner (1 January 2016). Trigonometry. Cengage Learning. pp. 124–. ISBN 978-1-305-65222-4. https://books.google.com/books?id=xitTCwAAQBAJ&dq=ailles+rectangle&pg=PA124.
- ↑ DOUGLAS S. AILLES (1 October 1971). "Triangles and Trigonometry". The Mathematics Teacher 64 (6): 562. doi:10.5951/MT.64.6.0562. https://www.jstor.org/stable/27958618. Retrieved 2021-07-22.
- ↑ "Third Ailles Rectangle". Stack Exchange. 11 February 2016. https://math.stackexchange.com/q/1651208.
- ↑ Colin Beveridge (31 August 2015). "The Mathematical Ninja and Ailles' Rectangle". http://www.flyingcoloursmaths.co.uk/the-mathematical-ninja-and-ailles-rectangle/.
 |