Crossbar theorem
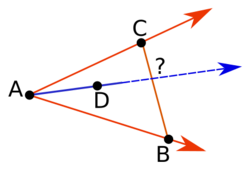
In geometry, the crossbar theorem states that if ray AD is between ray AC and ray AB, then ray AD intersects line segment BC.[1]
This result is one of the deeper results in axiomatic plane geometry.[2] It is often used in proofs to justify the statement that a line through a vertex of a triangle lying inside the triangle meets the side of the triangle opposite that vertex. This property was often used by Euclid in his proofs without explicit justification.[3]
Some modern treatments (not Euclid's) of the proof of the theorem that the base angles of an isosceles triangle are congruent start like this: Let ABC be a triangle with side AB congruent to side AC. Draw the angle bisector of angle A and let D be the point at which it meets side BC. And so on. The justification for the existence of point D is the often unstated crossbar theorem. For this particular result, other proofs exist which do not require the use of the crossbar theorem.[4]
See also
Notes
- ↑ Greenberg 1974, p. 69
- ↑ Kay 1993, p. 122
- ↑ Blau 2003, p. 135
- ↑ Moise 1974, p. 70
References
- Blau, Harvey I. (2003), Foundations of Plane Geometry, Upper Saddle River, NJ: Prentice Hall, ISBN 0-13-047954-3
- Greenberg, Marvin J. (1974), Euclidean and Non-Euclidean Geometries, San Francisco: W. H. Freeman, ISBN 0-7167-0454-4
- Kay, David C. (1993), College Geometry: A Discovery Approach, New York: HarperCollins, ISBN 0-06-500006-4
- Moise, Edwin E. (1974), Elementary Geometry from an Advanced Standpoint (2nd ed.), Reading, MA: Addison-Wesley, ISBN 0-201-04793-4
 |