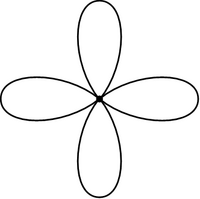
Bouquet graph

[math]\displaystyle{ B_4 }[/math], a bouquet with one vertex and four self-loop edges

In mathematics, a bouquet graph [math]\displaystyle{ B_m }[/math], for an integer parameter [math]\displaystyle{ m }[/math], is an undirected graph with one vertex and [math]\displaystyle{ m }[/math] edges, all of which are self-loops. It is the graph-theoretic analogue of the topological bouquet, a space of [math]\displaystyle{ m }[/math] circles joined at a point. When the context of graph theory is clear, it can be called more simply a bouquet.

Ribbon graph representation of an embedding of [math]\displaystyle{ B_3 }[/math] onto the projective plane.

Although bouquets have a very simple structure as graphs, they are of some importance in topological graph theory because their graph embeddings can still be non-trivial. In particular, every cellularly embedded graph can be reduced to an embedded bouquet by a partial duality applied to the edges of any spanning tree of the graph, or alternatively by contracting the edges of any spanning tree.

In graph-theoretic approaches to group theory, every Cayley–Serre graph (a variant of Cayley graphs with doubled edges) can be represented as the covering graph of a bouquet.