Cone-shape distribution function
The cone-shape distribution function, also known as the Zhao–Atlas–Marks time-frequency distribution,[1] (acronymized as the ZAM [2][3][4] distribution[5] or ZAMD[1]), is one of the members of Cohen's class distribution function.[1][6] It was first proposed by Yunxin Zhao, Les E. Atlas, and Robert J. Marks II in 1990.[7] The distribution's name stems from the twin cone shape of the distribution's kernel function on the [math]\displaystyle{ t, \tau }[/math] plane.[8] The advantage of the cone kernel function is that it can completely remove the cross-term between two components having the same center frequency. Cross-term results from components with the same time center, however, cannot be completely removed by the cone-shaped kernel.[9][10]
Mathematical definition
The definition of the cone-shape distribution function is:
- [math]\displaystyle{ C_x(t, f)=\int_{-\infty}^{\infty}\int_{-\infty}^{\infty}A_x(\eta,\tau)\Phi(\eta,\tau)\exp (j2\pi(\eta t-\tau f))\, d\eta\, d\tau, }[/math]
where
- [math]\displaystyle{ A_x(\eta,\tau)=\int_{-\infty}^{\infty}x(t+\tau /2)x^*(t-\tau /2)e^{-j2\pi t\eta}\, dt, }[/math]
and the kernel function is
- [math]\displaystyle{ \Phi \left(\eta,\tau \right) = \frac{\sin \left(\pi \eta \tau \right)}{ \pi \eta \tau }\exp \left(-2\pi \alpha \tau^2 \right). }[/math]
The kernel function in [math]\displaystyle{ t, \tau }[/math] domain is defined as:
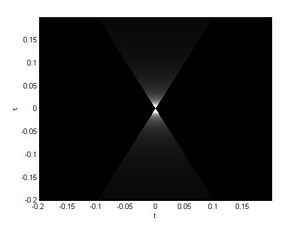
- [math]\displaystyle{ \phi \left(t,\tau \right) = \begin{cases} \frac{1}{\tau} \exp \left(-2\pi \alpha \tau^2 \right), & |\tau | \ge 2|t|, \\ 0, & \mbox{otherwise}. \end{cases} }[/math]
Following are the magnitude distribution of the kernel function in [math]\displaystyle{ t, \tau }[/math] domain.
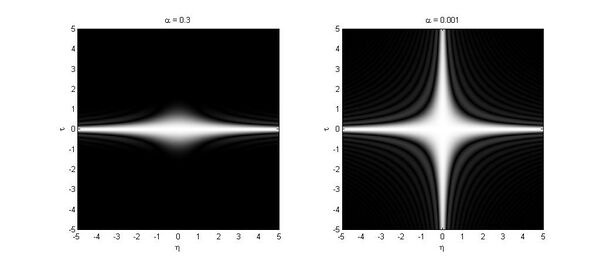
Following are the magnitude distribution of the kernel function in [math]\displaystyle{ \eta, \tau }[/math] domain with different [math]\displaystyle{ \alpha }[/math] values.
As is seen in the figure above, a properly chosen kernel of cone-shape distribution function can filter out the interference on the [math]\displaystyle{ \tau }[/math] axis in the [math]\displaystyle{ \eta, \tau }[/math] domain, or the ambiguity domain. Therefore, unlike the Choi-Williams distribution function, the cone-shape distribution function can effectively reduce the cross-term results form two component with same center frequency. However, the cross-terms on the [math]\displaystyle{ \eta }[/math] axis are still preserved.
The cone-shape distribution function is in the MATLAB Time-Frequency Toolbox[11] and National Instruments' LabVIEW Tools for Time-Frequency, Time-Series, and Wavelet Analysis [12]
See also
- Cohen's class distribution function
- Choi-Williams distribution function
- Wigner distribution function
- Ambiguity function
- Short-time Fourier transform
References
- ↑ 1.0 1.1 1.2 Leon Cohen, Time Frequency Analysis: Theory and Applications, Prentice Hall, (1994)
- ↑ L.M. Khadra; J. A. Draidi; M. A. Khasawneh; M. M. Ibrahim. (1998). "Time-frequency distributions based on generalized cone-shaped kernels for the representation of nonstationary signals". Journal of the Franklin Institute 335 (5): 915–928. doi:10.1016/s0016-0032(97)00023-9.
- ↑ Deze Zeng; Xuan Zeng; G. Lu; B. Tang (2011). "Automatic modulation classification of radar signals using the generalised time-frequency representation of Zhao, Atlas and Marks". IET Radar, Sonar & Navigation 5 (4): 507–516. doi:10.1049/iet-rsn.2010.0174.
- ↑ James R. Bulgrin; Bernard J. Rubal; Theodore E. Posch; Joe M. Moody. "Comparison of binomial, ZAM and minimum cross-entropy time-frequency distributions of intracardiac heart sounds". Signals, Systems and Computers, 1994. 1994 Conference Record of the Twenty-Eighth Asilomar Conference on 1: 383–387.
- ↑ Christos, Skeberis, Zaharias D. Zaharis, Thomas D. Xenos, and Dimitrios Stratakis. (2014). "ZAM distribution analysis of radiowave ionospheric propagation interference measurements". Telecommunications and Multimedia (TEMU), 2014 International Conference on: 155–161.
- ↑ Leon Cohen (1989). "Time-frequency distributions-a review". Proceedings of the IEEE 77 (7): 941–981. doi:10.1109/5.30749.
- ↑ Y. Zhao; L. E. Atlas; R. J. Marks II (July 1990). "The use of cone-shape kernels for generalized time-frequency representations of nonstationary signals". IEEE Transactions on Acoustics, Speech, and Signal Processing 38 (7): 1084–1091. doi:10.1109/29.57537.
- ↑ R.J. Marks II (2009). Handbook of Fourier analysis & its applications. Oxford University Press.
- ↑ Patrick J. Loughlin; James W. Pitton; Les E. Atlas (1993). "Bilinear time-frequency representations: New insights and properties". IEEE Transactions on Signal Processing 41 (2): 750–767. doi:10.1109/78.193215. Bibcode: 1993ITSP...41..750L.
- ↑ Seho Oh; R. J. Marks II (1992). "Some properties of the generalized time frequency representation with cone-shaped kernel". IEEE Transactions on Signal Processing 40 (7): 1735–1745. doi:10.1109/78.143445. Bibcode: 1992ITSP...40.1735O.
- ↑ [1] Time-Frequency Toolbox For Use with MATLAB
- ↑ [2] National Instruments. LabVIEW Tools for Time-Frequency, Time-Series, and Wavelet Analysis. [3] TFA Cone-Shaped Distribution VI
 |