Desmic system
thumb|right|Two desmic tetrahedra. The third tetrahedron of this system is not shown, but has one vertex at the center and the other three on the plane at infinity.
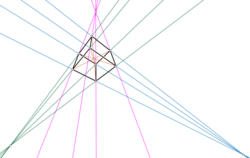
In projective geometry, a desmic system (from el δεσμός 'band, chain') is a set of three tetrahedra in 3-dimensional projective space, such that any two are desmic (related such that each edge of one cuts a pair of opposite edges of the other). It was introduced by Stephanos (1879). The three tetrahedra of a desmic system are contained in a pencil of quartic surfaces.
Every line that passes through two vertices of two tetrahedra in the system also passes through a vertex of the third tetrahedron. The 12 vertices of the desmic system and the 16 lines formed in this way are the points and lines of a Reye configuration.
Example
The three tetrahedra given by the equations
- [math]\displaystyle{ \displaystyle (w^2-x^2)(y^2-z^2) = 0 }[/math]
- [math]\displaystyle{ \displaystyle (w^2-y^2)(x^2-z^2) = 0 }[/math]
- [math]\displaystyle{ \displaystyle (w^2-z^2)(y^2-x^2) = 0 }[/math]
form a desmic system, contained in the pencil of quartics
- [math]\displaystyle{ \displaystyle a(w^2x^2+y^2z^2) + b(w^2y^2+x^2z^2) + c (w^2z^2+x^2y^2) = 0 }[/math]
for a + b + c = 0.
References
- Borwein, Peter B (1983), "The Desmic conjecture", Journal of Combinatorial Theory, Series A 35 (1): 1–9, doi:10.1016/0097-3165(83)90022-5.
- Hudson, R. W. H. T. (1990), Kummer's quartic surface, Cambridge Mathematical Library, Cambridge University Press, ISBN 978-0-521-39790-2, https://archive.org/details/184605691.
- Stephanos, Cyparissos (1879), "Sur les systèmes desmiques de trois tétraèdres", Bulletin des sciences mathématiques et astronomiques, Série 2 3 (1): 424–456, http://www.numdam.org/item?id=BSMA_1879_2_3_1_424_1.
External links
 |

