Isbell duality
Isbell conjugacy or Isbell duality (named after John R. Isbell[1][2]) is a fundamental construction of enriched category theory formally introduced by William Lawvere in 1986.[3][4] That is a duality between covariant and contravariant representable presheaves associated with an objects of categories under the Yoneda embedding.[5]
Definition
The Yoneda embedding
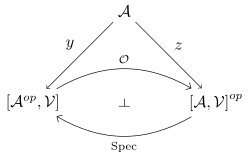
[math]\displaystyle{ Y:\mathcal{A} \rightarrow \left[\mathcal{A}^{op}, \mathcal{V} \right] }[/math]
[math]\displaystyle{ X \mapsto \mathrm{hom} (-,X). }[/math]
and the co-Yoneda embedding[1][6] or the dual Yoneda embedding[7]
[math]\displaystyle{ Z:\mathcal{A} \rightarrow \left[\mathcal{A}, \mathcal{V} \right]^{op} }[/math]
[math]\displaystyle{ X \mapsto \mathrm{hom} (X,-). }[/math]
Let [math]\displaystyle{ \mathcal{V} }[/math] be a symmetric monoidal closed category, and let [math]\displaystyle{ \mathcal{A} }[/math] be a small category enriched in [math]\displaystyle{ \mathcal{V} }[/math].
The Isbell conjugacy is an adjunction between the categories; [math]\displaystyle{ \left(\mathcal{O} \dashv \mathrm{Spec} \right) \colon \left[\mathcal{A}^{op}, \mathcal{V} \right] {\underset{\mathrm{Spec}}{\overset{\mathcal{O}}{\rightleftarrows}}} \left[\mathcal{A}, \mathcal{V} \right]^{op} }[/math].[3][1][8][9][6]
The functors [math]\displaystyle{ \mathcal{O} \dashv \mathrm{Spec} }[/math] of Isbell duality are such that [math]\displaystyle{ \mathcal{O} \cong \mathrm{Lan_{Y}Z} }[/math] and [math]\displaystyle{ \mathrm{Spec} \cong \mathrm{Lan_{Z}Y} }[/math].[8][10][note 1]
See also
References
Bibliography
- Avery, Tom; Leinster, Tom (2021), "Isbell conjugacy and the reflexive completion", Theory and Applications of Categories 36: 306–347
- Baez, John C. (2022), "Isbell Duality", Notices Amer. Math. Soc. 70: 140–141, https://www.ams.org/journals/notices/202301/noti2602/noti2602.html?adat=January%202023&trk=2602&galt=none&cat=column&pdfissue=202301&pdffile=rnoti-p140.pdf
- Day, Brian J.; Lack, Stephen (2007), "Limits of small functors", Journal of Pure and Applied Algebra 210 (3): 651–663, doi:10.1016/j.jpaa.2006.10.019.
- Di Liberti, Ivan (2020), "Codensity: Isbell duality, pro-objects, compactness and accessibility", Journal of Pure and Applied Algebra 224 (10), doi:10.1016/j.jpaa.2020.106379
- Fosco, Loregian (22 July 2021), (Co)end Calculus, Cambridge University Press, doi:10.1017/9781108778657, ISBN 9781108746120, https://books.google.com/books?id=cfIuEAAAQBAJ&dq&pg=PA90
- Gutierres, Gonçalo; Hofmann, Dirk (2013), "Approaching Metric Domains", Applied Categorical Structures 21 (6): 617–650, doi:10.1007/s10485-011-9274-z
- Shen, Lili; Zhang, Dexue (2013), "Categories enriched over a quantaloid: Isbell adjunctions and Kan adjunctions", Theory and Applications of Categories 28 (20): 577-615, http://www.tac.mta.ca/tac/volumes/28/20/28-20.pdf
- Isbell, J. R. (1960), "Adequate subcategories", Illinois Journal of Mathematics 4 (4), doi:10.1215/ijm/1255456274
- Isbell, John R. (1966), "Structure of categories", Bulletin of the American Mathematical Society 72 (4): 619–656, doi:10.1090/S0002-9904-1966-11541-0
- {{citation
| last = Kelly | first = Gregory Maxwell | isbn = 0-521-28702-2 | mr = 651714 | publisher = Cambridge University Press, Cambridge-New York | series = London Mathematical Society Lecture Note Series | title = Basic concepts of enriched category theory | volume = 64
- "Taking categories seriously (p. 169)", Revista Colombiana de Matemáticas 20 (3–4): 147–178, 1986, http://eudml.org/doc/181771
- Melliès, Paul-André; Zeilberger, Noam (2018), "An Isbell duality theorem for type refinement systems", Mathematical Structures in Computer Science 28 (6): 736–774, doi:10.1017/S0960129517000068
- Rutten, J.J.M.M. (1998), "Weighted colimits and formal balls in generalized metric spaces", Topology and Its Applications 89 (1–2): 179–202, doi:10.1016/S0166-8641(97)00224-1
- Sturtz, Kirk (2018), "The factorization of the Giry monad", Advances in Mathematics 340: 76–105, doi:10.1016/j.aim.2018.10.007
- Wood, R.J (1982), "Some remarks on total categories", Journal of Algebra 75 (2): 538–545, doi:10.1016/0021-8693(82)90055-2
Footnote
- ↑ For the symbol Lan, see left Kan extension.
External links
- Loregian, Fosco (2018), Kan extensions, https://tetrapharmakon.github.io/stuff/REFCARDS_kan.pdf
- Di Liberti, Ivan; Loregian, Fosco (2019). "On the unicity of formal category theories". arXiv:1901.01594 [math.CT].
- Isbell duality, https://ncatlab.org/nlab/show/Isbell+duality
- Yoneda embedding, https://ncatlab.org/nlab/show/Yoneda+embedding
- co-Yoneda lemma, https://ncatlab.org/nlab/show/co-Yoneda+lemma
 |