Lawvere–Tierney topology

In mathematics, a Lawvere–Tierney topology is an analog of a Grothendieck topology for an arbitrary topos, used to construct a topos of sheaves. A Lawvere–Tierney topology is also sometimes also called a local operator or coverage or topology or geometric modality. They were introduced by William Lawvere (1971) and Myles Tierney.

Definition

If E is a topos, then a topology on E is a morphism j from the subobject classifier Ω to Ω such that j preserves truth ([math]\displaystyle{ j \circ \mbox{true} = \mbox{true} }[/math]), preserves intersections ([math]\displaystyle{ j \circ \wedge = \wedge \circ (j \times j) }[/math]), and is idempotent ([math]\displaystyle{ j \circ j = j }[/math]).

j-closure

Commutative diagrams showing how j-closure operates. Ω and t are the subobject classifier. χ_s is the characteristic morphism of s as a subobject of A and [math]\displaystyle{ j \circ \chi_s }[/math] is the characteristic morphism of [math]\displaystyle{ \bar s }[/math] which is the j-closure of s. The bottom two squares are pullback squares and they are contained in the top diagram as well: the first one as a trapezoid and the second one as a two-square rectangle.

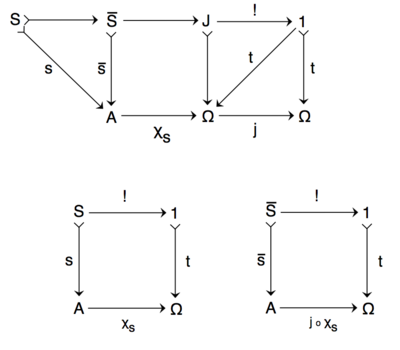
Given a subobject [math]\displaystyle{ s:S \rightarrowtail A }[/math] of an object A with classifier [math]\displaystyle{ \chi_s:A \rightarrow \Omega }[/math], then the composition [math]\displaystyle{ j \circ \chi_s }[/math] defines another subobject [math]\displaystyle{ \bar s:\bar S \rightarrowtail A }[/math] of A such that s is a subobject of [math]\displaystyle{ \bar s }[/math], and [math]\displaystyle{ \bar s }[/math] is said to be the j-closure of s.

Some theorems related to j-closure are (for some subobjects s and w of A):

• inflationary property: [math]\displaystyle{ s \subseteq \bar s }[/math]
• idempotence: [math]\displaystyle{ \bar s \equiv \bar \bar s }[/math]
• preservation of intersections: [math]\displaystyle{ \overline{s \cap w} \equiv \bar s \cap \bar w }[/math]
• preservation of order: [math]\displaystyle{ s \subseteq w \Longrightarrow \bar s \subseteq \bar w }[/math]
• stability under pullback: [math]\displaystyle{ \overline{f^{-1}(s)} \equiv f^{-1}(\bar s) }[/math].

Examples

Grothendieck topologies on a small category C are essentially the same as Lawvere–Tierney topologies on the topos of presheaves of sets over C.

References

• "Quantifiers and sheaves", Actes du Congrès International des Mathématiciens (Nice, 1970), 1, Paris: Gauthier-Villars, 1971, pp. 329–334, https://pdfs.semanticscholar.org/6630/846a00261a071b71e264e0f532e29cd9152f.pdf 
• Mac Lane, Saunders; Moerdijk, Ieke (2012), Sheaves in geometry and logic. A first introduction to topos theory, Universitext, Springer, ISBN 978-1-4612-0927-0, https://books.google.com/books?id=LZWLBAAAQBAJ&pg=PP8 
• McLarty, Colin (1995), Elementary Categories, Elementary Toposes, Oxford Logic Guides, 21, Oxford University Press, p. 196, ISBN 978-0-19-158949-2, https://books.google.com/books?id=V8cON1x39bIC&pg=PR9