Moss's Egg
From HandWiki
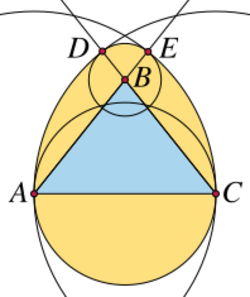
In Euclidean geometry, Moss's Egg is an oval made by smoothly connecting four circular arcs. It can be constructed from any isosceles triangle ABC with apex B, as long as the angle at B is greater than 60°; often, ABC is an isosceles right triangle.
To construct Moss's egg:[1][2][3]
- Draw a semicircle on the base AC of the triangle, outside of the triangle.
- Connect it to a circular arc centered at C from A to a point D on line BC, and by another circular arc centered at A from C to a point E on line AB. When the apex angle at B is greater than 60°, these two points D and E will be outside the triangle, equidistant from B.
- Complete the oval by a circular arc centered at B, from D to E.
References
- ↑ Dixon, R (1991). Mathographics. Dover. p. 5. https://archive.org/details/mathographics0000dixo.
- ↑ Weisstein, Eric. "Math World". Wolfram Math World. http://mathworld.wolfram.com/MosssEgg.html. Retrieved 19 August 2015.
- ↑ Hreinsdóttir, Freyja. "Euclidean Eggs". https://www.dynamat.oriw.eu/upload_pdf/20121022_154322__0.pdf. Retrieved 18 June 2020.
External links
- Video: How to draw an egg, The Aperiodical