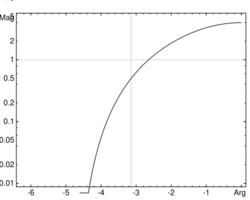
Nichols plot
The Nichols plot is a plot used in signal processing and control design, named after American engineer Nathaniel B. Nichols.[1][2][3]
Use in control design
Given a transfer function,
- [math]\displaystyle{ G(s) = \frac{Y(s)}{X(s)} }[/math]
with the closed-loop transfer function defined as,
- [math]\displaystyle{ M(s) = \frac{G(s)}{1+G(s)} }[/math]
the Nichols plots displays [math]\displaystyle{ 20 \log_{10}(|G(s)|) }[/math] versus [math]\displaystyle{ \arg(G(s)) }[/math]. Loci of constant [math]\displaystyle{ 20 \log_{10}(|M(s)|) }[/math] and [math]\displaystyle{ \arg(M(s)) }[/math] are overlaid to allow the designer to obtain the closed loop transfer function directly from the open loop transfer function. Thus, the frequency [math]\displaystyle{ \omega }[/math] is the parameter along the curve. This plot may be compared to the Bode plot in which the two inter-related graphs - [math]\displaystyle{ 20 \log_{10}(|G(s)|) }[/math] versus [math]\displaystyle{ \log_{10}(\omega) }[/math] and [math]\displaystyle{ \arg(G(s)) }[/math] versus [math]\displaystyle{ \log_{10}(\omega) }[/math]) - are plotted.
In feedback control design, the plot is useful for assessing the stability and robustness of a linear system. This application of the Nichols plot is central to the quantitative feedback theory (QFT) of Horowitz and Sidi, which is a well known method for robust control system design.
In most cases, [math]\displaystyle{ \arg(G(s)) }[/math] refers to the phase of the system's response. Although similar to a Nyquist plot, a Nichols plot is plotted in a Cartesian coordinate system while a Nyquist plot is plotted in a Polar coordinate system.
See also
References
- ↑ Isaac M. Howowitz, Synthesis of Feedback Systems, Academic Press, 1963, Lib Congress 63-12033 p. 194-198
- ↑ Boris J. Lurie and Paul J. Enright, Classical Feedback Control, Marcel Dekker, 2000, ISBN:0-8247-0370-7 p. 10
- ↑ Allen Stubberud, Ivan Williams, and Joseph DeStefano, Shaums Outline Feedback and Control Systems, McGraw-Hill, 1995, ISBN:0-07-017052-5 ch. 17
External links
 |