Physics:Breit-wigner distribution
Probability density functions of the general form
are also known in statistics as Cauchy distributions. The Breit-Wigner (also known as the Lorentz) distribution is a generalized form originally introduced Breit36 to describe the cross-section of resonant nuclear scattering in the form
which had been derived from the transition probability of a resonant state with known lifetime (Bohr69, Breit59, Fermi51, Paul69). In this form, the integral over all energies is 1. The variance and higher moments of the Breit-Wigner distribution are infinite. The distribution is fully defined by E0, the position of its maximum (about which the distribution is symmetric), and by ![]() , the full width at half maximum (FWHM), as obviously
, the full width at half maximum (FWHM), as obviously
In the above form, the Breit-Wigner distribution has also been widely used for describing the non-interfering production cross-section of particle resonant states, the parameters E0 (= mass of resonance) and ![]() (= width of resonance) being determined from the observed data. Observed Breit-Wigner distributions usually have a width larger than
(= width of resonance) being determined from the observed data. Observed Breit-Wigner distributions usually have a width larger than ![]() , being a convolution with a resolution function due to measurement uncertainties.
, being a convolution with a resolution function due to measurement uncertainties.
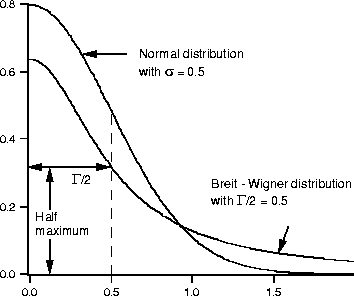
The Gaussian curve decreases much faster than the Breit-Wigner curve in the tails. For a Gaussian, FWHM = 2.355 ![]()
![]() here being the standard deviation. In the diagram below, both curves are normalized to the same integral; a normal distribution with the same FWHM as the Breit-Wigner distribution would be even narrower.
here being the standard deviation. In the diagram below, both curves are normalized to the same integral; a normal distribution with the same FWHM as the Breit-Wigner distribution would be even narrower.