Physics:Moving load
In structural dynamics, a moving load changes the point at which the load is applied over time.[citation needed] Examples include a vehicle that travels across a bridge[citation needed] and a train moving along a track.[citation needed]
Properties
In computational models, load is usually applied as
- a simple massless force,[citation needed]
- an oscillator, or
- an inertial force (mass and a massless force).[citation needed]
Numerous historical reviews of the moving load problem exist.[1][2] Several publications deal with similar problems.[3]
The fundamental monograph is devoted to massless loads.[4] Inertial load in numerical models is described in [5]
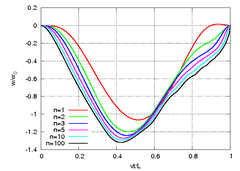
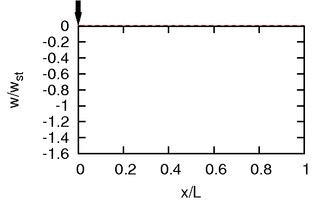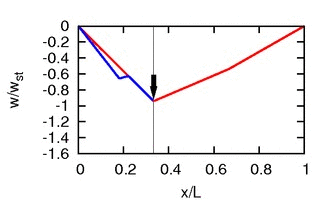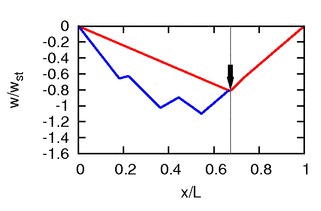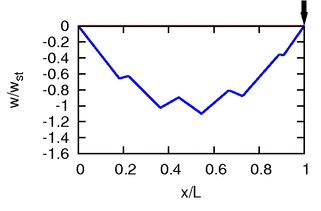
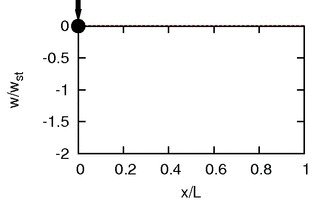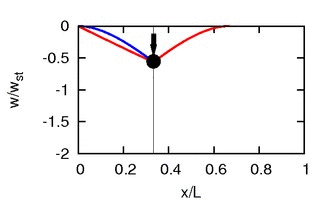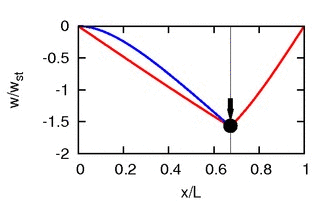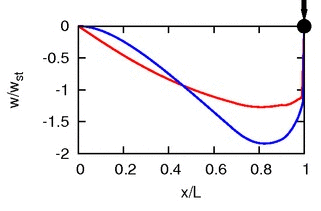
Unexpected property of differential equations that govern the motion of the mass particle travelling on the string, Timoshenko beam, and Mindlin plate is described in.[6] It is the discontinuity of the mass trajectory near the end of the span (well visible in string at the speed v=0.5c).[citation needed] The moving load significantly increases displacements.[citation needed] The critical velocity, at which the growth of displacements is the maximum, must be taken into account in engineering projects.[citation needed]
Structures that carry moving loads can have finite dimensions or can be infinite and supported periodically or placed on the elastic foundation.[citation needed]
Consider simply supported string of the length l, cross-sectional area A, mass density ρ, tensile force N, subjected to a constant force P moving with constant velocity v. The motion equation of the string under the moving force has a form[citation needed]
- [math]\displaystyle{ -N\frac{\partial^2w(x,t)}{\partial x^2}+\rho A\frac{\partial^2w(x,t)}{\partial t^2}=\delta(x-vt)P\ . }[/math]
Displacements of any point of the simply supported string is given by the sinus series[citation needed]
- [math]\displaystyle{ w(x,t) = \frac{2P}{\rho Al}\sum_{j=1}^{\infty}\frac{1}{\omega_{(j)}^2-\omega^2}\left(\sin(\omega t)-\frac{\omega}{\omega_{(j)}}\sin(\omega_{(j)}t)\right)\sin\frac{j\pi x}{l}\ , }[/math]
where
- [math]\displaystyle{ \omega=\frac{j\pi v}{l}\ , }[/math]
and the natural circular frequency of the string
- [math]\displaystyle{ \omega_{(j)}^2=\frac{j^2\pi^2}{l^2}\frac{N}{\rho A}\ . }[/math]
In the case of inertial moving load, the analytical solutions are unknown.[citation needed] The equation of motion is increased by the term related to the inertia of the moving load. A concentrated mass m accompanied by a point force P:[citation needed]
- [math]\displaystyle{ -N\frac{\partial^2w(x,t)}{\partial x^2}+\rho A\frac{\partial^2w(x,t)}{\partial t^2}=\delta(x-vt)P-\delta(x-vt)m\frac{\mbox{d}^2w(vt,t)}{\mbox{d}t^2}\ . }[/math]
The last term, because of complexity of computations, is often neglected by engineers.[citation needed] The load influence is reduced to the massless load term.[citation needed] Sometimes the oscillator is placed in the contact point.[citation needed] Such approaches are acceptable only in low range of the travelling load velocity.[citation needed] In higher ranges both the amplitude and the frequency of vibrations differ significantly in the case of both types of a load.[citation needed]
The differential equation can be solved in a semi-analytical way only for simple problems.[citation needed] The series determining the solution converges well and 2-3 terms are sufficient in practice.[citation needed] More complex problems can be solved by the finite element method[citation needed] or space-time finite element method.[citation needed]
| massless load | inertial load |
|---|---|
The discontinuity of the mass trajectory is also well visible in the Timoshenko beam.[citation needed] High shear stiffness emphasizes the phenomenon.[citation needed]
The Renaudot approach vs. the Yakushev approach
Renaudot approach
- [math]\displaystyle{ \delta(x-vt)\frac{\mbox{d}}{\mbox{d}t}\left[m\frac{\mbox{d}w(vt,t)}{\mbox{d}t}\right]=\delta(x-vt)m\frac{\mbox{d}^2w(vt,t)}{\mbox{d}t^2}\ . ===Yakushev approach=== : \lt math\gt \frac{\mbox{d}}{\mbox{d}t}\left[\delta(x-vt)m\frac{\mbox{d}w(vt,t)}{\mbox{d}t}\right]=-\delta^\prime(x-vt)mv\frac{\mbox{d}w(vt,t)}{\mbox{d}t}+\delta(x-vt)m\frac{\mbox{d}^2w(vt,t)}{\mbox{d}t^2}\ . ==Massless string under moving inertial load== Consider a massless string, which is a particular case of moving inertial load problem. The first to solve the problem was Smith.\lt ref name="smith64"\gt {{cite news|author=C.E. Smith|title=Motion of a stretched string carrying a moving mass particle|journal=J. Appl. Mech.|year=1964|volume=31|number=1|pages=29–37}}\lt /ref\gt The analysis will follow the solution of Fryba.\lt ref name="fryba" /\gt Assuming {{math|ρ}}=0, the equation of motion of a string under a moving mass can be put into the following form{{Citation needed|date=June 2021}} : \lt math\gt -N\frac{\partial^2w(x,t)}{\partial x^2}=\delta(x-vt)P-\delta(x-vt)\,m\frac{\mbox{d}^2w(vt,t)}{\mbox{d}t^2}\ . }[/math]
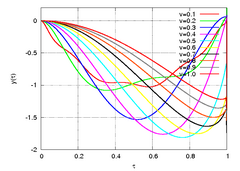
We impose simply-supported boundary conditions and zero initial conditions.[citation needed] To solve this equation we use the convolution property.[citation needed] We assume dimensionless displacements of the string y and
- [math]\displaystyle{ y(\tau)=\frac{w(vt,t)}{w_{st}}\ ,\ \ \ \ \tau\ =\ \frac{vt}{l}\ , }[/math]
where wst is the static deflection in the middle of the string. The solution is given by a sum
- [math]\displaystyle{ y(\tau)=\frac{4\,\alpha}{\alpha\,-\,1}\,\tau\,(\tau-1)\,\sum_{k=1}^\infty\,\prod_{i=1}^k\frac{(a+i-1)(b+i-1)}{c+i-1}\;\frac{\tau^k}{k!}\ , }[/math]
where α is the dimensionless parameters :
- [math]\displaystyle{ \alpha=\frac{Nl}{2mv2}\,\gt \,0\ \ \ \wedge\ \ \ \alpha\,\neq\,1\ . }[/math]
Parameters a, b and c are given below
- [math]\displaystyle{ a_{1,2}=\frac{3\,\pm\,\sqrt{1+8\alpha}}{2}\ ,\ \ \ \ \ b_{1,2}=\frac{3\,\mp\,\sqrt{1+8\alpha}}{2}\ ,\ \ \ \ \ c=2\ . }[/math]
In the case of α=1, the considered problem has a closed solution: [math]\displaystyle{ y(\tau )=\left[\frac{4}{3}\tau (1-\tau) -\frac{4}{3}\tau \left( 1+2 \tau\ln (1-\tau )+2\ln (1-\tau )\right)\right]\ . }[/math]
References
- ↑ Inglis, C.E. (1934). A Mathematical Treatise on Vibrations in Railway Bridges. Cambridge University Press.
- ↑ Schallenkamp, A. (1937). "Schwingungen von Tragern bei bewegten Lasten" (in German). Ingenieur-Archiv (Stringer Nature) 8 (3): 182–98. doi:10.1007/BF02085995.
- ↑ A.V. Pesterev; L.A. Bergman; C.A. Tan; T.C. Tsao; B. Yang (2003). "On Asymptotics of the Solution of the Moving Oscillator Problem". J. Sound Vib. 260: pp. 519–36. http://www.eng.wayne.edu/user_files/258/09_EquivalenceJSV_JournalArticle.pdf.
- ↑ Fryba, L. (1999). Vibrations of Solids and Structures Under Moving Loads. Thomas Telford House. ISBN 9780727727411. https://books.google.com/books?id=3RP4T4Oc0LUC.
- ↑ Bajer, C.I.; Dyniewicz, B. (2012). Numerical Analysis of Vibrations of Structures Under Moving Inertial Load. Lecture Notes in Applied and Computational Mechanics. 65. Springer. doi:10.1007/978-3-642-29548-5. ISBN 978-3-642-29547-8.
- ↑ B. Dyniewicz; C.I. Bajer (2009). "Paradox of the Particle's Trajectory Moving on a String". Arch. Appl. Mech. 79 (3): 213–23. doi:10.1007/s00419-008-0222-9. Bibcode: 2009AAM....79..213D.
 |