Physics:Pendulum wave
Lua error in Module:Multiple_image at line 163: attempt to perform arithmetic on local 'totalwidth' (a nil value). A pendulum wave is an elementary physics demonstration and kinetic art comprising a number of uncoupled simple pendulums with monotonically increasing lengths. As the pendulums oscillate, they appear to produce travelling and standing waves, beating, and random motion.[1][2][3]
History
Ernst Mach designed and constructed the first pendulum wave demonstration around 1867 at Charles-Ferdinand University in Prague. In the Czech Republic, the demonstration is called Machuv vlnostroj (the wave machine of Mach). Prof Eric J. Heller at Harvard University suggested the use of the demonstration to simulate quantum revival.[1]
In 2001, two University of Minnesota Morris researchers have derived a continuous function explaining the patterns in the pendulums using an extension to the equation for traveling waves in one dimension, and showed that their cycling arises from aliasing of the underlying continuous function.[4]
In 2020, illusionist Kevin McMahon, incorporated a massive pendulum wave apparatus, supposedly with flaming cannonballs, as a stunt in Britain's Got Talent (series 14) under the stage name Kevin Quantum.[5]
Design
|
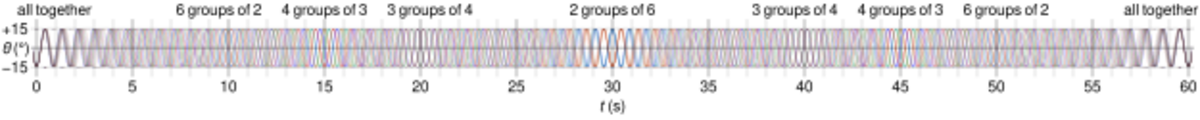
The lengths of the pendulums are set such that in a given time t, the first pendulum completes n oscillations, and each subsequent one completes one more oscillation than the previous. As all pendulums are started together, their relative phases change continuously, but after time t, they come back in sync and the sequence repeats.[1] For small perturbations, the period of a pendulum is given by
where L is the length of the pendulum and g is the standard acceleration due to gravity.[6] As t/n is the period of a pendulum completing n oscillations in t,
A common choice of t is 60 seconds. Thus, for g ≈ 9.8 ms−2,
|
Parameters of the |
See also
- Newton's cradle – a set of pendulums constrained to swing along the axis of the apparatus and collide with one another
References
- ↑ Jump up to: 1.0 1.1 1.2 Harvard Natural Sciences Lecture Demonstrations, Pendulum Waves
- ↑ K P Zetie, The pendulum wave machine, Physics Education, Volume 50, Number 3, 23 April 2015
- ↑ Fyzmatik.pise, Machův vlnostroj, 16. červen 2012
- ↑ James A. Flaten and Kevin A. Parendo, Pendulum waves: A lesson in aliasing, American Journal of Physics 69, 778 (2001)
- ↑ BBC News, The Faking It magician who now teaches magic, 18 December 2020
- ↑ Halliday, David; Robert Resnick; Jearl Walker (1997). Fundamentals of Physics, 5th Ed. New York: John Wiley & Sons.. p. 381. ISBN 978-0-471-14854-8. https://archive.org/details/fundamentalsofp000davi/page/381.
 |