Physics:Willam-Warnke yield criterion
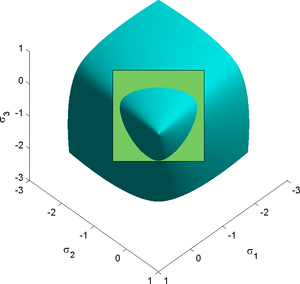
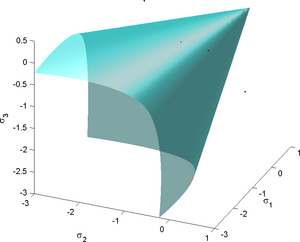
The Willam-Warnke yield criterion [1] is a function that is used to predict when failure will occur in concrete and other cohesive-frictional materials such as rock, soil, and ceramics. This yield criterion has the functional form
- [math]\displaystyle{ f(I_1, J_2, J_3) = 0 \, }[/math]
where [math]\displaystyle{ I_1 }[/math] is the first invariant of the Cauchy stress tensor, and [math]\displaystyle{ J_2, J_3 }[/math] are the second and third invariants of the deviatoric part of the Cauchy stress tensor. There are three material parameters ([math]\displaystyle{ \sigma_c }[/math] - the uniaxial compressive strength, [math]\displaystyle{ \sigma_t }[/math] - the uniaxial tensile strength, [math]\displaystyle{ \sigma_b }[/math] - the equibiaxial compressive strength) that have to be determined before the Willam-Warnke yield criterion may be applied to predict failure.
In terms of [math]\displaystyle{ I_1, J_2, J_3 }[/math], the Willam-Warnke yield criterion can be expressed as
- [math]\displaystyle{ f := \sqrt{J_2} + \lambda(J_2,J_3)~(\tfrac{I_1}{3} - B) = 0 }[/math]
where [math]\displaystyle{ \lambda }[/math] is a function that depends on [math]\displaystyle{ J_2,J_3 }[/math] and the three material parameters and [math]\displaystyle{ B }[/math] depends only on the material parameters. The function [math]\displaystyle{ \lambda }[/math] can be interpreted as the friction angle which depends on the Lode angle ([math]\displaystyle{ \theta }[/math]). The quantity [math]\displaystyle{ B }[/math] is interpreted as a cohesion pressure. The Willam-Warnke yield criterion may therefore be viewed as a combination of the Mohr-Coulomb and the Drucker-Prager yield criteria.
Willam-Warnke yield function
In the original paper, the three-parameter Willam-Warnke yield function was expressed as
- [math]\displaystyle{ f = \cfrac{1}{3z}~\cfrac{I_1}{\sigma_c} + \sqrt{\cfrac{2}{5}}~\cfrac{1}{r(\theta)}\cfrac{\sqrt{J_2}}{\sigma_c} - 1 \le 0 }[/math]
where [math]\displaystyle{ I_1 }[/math] is the first invariant of the stress tensor, [math]\displaystyle{ J_2 }[/math] is the second invariant of the deviatoric part of the stress tensor, [math]\displaystyle{ \sigma_c }[/math] is the yield stress in uniaxial compression, and [math]\displaystyle{ \theta }[/math] is the Lode angle given by
- [math]\displaystyle{ \theta = \tfrac{1}{3}\cos^{-1}\left(\cfrac{3\sqrt{3}}{2}~\cfrac{J_3}{J_2^{3/2}}\right) ~. }[/math]
The locus of the boundary of the stress surface in the deviatoric stress plane is expressed in polar coordinates by the quantity [math]\displaystyle{ r(\theta) }[/math] which is given by
- [math]\displaystyle{ r(\theta) := \cfrac{u(\theta)+v(\theta)}{w(\theta)} }[/math]
where
- [math]\displaystyle{ \begin{align} u(\theta) := & 2~r_c~(r_c^2-r_t^2)~\cos\theta \\ v(\theta) := & r_c~(2~r_t - r_c)\sqrt{4~(r_c^2 - r_t^2)~\cos^2\theta + 5~r_t^2 - 4~r_t~r_c} \\ w(\theta) := & 4(r_c^2 - r_t^2)\cos^2\theta + (r_c-2~r_t)^2 \end{align} }[/math]
The quantities [math]\displaystyle{ r_t }[/math] and [math]\displaystyle{ r_c }[/math] describe the position vectors at the locations [math]\displaystyle{ \theta=0^\circ, 60^\circ }[/math] and can be expressed in terms of [math]\displaystyle{ \sigma_c, \sigma_b, \sigma_t }[/math] as (here [math]\displaystyle{ \sigma_b }[/math] is the failure stress under equi-biaxial compression and [math]\displaystyle{ \sigma_t }[/math] is the failure stress under uniaxial tension)
- [math]\displaystyle{ r_c := \sqrt{\cfrac{6}{5}}\left[\cfrac{\sigma_b\sigma_t}{3\sigma_b\sigma_t + \sigma_c(\sigma_b - \sigma_t)}\right] ~;~~ r_t := \sqrt{\cfrac{6}{5}}\left[\cfrac{\sigma_b\sigma_t}{\sigma_c(2\sigma_b+\sigma_t)}\right] }[/math]
The parameter [math]\displaystyle{ z }[/math] in the model is given by
- [math]\displaystyle{ z := \cfrac{\sigma_b\sigma_t}{\sigma_c(\sigma_b-\sigma_t)} ~. }[/math]
The Haigh-Westergaard representation of the Willam-Warnke yield condition can be written as
- [math]\displaystyle{ f(\xi, \rho, \theta) = 0 \, \quad \equiv \quad f := \bar{\lambda}(\theta)~\rho + \bar{B}~\xi - \sigma_c \le 0 }[/math]
where
- [math]\displaystyle{ \bar{B} := \cfrac{1}{\sqrt{3}~z} ~;~~ \bar{\lambda} := \cfrac{1}{\sqrt{5}~r(\theta)} ~. }[/math]
Modified forms of the Willam-Warnke yield criterion
An alternative form of the Willam-Warnke yield criterion in Haigh-Westergaard coordinates is the Ulm-Coussy-Bazant form:[2]
- [math]\displaystyle{ f(\xi, \rho, \theta) = 0 \, \quad \text{or} \quad f := \rho + \bar{\lambda}(\theta)~\left(\xi - \bar{B}\right) = 0 }[/math]
where
- [math]\displaystyle{ \bar{\lambda} := \sqrt{\tfrac{2}{3}}~\cfrac{u(\theta)+v(\theta)}{w(\theta)} ~;~~ \bar{B} := \tfrac{1}{\sqrt{3}}~\left[\cfrac{\sigma_b\sigma_t}{\sigma_b-\sigma_t}\right] }[/math]
and
- [math]\displaystyle{ \begin{align} r_t := & \cfrac{\sqrt{3}~(\sigma_b-\sigma_t)}{2\sigma_b-\sigma_t} \\ r_c := & \cfrac{\sqrt{3}~\sigma_c~(\sigma_b-\sigma_t)}{(\sigma_c+\sigma_t)\sigma_b-\sigma_c\sigma_t} \end{align} }[/math]
The quantities [math]\displaystyle{ r_c, r_t }[/math] are interpreted as friction coefficients. For the yield surface to be convex, the Willam-Warnke yield criterion requires that [math]\displaystyle{ 2~r_t \ge r_c \ge r_t/2 }[/math] and [math]\displaystyle{ 0 \le \theta \le \cfrac{\pi}{3} }[/math].
See also
References
- ↑ Willam, K. J. and Warnke, E. P. (1975). Constitutive models for the triaxial behavior of concrete. Proceedings of the International Assoc. for Bridge and Structural Engineering , vol 19, pp. 1- 30.
- ↑ Ulm, F-J., Coussy, O., Bazant, Z. (1999) The ‘‘Chunnel’’ Fire. I: Chemoplastic softening in rapidly heated concrete. ASCE Journal of Engineering Mechanics, vol. 125, no. 3, pp. 272-282.
- Chen, W. F. (1982). Plasticity in Reinforced Concrete. McGraw Hill. New York.
External links
- Kaspar Willam and E.P. Warnke (1974). Constitutive model for the triaxial behavior of concrete
- Palko, J. L. (1993). Interactive reliability model for whisker-toughened ceramics
- The ‘‘Chunnel’’ Fire. I: Chemoplastic softening in rapidly heated concrete by Franz-Josef Ulm, Olivier Coussy, and Zdeneˇk P. Bazˇant.