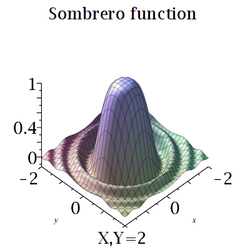
Sombrero function
A sombrero function (sometimes called besinc function or jinc function[1]) is the 2-dimensional polar coordinate analog of the sinc function, and is so-called because it is shaped like a sombrero hat. This function is frequently used in image processing.[2] It can be defined through the Bessel function of the first kind ([math]\displaystyle{ J_1 }[/math]) where ρ2 = x2 + y2. [math]\displaystyle{ \operatorname{somb} (\rho) = \frac{2 J_1(\pi \rho)}{\pi \rho}. }[/math]
The normalization factor 2 makes somb(0) = 1. Sometimes the π factor is omitted, giving the following alternative definition: [math]\displaystyle{ \operatorname{somb} (\rho) = \frac{2 J_1(\rho)}{\rho}. }[/math]
The factor of 2 is also often omitted, giving yet another definition and causing the function maximum to be 0.5:[3] [math]\displaystyle{ \operatorname{somb} (\rho) = \frac{ J_1(\rho)}{\rho}. }[/math]
The Fourier transform of the 2D circle function ([math]\displaystyle{ circ(\rho) }[/math]) is a sombrero function. Thus a sombrero function also appears in the intensity profile of far-field diffraction through a circular aperture, known as an Airy disk.
References
- ↑ Richard E. Blahut (2004-11-18). Theory of Remote Image Formation. Cambridge University Press. p. 82. ISBN 9781139455305. https://books.google.com/books?id=d8FMlHewYp0C&pg=PA82&dq=%22jinc+function%22.
- ↑ William R. Hendee, Peter Neil Temple Wells (1997-06-27). The perception of visual information. p. 204. ISBN 978-0-387-94910-9. https://books.google.com/books?id=T_KNSWU4uz4C&pg=PA204.
- ↑ Weisstein, Eric W.. "Jinc Function". http://mathworld.wolfram.com/JincFunction.html.
 |