Swastika curve
From HandWiki
Short description: Mathematical quartic curve
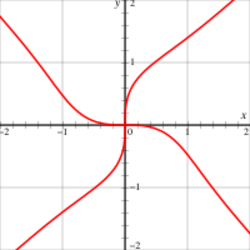
The swastika curve is the name given by Martyn Cundy and A. P. Rollett in their book Mathematical Models[1] to a type of quartic plane curve.
Equations
The plane curve with the Cartesian equation
- [math]\displaystyle{ y^4-x^4 = xy,\, }[/math]
or, equivalently, the polar equation
- [math]\displaystyle{ r^2 = \frac{\sin(\theta)\cos(\theta)}{\sin^4(\theta) - \cos^4(\theta)} = - \frac{\tan(2\theta)}{2}. \, }[/math]
The curve looks similar to the right-handed swastika. It can be inverted with respect to a unit circle to resemble a left-handed swastika. The Cartesian equation then becomes a quartic curve,
- [math]\displaystyle{ x^4 - y^4 = xy. \, }[/math]
References
- ↑ H. Martyn Cundy; A.P. Rollett (1961). Mathematical Models (second ed.). Oxford University Press. p. 71.
External links