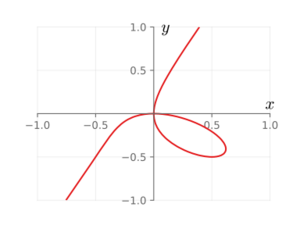
Trident curve
From HandWiki
In mathematics, a trident curve (also trident of Newton or parabola of Descartes) is any member of the family of curves that have the formula:
- [math]\displaystyle{ xy+ax^3+bx^2+cx=d }[/math]
Trident curves are cubic plane curves with an ordinary double point in the real projective plane at x = 0, y = 1, z = 0; if we substitute x = x/z and y = 1/z into the equation of the trident curve, we get
- [math]\displaystyle{ ax^3+bx^2z+cxz^2+xz = dz^3, }[/math]
which has an ordinary double point at the origin. Trident curves are therefore rational plane algebraic curves of genus zero.
References
- Lawrence, J. Dennis (1972). A Catalog of Special Plane Curves. Dover Publications. p. 110. ISBN 0-486-60288-5. https://archive.org/details/catalogofspecial00lawr/page/110.
External links
- O'Connor, John J.; Robertson, Edmund F., "Trident of Newton", MacTutor History of Mathematics archive, University of St Andrews, http://www-history.mcs.st-andrews.ac.uk/Curves/Trident.html.
 |