Uncomputation
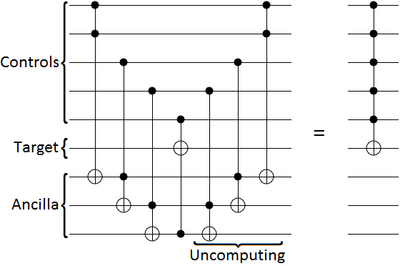
Uncomputation is a technique, used in reversible circuits, for cleaning up temporary effects on ancilla bits so that they can be re-used.[1]
Uncomputation is a fundamental step in quantum computing algorithms. Whether or not intermediate effects have been uncomputed affects how states interfere with each other when measuring results.[2]
The process is primarily motivated by the principle of implicit measurement.[3], which states that discarding a register during computation is physically equivalent to measuring it. Failure to uncompute garbage registers can have unintentional consequences. For example, if we take the state [math]\displaystyle{ }[/math] [math]\displaystyle{ \frac{1}{\sqrt 2}(|0\rangle|g_0\rangle + |1\rangle|g_1\rangle) }[/math] where [math]\displaystyle{ g_0 }[/math] and [math]\displaystyle{ g_1 }[/math] are garbage registers. Then, if we do not apply any further operations to those registers, according to the principle of implicit measurement, the entangled state has been measured, resulting in a collapse to either [math]\displaystyle{ |0\rangle|g_0\rangle }[/math] or [math]\displaystyle{ |1\rangle|g_1\rangle }[/math] with probability [math]\displaystyle{ \frac{1}{2} }[/math]. What makes this undesirable is that wave-function collapse occurs before the program terminates, and thus may not yield the expected result.
References
- ↑ Aaronson, Scott; Grier, Daniel; Schaeffer, Luke (2015). "The Classification of Reversible Bit Operations". arXiv:1504.05155 [quant-ph].
- ↑ Aaronson, Scott (2002). "Quantum Lower Bound for Recursive Fourier Sampling". Quantum Information and Computation ():, 00 3 (2): 165–174. doi:10.26421/QIC3.2-7. Bibcode: 2002quant.ph..9060A.
- ↑ Nielsen, Michael; Chuang, Isaac. "Quantum Computation and Quantum Information"
 |

