Zhao Youqin's π algorithm

Zhao Youqin's π algorithm

A page from Zhao Youqin's book Ge Xiang Xin Shu vol 5

Zhao Youqin's π algorithm was an algorithm devised by Yuan dynasty Chinese astronomer and mathematician Zhao Youqin (赵友钦, ? – 1330) to calculate the value of π in his book Ge Xiang Xin Shu (革象新书).

Algorithm

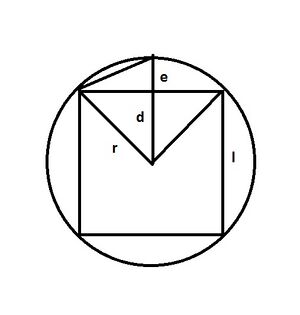
Zhao Youqin started with an inscribed square in a circle with radius r.

If $\ell$ denotes the length of a side of the square, draw a perpendicular line d from the center of the circle to side l. Let e denotes r − d. Then from the diagram:

$d=\sqrt{r^2-{\left(\frac{\ell }{2}\right)}^2}$

$e=r-d=r-\sqrt{r^2-{\left(\frac{\ell }{2}\right)}^2}.$

Extend the perpendicular line d to dissect the circle into an octagon; ${\ell }_2$ denotes the length of one side of octagon.

${\ell }_2=\sqrt{{\left(\frac{\ell }{2}\right)}^2+e^2}$

${\ell }_2=\frac{1}{2}\sqrt{{\ell }^2+4{(r-\frac{1}{2}\sqrt{4r^2-{\ell }^2})}^2}$

Let $l_3$ denotes the length of a side of hexadecagon

${\ell }_3=\frac{1}{2}\sqrt{{\ell }_2^2+4{(r-\frac{1}{2}\sqrt{4r^2-{\ell }_2^2})}^2}$

similarly

${\ell }_{n+1}=\frac{1}{2}\sqrt{{\ell }_n^2+4{(r-\frac{1}{2}\sqrt{4r^2-{\ell }_n^2})}^2}$

Proceeding in this way, he at last calculated the side of a 16384-gon, multiplying it by 16384 to obtain 3141.592 for a circle with diameter = 1000 units, or

$\pi =3.141592.$

He multiplied this number by 113 and obtained 355. From this he deduced that of the traditional values of π, that is 3, 3.14, ⁠22/7⁠ and ⁠355/113⁠, the last is the most exact.

See also

• Liu Hui's π algorithm