Engineering:Pressure tank
A pressure tank or pressurizer is used in a piping system to maintain a desired pressure. Applications include buffering water pressure in homes.[1]
A simple well water control system
Referring to the figure on the left, a submersible water pump is installed in a well. The pressure switch turns the water pump on when it senses a pressure that is less than Plo and turns it off when it senses a pressure greater than Phi. While the pump is on, the pressure tank fills up. The pressure tank is then depleted as it supplies water in the specified pressure range to prevent "short-cycling", in which the pump tries to establish the proper pressure by rapidly cycling between Plo and Phi.
A simple pressure tank would be just a tank which held water with an air space above the water which would compress as more water entered the tank. Modern systems isolate the water from the pressurized air using a flexible rubber or plastic diaphragm or bladder, because otherwise the air will dissolve in the water and be removed from the tank by usage. Eventually there will be little or no air and the tank will become "waterlogged" causing short-cycling, and will need to be drained to restore operation. The diaphragm or bladder may itself exert a pressure on the water, but it is usually small and will be neglected in the following discussion.
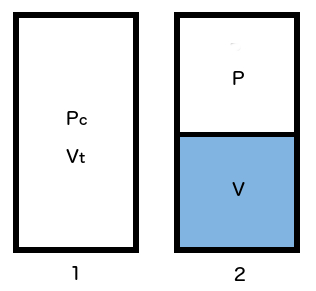
Referring to the diagram on the right, a pressure tank is generally pressurized when empty with a "charging pressure" Pc, which is usually about 2 psi below the turn-on pressure Plo (Case 1). The total volume of the tank is Vt. When in use, the air in the tank will be compressed to pressure P and there will be a volume V of water in the tank (Case 2). In the following development, all pressures are gauge pressures, which are the pressures above atmospheric pressure (Pa, which is altitude dependent). The ideal gas law may be written for both cases, and the amount of air in each case is equal:
- [math]\displaystyle{ (P_c+P_a)V_t = NkT \qquad \text{Case 1} }[/math]
- [math]\displaystyle{ (P+P_a)(V_t-V) = NkT \qquad \mathrm{Case 2} }[/math]
where N is the number of molecules of gas (equal in both cases), k is Boltzmann's Constant and T is the temperature in Kelvin. Assuming that the temperature is equal for both cases, the above equations can be solved for the water pressure/volume relationship in the tank:
- [math]\displaystyle{ V(P) = V_t\frac{P-P_c}{P+P_a} }[/math]
- [math]\displaystyle{ P(V) = \frac{P_a V+P_c V_t}{V_t-V} }[/math]
Tanks are generally specified by their total volume Vt and the "drawdown" (ΔV ), which is the amount of water the tank will eject as the tank pressure goes from Phi to Plo, which are established by the pressure switch:[2][3]
- [math]\displaystyle{ \Delta V =V(P_{hi}) - V(P_{lo}) = V_t \frac{(P_a+P_c)(P_{hi}-P_{lo})}{(P_{hi}+P_a)(P_{lo}+P_a)} }[/math]
The reason for the charging pressure can now be seen: The larger the charging pressure, the larger the drawdown. However, a charging pressure above Plo will not allow the pump to turn on when the water pressure is below Plo, so it is kept a bit below Plo. Another important parameter is the drawdown factor (fΔV), which is the ratio of the drawdown to the total tank volume:
- [math]\displaystyle{ f_{\Delta V}= \frac{(P_a+P_c)(P_{hi}-P_{lo})}{(P_{hi}+P_a)(P_{lo}+P_a)} }[/math]
This factor is independent of the tank size so that the drawdown can be calculated for any tank, given its total volume, atmospheric pressure, charging pressure, and the limiting pressures established by the pressure switch.
See also
References
- ↑ "Pressure Tanks - Do I need one?". 2022-09-30. https://justwaterpumps.com.au/blog/pressure-tanks-do-i-need-one-/.
- ↑ Pelican, Bob (Feb 1, 2006). "How to Calculate and Control Pressure Tank Drawdown". The Driller. https://www.thedriller.com/articles/84709-how-to-calculate-and-control-pressure-tank-drawdown. Retrieved May 29, 2022.
- ↑ Drawdown is more simply expressed in absolute pressures:
- [math]\displaystyle{ \Delta V = V_t P_c \left(\frac{1}{P_{lo}}-\frac{1}{P_{hi}}\right) }[/math]
Bibliography
- Calder, N. (2005). Boatowner's Mechanical and Electrical Manual: How to Maintain, Repair, and Improve Your Boat's Essential Systems. McGraw-Hill Education. ISBN 978-0-07-178406-1. https://books.google.com/books?id=wyc1TfAN-0cC. Retrieved 2022-09-30.
External links
- Direct, Tanks. "Submersible Water Pumps - Sump Pumps". https://www.tanks-direct.co.uk/water-tanks/pumps/submersible-pumps/c1040?msclkid=e5cebfdffa811c204e30dce95224ebae&utm_source=bing&utm_medium=cpc&utm_campaign=Search-Water-Pumps&utm_term=water%20pumps&utm_content=Search-Water-Pumps-Submersible%20Water%20Pumps.
 |