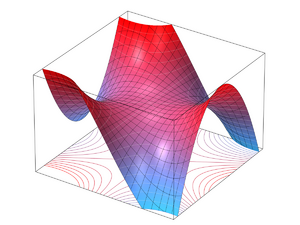
Monkey saddle
In mathematics, the monkey saddle is the surface defined by the equation
- [math]\displaystyle{ z = x^3 - 3xy^2, \, }[/math]
or in cylindrical coordinates
- [math]\displaystyle{ z = \rho^3 \cos(3\varphi). }[/math]
It belongs to the class of saddle surfaces, and its name derives from the observation that a saddle for a monkey would require two depressions for the legs and one for the tail. The point [math]\displaystyle{ (0,0,0) }[/math] on the monkey saddle corresponds to a degenerate critical point of the function [math]\displaystyle{ z(x,y) }[/math] at [math]\displaystyle{ (0, 0) }[/math]. The monkey saddle has an isolated umbilical point with zero Gaussian curvature at the origin, while the curvature is strictly negative at all other points.
One can relate the rectangular and cylindrical equations using complex numbers [math]\displaystyle{ x+iy = r e^{i\varphi}: }[/math]
- [math]\displaystyle{ z = x^3 - 3xy^2 = \operatorname{Re} [(x+iy)^3] = \operatorname{Re}[r^3 e^{3i\varphi}] = r^3\cos(3\varphi). }[/math]
By replacing 3 in the cylindrical equation with any integer [math]\displaystyle{ k \geq 1, }[/math] one can create a saddle with [math]\displaystyle{ k }[/math] depressions. [1]
Another orientation of the monkey saddle is the Smelt petal defined by [math]\displaystyle{ x+y+z+xyz=0, }[/math] so that the z-axis of the monkey saddle corresponds to the direction [math]\displaystyle{ (1,1,1) }[/math] in the Smelt petal.[2][3]
Horse saddle
The term horse saddle may be used in contrast to monkey saddle, to designate an ordinary saddle surface in which z(x,y) has a saddle point, a local minimum or maximum in every direction of the xy-plane. In contrast, the monkey saddle has a stationary point of inflection in every direction.
References
- ↑ Peckham, S.D. (2011) Monkey, starfish and octopus saddles, Proceedings of Geomorphometry 2011, Redlands, CA, pp. 31-34, https://www.researchgate.net/publication/256808897_Monkey_Starfish_and_Octopus_Saddles
- ↑ J., Rimrott, F. P. (1989). Introductory Attitude Dynamics. New York, NY: Springer New York. pp. 26. ISBN 9781461235026. OCLC 852789976.
- ↑ Chesser, H.; Rimrott, F.P.J. (1985). Rasmussen, H.. ed. "Magnus Triangle and Smelt Petal". CANCAM '85: Proceedings, Tenth Canadian Congress of Applied Mechanics, June 2-7, 1985, the University of Western Ontario, London, Ontario, Canada.
External links
 |