Physics:Miles-Phillips mechanism
In physical oceanography and fluid mechanics, the Miles-Phillips mechanism describes the generation of wind waves from a flat surface by the use of two distinct mechanisms. Both mechanisms are applied on the gravity-capillary waves and have in common that waves are generated by a resonance phenomenon. The Miles mechanism is based on the hypothesis that waves arise as an instability of the sea-atmosphere system. The Phillips mechanism assumes that turbulent eddies in the atmospheric boundary layer induce pressure fluctuations at the sea surface.
History
It was Jeffreys.[1] in 1925 who was the first to produce a plausible explanation for the shift in atmospheric pressure necessary for the energy transfer from the wind to the waves. He assumed that this energy transfer was caused by form drag linked to flow separation. Furthermore, he assumed that flow separation occurred on the downwind side of the wave crests. With the use of dimensional analysis, Jeffreys showed that the shift in pressure can be displayed as [math]\displaystyle{ p = S \rho_a (U_{\infty} - C)^2 \frac{\partial \eta}{\partial x} }[/math]
where [math]\displaystyle{ S }[/math] the sheltering coefficient, [math]\displaystyle{ \rho_a }[/math] the density of the atmosphere, [math]\displaystyle{ U_{\infty} }[/math] the wind speed, [math]\displaystyle{ C }[/math] the wind phase speed and [math]\displaystyle{ \eta }[/math] the free surface elevation is. The subscript of [math]\displaystyle{ \infty }[/math] is used to make the distinction that no boundary layer is considered in this theory. Expanding this pressure term to the energy transfer yields [math]\displaystyle{ \frac{\partial E}{\partial t} = \frac{1}{2\rho_w g} S \rho_a (U_{\infty} - C)^2 (ak)^2 C }[/math]
where [math]\displaystyle{ \rho_w }[/math] the density of the water, [math]\displaystyle{ g }[/math] the gravitational acceleration, [math]\displaystyle{ a }[/math] the wave amplitude and [math]\displaystyle{ k }[/math] the wavenumber is.
With this theory Jeffreys calculated the sheltering coefficient at a value of 0.3 based on observations of wind speeds.
Ursell[2] examined in 1956 available data on pressure variation from multiple sources and concluded that the value of [math]\displaystyle{ S }[/math] found by Jeffreys was too large. This result led to the rejection of the theory from Jeffreys.
Ursell's work also resulted to new advances in the search for a plausible mechanism for wind generated waves. These advances led a year later to two new theoretical concepts: the Miles and Phillips mechanisms.
Miles' Theory
John W. Miles developed his theory in 1957[3] for inviscid, incompressible air and water. He made the assumption that the air can be expressed as a mean shear flow with varying height above the surface.
By solving the equations for the coupled sea-atmosphere system, Miles was able to express the free surface elevation as a function of wave parameters and sea-atmosphere characteristics as
[math]\displaystyle{ \eta = a \exp\left[\frac{1}{2} \rho_a / \rho_w \beta k C_w \left(\frac{U_r}{C_w}\right)^2 t \right] \exp[i(kx - \omega t)] }[/math]
where [math]\displaystyle{ \beta }[/math] the scale parameter, [math]\displaystyle{ C_w }[/math] the phase speed of free gravity waves, [math]\displaystyle{ U_r }[/math] a reference wind speed and [math]\displaystyle{ \omega }[/math] the angular frequency of the wave is.
Furthermore, Miles defined the growth rate of the energy [math]\displaystyle{ \gamma }[/math] as
[math]\displaystyle{ \gamma = (\rho_a/\rho_w)\beta \omega \left(\frac{U_r}{C_w} \cos\phi\right)^2 }[/math]
In his 1957 paper, Miles determined [math]\displaystyle{ \gamma }[/math] by solving the inviscid form of the Orr-Sommerfeld equation.
Miles further expanded his theory on the growth rate of wind driven waves by finding an expression for the dimensionless growth rate [math]\displaystyle{ \frac{\gamma}{\sigma} }[/math] at a critical height [math]\displaystyle{ z_c }[/math] above the surface where the wind speed [math]\displaystyle{ U }[/math] equal is to the phase speed of the gravity waves [math]\displaystyle{ C_w }[/math].
[math]\displaystyle{ \frac{\gamma}{\sigma} = -\frac{\pi}{2k}(\rho_a/\rho_w)|\chi|^2 \frac{\left(\frac{\partial^2 U_r}{\partial z^2}\right)_{z=z_c}}{\left(\frac{\partial U_r}{\partial z}\right)_{z=z_c}} }[/math]
with [math]\displaystyle{ \sigma }[/math] the frequency of the wave and [math]\displaystyle{ \chi }[/math] the dimensional amplitude of the wave-induced velocity field.
This result represents Miles' classical result for the growth of surface waves due to shear flow. Despite the fact that this theory gives an accurate description of the transfer of energy from the wind to the waves, it also has some limitations
- Miles considered the case of inviscid air and water, which means that viscous effects are neglected in this case.
- The effects that waves have on the atmospheric boundary layer are not taken into account.
- Only the case of linear effects are examined with this theory.
- Miles theory predicts growth of waves for all wind speeds, observations show however that there exist a minimum wind speed before growth occurs[4]
In a subsequent collaboration with Conte[5] (1959), the wind speed as a function of height was found by integrating the Orr-Sommerfeld equation with the assumption of a logarithmic boundary layer and that in the equilibrium state no current below the sea surface exist [math]\displaystyle{ U(z) = \frac{u_*}{\kappa} \log\left(1 + \frac{z}{z_0}\right) }[/math]
where [math]\displaystyle{ \kappa }[/math] the von Karman's constant, [math]\displaystyle{ u_* = (\tau/\rho_a)^{1/2} }[/math] the friction velocity, [math]\displaystyle{ \tau }[/math] the Reynolds' stress and [math]\displaystyle{ z_0 }[/math] the roughness length is.
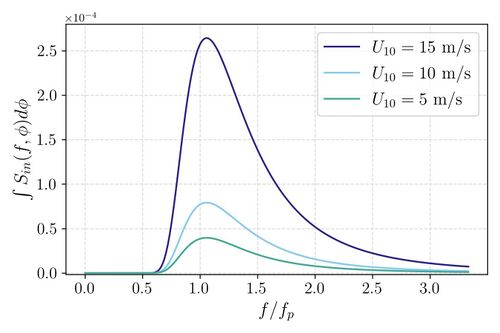
The atmospheric input from the wind to the waves is represented by [math]\displaystyle{ S_{in} }[/math]. For the theory from Miles, Snyder and Cox[6] (1967) were the first to produce a relationship for the experimental growth rate due to atmospheric forcing by use of experimental data. They found
[math]\displaystyle{ S_{in}(f,\phi) = (\rho_a/\rho_w) \beta \omega \left(\frac{U_{10}}{C}\cos\phi-1\right)^2 F(f,\phi) }[/math]
where [math]\displaystyle{ U_{10} }[/math] the wind speed measured at a height of 10 meters, [math]\displaystyle{ \phi }[/math] the angle between the wind and the waves and [math]\displaystyle{ F(f,\phi) }[/math] a spectrum of the form of the JONSWAP.
Phillips' Theory
At the same time, but independently from Miles, Phillips[7] (1957) developed his theory for the generation of waves based on the resonance between a fluctuating pressure field and surface waves. The main idea behind Phillips' theory is that this resonance mechanism causes the waves to grow when the length of the waves matches the length of the atmospheric pressure fluctuations. This means that that energy will be transferred to the components in the spectrum which satisfy the resonance condition.
Phillips determined the atmospheric source term for his theory as the following
[math]\displaystyle{ S_{in}(f,\phi)=\frac{2\pi^2\omega}{\rho_w^2 C^3 C_g} \Pi(\mathbf{k},\omega) }[/math]
where [math]\displaystyle{ \Pi(\mathbf{k},\omega) }[/math] is the frequency spectrum, with the three dimensional wave number [math]\displaystyle{ \mathbf{k} }[/math].
The strong points from this theory are that waves can grow from a initially smooth surface, so don't need the presence of surface waves. In addition, contrary to Miles' theory, this theory does predict that no wave growth can occur if the wind speed is below a certain value.
Miles his theory predicts an exponential growth with time, the theory from Phillips however predicts a linear growth with time. The linear growth of the wave is especially observed in the earliest stages of wave growth. For later stages, Miles' exponential growth is more consistent with observations.
See also
- Gravity waves
- Wind waves
- Wind stress
- Swell
References
- ↑ Jeffreys, H. (1925). "On the formation of water waves by wind". Proceedings of the Royal Society: 341-347. doi:10.1098/rspa.1925.0015.
- ↑ Ursell, F. (1956). "Wave generation by wind". Surveys in Mechanics: 216-249.
- ↑ Miles, J. (1957). "On the generation of surface waves by shear flows". Journal of Fluid Mechanics: 185-204. doi:10.1017/S0022112057000567.
- ↑ Janssen, P. (2004). The interaction of ocean waves and wind. Cambridge University Press. pp. 88-89. ISBN 9780521465403.
- ↑ Miles, J.; Conte, S. (1959). "On the Numerical Integration of the Orr-Sommerfeld Equation". Journal of the Society for Industrial and Applied Mathematics: 361-366. doi:10.1137/0107030.
- ↑ Snyder, R.; Cox, C. (1967). "A field study of the wind generation of ocean waves". Journal of Marine Research: 141-178.
- ↑ Phillips, O. (1957). "On the generation of waves by turbulent wind". Journal of Fluid Mechanics: 417-445. doi:10.1017/S0022112057000233.

