Dinatural transformation
From HandWiki
Short description: Generalization of natural transformations
In category theory, a branch of mathematics, a dinatural transformation [math]\displaystyle{ \alpha }[/math] between two functors
- [math]\displaystyle{ S,T : C^{\mathrm{op}}\times C\to D, }[/math]
written
- [math]\displaystyle{ \alpha : S\ddot\to T, }[/math]
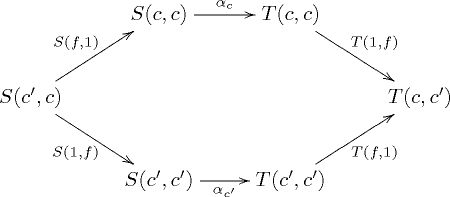
is a function that to every object [math]\displaystyle{ c }[/math] of [math]\displaystyle{ C }[/math] associates an arrow
- [math]\displaystyle{ \alpha_c : S(c,c)\to T(c,c) }[/math] of [math]\displaystyle{ D }[/math]
and satisfies the following coherence property: for every morphism [math]\displaystyle{ f:c\to c' }[/math] of [math]\displaystyle{ C }[/math] the diagram
commutes.[1]
The composition of two dinatural transformations need not be dinatural.
See also
References
- ↑ Mac Lane, Saunders (2013). Categories for the working mathematician. Springer Science & Business Media. p. 218.
External links
 |