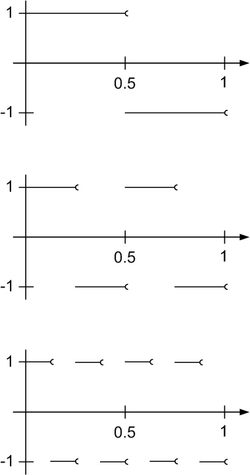
Rademacher system

In mathematics, in particular in functional analysis, the Rademacher system, named after Hans Rademacher, is an incomplete orthogonal system of functions on the unit interval of the following form:

[math]\displaystyle{ \{ t \mapsto r_{n}(t)=\sgn ( \sin 2^{n+1} \pi t ) ; t \in [0,1], n \in \N \}. }[/math]

The Rademacher system is stochastically independent, and is closely related to the Walsh system. Specifically, the Walsh system can be constructed as a product of Rademacher functions.

References

• Rademacher, Hans (1922). "Einige Sätze über Reihen von allgemeinen Orthogonalfunktionen". Math. Ann. 87 (1): 112–138. doi:10.1007/BF01458040. 
• Hazewinkel, Michiel, ed. (2001), "Orthogonal system", Encyclopedia of Mathematics, Springer Science+Business Media B.V. / Kluwer Academic Publishers, ISBN 978-1-55608-010-4, https://www.encyclopediaofmath.org/index.php?title=Main_Page 
• Heil, Christopher E. (1997). "A basis theory primer". http://www.math.gatech.edu/~heil/papers/bases.pdf. 
• Curbera, Guillermo P. (2009). "How Summable are Rademacher Series?". Vector Measures, Integration and Related Topics. Basel: Birkhäuser Basel. pp. 135–148. doi:10.1007/978-3-0346-0211-2_13. ISBN 978-3-0346-0210-5. 

External links

• Rademacher system in the Encyclopedia of Mathematics