Bimonster group
From HandWiki
In mathematics, the bimonster is a group that is the wreath product of the monster group M with Z2:
- [math]\displaystyle{ Bi = M \wr \mathbb{Z}_2. \, }[/math]
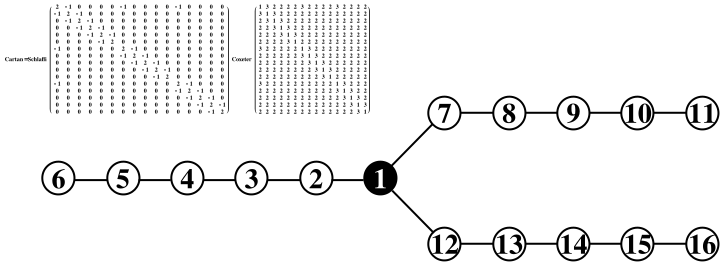
The Bimonster is also a quotient of the Coxeter group corresponding to the Dynkin diagram Y555, a Y-shaped graph with 16 nodes:
- Script error: No such module "Dynkin".
John H. Conway conjectured that a presentation of the bimonster could be given by adding a certain extra relation to the presentation defined by the Y555 diagram; this was proved in 1990 by A. A. Ivanov a mathematician not the famous painter and Simon P. Norton.
See also
- Triality - simple Lie group D4, Y111
- Affine E 6 Y222
References
- Basak, Tathagata (2007), "The complex Lorentzian Leech lattice and the Bimonster", Journal of Algebra 309 (1): 32–56, doi:10.1016/j.jalgebra.2006.05.033.
- "The Bimonster, the group Y555, and the projective plane of order 3", Computers in Algebra (Chicago, IL, 1985), Lecture Notes in Pure and Applied Mathematics, 111, New York: Dekker, 1988, pp. 27–50.
- "Hyperbolic reflections for the Bimonster and 3Fi24", Groups, Combinatorics & Geometry (Durham, 1990), London Math. Soc. Lecture Note Ser., 165, Cambridge: Cambridge University Press, 1992, pp. 24–45, doi:10.1017/CBO9780511629259.006.
- "26 implies the Bimonster", Journal of Algebra 235 (2): 805–814, 2001, doi:10.1006/jabr.2000.8494.
- Simons, Christopher Smyth (1997), Hyperbolic reflection groups, completely replicable functions, the Monster and the Bimonster, Ph.D. thesis, Princeton University, Department of Mathematics, ISBN 978-0591-50546-7.
- Soicher, Leonard H. (1989), "From the Monster to the Bimonster", Journal of Algebra 121 (2): 275–280, doi:10.1016/0021-8693(89)90064-1.
External links
- Weisstein, Eric W.. "Bimonster". http://mathworld.wolfram.com/Bimonster.html. (Note: incorrectly named here as [36,6,6])
 |