Biryukov equation

Short description: Non-linear second-order differential equation

Sine oscillations F = 0.01

In the study of dynamical systems, the Biryukov equation (or Biryukov oscillator), named after Vadim Biryukov (1946), is a non-linear second-order differential equation used to model damped oscillators.

The equation is given by $$\frac{d^{2}y}{d{t}^2}+f(y)\frac{dy}{dt}+y=0,(1)$$

where ƒ(y) is a piecewise constant function which is positive, except for small y as

$$\begin{array}{rl}& f(y)=\{\begin{array}{ll}-F,& |y|\le Y_0;\\ F,& |y|>Y_0.\end{array}\\ & F=\text{const.}>0,Y_0=\text{const.}>0.\end{array}$$

Eq. (1) is a special case of the Lienard equation; it describes the auto-oscillations.

Solution (1) at separate time intervals when f(y) is constant is given by

$$y_k(t)=A_{1,k}\exp (s_{1,k}t)+A_{2,k}\exp (s_{2,k}t)(2)$$

where exp denotes the exponential function. Here $$s_k=\{\begin{array}{ll}\frac{F}{2}\mp \sqrt{{\left(\frac{F}{2}\right)}^2-1},& |y|<Y_0;\\ -\frac{F}{2}\mp \sqrt{{\left(\frac{F}{2}\right)}^2-1}& \text{otherwise.}\end{array}$$ Expression (2) can be used for real and complex values of s_k.

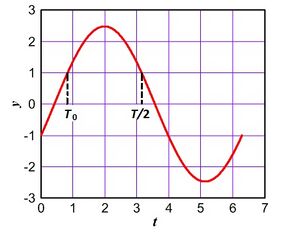
The first half-period’s solution at

$y(0)=\pm Y_0$

is

Relaxation oscillations F = 4

$$\begin{array}{rl}y(t)& =\{\begin{array}{ll}{y}_1(t),& 0\le t<T_0;\\ y_2(t),& T_0\le t<\frac{T}{2}.\end{array}\\ y_1(t)& =A_{1,k}\cdot \exp (s_{1,k}t)+A_{2,k}\cdot \exp (s_{2,k}t),\\ y_2(t)& =A_{3,k}\cdot \exp (s_{3,k}t)+A_{4,k}\cdot \exp (s_{4,k}t).\end{array}$$

The second half-period’s solution is

$$y(t)=\{\begin{array}{ll}-y_1(t-\frac{T}{2}),& \frac{T}{2}\le t<\frac{T}{2}+T_0;\\ -y_2(t-\frac{T}{2}),& \frac{T}{2}+T_0\le t<T.\end{array}$$

The solution contains four constants of integration A₁, A₂, A₃, A₄, the period T and the boundary T₀ between y₁(t) and y₂(t) needs to be found. A boundary condition is derived from the continuity of y(t) and dy/dt.

Solution of (1) in the stationary mode thus is obtained by solving a system of algebraic equations as

$$\begin{array}{lll}& y_1(0)=-Y_0& y_1(T_0)=Y_0\\ & y_2(T_0)=Y_0& y_2\left(\frac{T}{2}\right)=Y_0\\ & {\frac{d{y}_1}{dt}|}_{T_0}={\frac{d{y}_2}{dt}|}_{T_0}& {\frac{d{y}_1}{dt}|}_0=-{\frac{d{y}_2}{dt}|}_{\frac{T}{2}}\end{array}$$

The integration constants are obtained by the Levenberg–Marquardt algorithm. With $f(y)=\mu (-1+y^2)$, $\mu =\text{const.}>0,$ Eq. (1) named Van der Pol oscillator. Its solution cannot be expressed by elementary functions in closed form.