Dodecahedral cupola
From HandWiki
| Dodecahedral cupola | ||
|---|---|---|
 Schlegel diagram | ||
| Type | Polyhedral cupola | |
| Schläfli symbol | {5,3} v rr{5,3} | |
| Cells | 64 | 1 rr{5,3} 1 {5,3} 30px 30 {}×{3} 30px 12 {}×{5} 30px 20 {3,3} |
| Faces | 194 | 80 triangles 90 squares 24 pentagons |
| Edges | 210 | |
| Vertices | 80 | |
| Dual | ||
| Symmetry group | [5,3,1], order 120 | |
| Properties | convex, regular-faced | |
In 4-dimensional geometry, the dodecahedral cupola is a polychoron bounded by a rhombicosidodecahedron, a parallel dodecahedron, connected by 30 triangular prisms, 12 pentagonal prisms, and 20 tetrahedra.[1]
Related polytopes
The dodecahedral cupola can be sliced off from a runcinated 120-cell, on a hyperplane parallel to a dodecahedral cell. The cupola can be seen in a pentagonal centered orthogonal projection of the runcinated 120-cell:
Runcinated 120-cell
|
Dodecahedron (cupola top) |
Rhombicosidodecahedron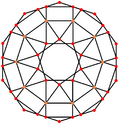 (cupola base) |
See also
References
- ↑ Convex Segmentochora Dr. Richard Klitzing, Symmetry: Culture and Science, Vol. 11, Nos. 1-4, 139-181, 2000 (4.152 dodecahedron || rhombicosidodecahedron)
External links
 |

