Dodecahedral pyramid
From HandWiki
| Dodecahedral pyramid | ||
|---|---|---|
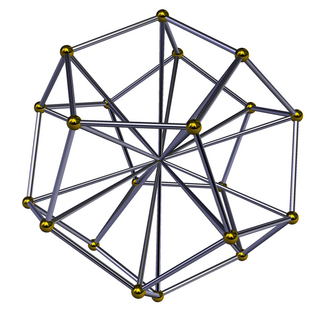 Schlegel diagram | ||
| Type | Polyhedral pyramid | |
| Schläfli symbol | ( ) ∨ {5,3} | |
| Cells | 13 | 1 {5,3} 12 ( ) ∨ {5} |
| Faces | 42 | 30 ()v{ } 12 {5} |
| Edges | 50 | |
| Vertices | 21 | |
| Dual | icosahedral pyramid | |
| Symmetry group | H3, [5,3,1], order 120 | |
| Properties | convex | |
In 4-dimensional geometry, the dodecahedral pyramid is bounded by one dodecahedron on the base and 12 pentagonal pyramid cells which meet at the apex. Since a dodecahedron's circumradius is greater than its edge length,[1] the pentagonal pyramids require tall isosceles triangle faces.
The dual to the dodecahedral pyramid is an icosahedral pyramid, seen as an icosahedral base, and 20 regular tetrahedra meeting at an apex.
References
- ↑ Klitzing, Richard. "3D convex uniform polyhedra o3o5x - doe". https://bendwavy.org/klitzing/dimensions/polyhedra.htm. sqrt[(9+3 sqrt(5))/8] ≒ 1.401259
External links
- Olshevsky, George. "Pyramid". Glossary for Hyperspace. Archived from the original on 4 February 2007. https://web.archive.org/web/20070204075028/members.aol.com/Polycell/glossary.html#Pyramid.
- Richard Klitzing, Axial-Symmetrical Edge Facetings of Uniform Polyhedra
 |