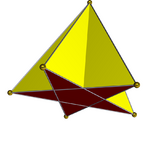
Pentagonal pyramid
| Pentagonal pyramid | |
|---|---|
 | |
| Type | Johnson J1 – J2 – J3 |
| Faces | 5 triangles 1 pentagon |
| Edges | 10 |
| Vertices | 6 |
| Vertex configuration | 5(32.5) (35) |
| Schläfli symbol | ( ) ∨ {5} |
| Symmetry group | C5v, [5], (*55) |
| Rotation group | C5, [5]+, (55) |
| Dual polyhedron | self |
| Properties | convex |
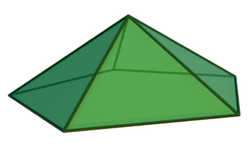
| Net | |
 | |
File:J2 pentagonal pyramid.stl
In geometry, a pentagonal pyramid is a pyramid with a pentagonal base upon which are erected five triangular faces that meet at a point (the apex). Like any pyramid, it is self-dual.
The regular pentagonal pyramid has a base that is a regular pentagon and lateral faces that are equilateral triangles. It is one of the Johnson solids (J2).
It can be seen as the "lid" of an icosahedron; the rest of the icosahedron forms a gyroelongated pentagonal pyramid, J11.
More generally an order-2 vertex-uniform pentagonal pyramid can be defined with a regular pentagonal base and 5 isosceles triangle sides of any height.
Cartesian coordinates
The pentagonal pyramid can be seen as the "lid" of a regular icosahedron; the rest of the icosahedron forms a gyroelongated pentagonal pyramid, J11. From the Cartesian coordinates of the icosahedron, Cartesian coordinates for a pentagonal pyramid with edge length 2 may be inferred as
- [math]\displaystyle{ (1,0,\tau),\,(-1,0,\tau),\,(0,\tau,1),\,(\tau,1,0),(\tau,-1,0),(0,-\tau,1) }[/math]
where Template:Tau (sometimes written as φ) is the golden ratio.[1]
The height H, from the midpoint of the pentagonal face to the apex, of a pentagonal pyramid with edge length a may therefore be computed as:
- [math]\displaystyle{ H = \left(\sqrt{{\frac{5-\sqrt{5}}{10}}}\right)a \approx 0.52573a. }[/math][2]
Its surface area A can be computed as the area of the pentagonal base plus five times the area of one triangle:
- [math]\displaystyle{ A = \frac{a^2}{2}\sqrt{\frac{5}{2}\left(10+\sqrt{5}+\sqrt{75+30\sqrt{5}}\right)} \approx 3.88554 \cdot a^2. }[/math][3][2]
Its volume can be calculated as:
- [math]\displaystyle{ V = \left(\frac{5 + \sqrt{5}}{24}\right)a^3 \approx 0.30150a^3. }[/math][3]
Related polyhedra
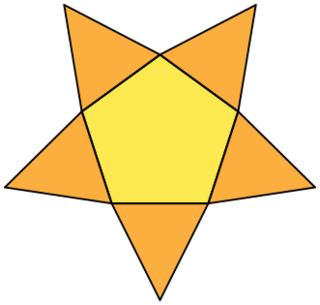
The pentagrammic star pyramid has the same vertex arrangement, but connected onto a pentagram base:
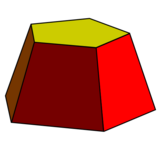 Pentagonal frustum is a pentagonal pyramid with its apex truncated |
 The top of an icosahedron is a pentagonal pyramid |
Example
References
- ↑ Weisstein, Eric W.. "Icosahedral Group" (in en). https://mathworld.wolfram.com/IcosahedralGroup.html.
- ↑ 2.0 2.1 Sapiña, R.. "Area and volume of a pentagonal pyramid and Johnson solid J₂" (in es). Problemas y ecuaciones. ISSN 2659-9899. https://www.problemasyecuaciones.com/geometria3D/volumen/Johnson/J2/calculadora-area-volumen-formulas.html. Retrieved 2020-06-29.
- ↑ 3.0 3.1 Weisstein, Eric W.. "Pentagonal Pyramid" (in en). https://mathworld.wolfram.com/PentagonalPyramid.html.
External links
- Eric W. Weisstein, Pentagonal pyramid (Johnson solid) at MathWorld.
- Virtual Reality Polyhedra www.georgehart.com: The Encyclopedia of Polyhedra ( VRML model)
 |