List of space groups

Short description: None

There are 230 space groups in three dimensions, given by a number index, and a full name in Hermann–Mauguin notation, and a short name (international short symbol). The long names are given with spaces for readability. The groups each have a point group of the unit cell.

Symbols

In Hermann–Mauguin notation, space groups are named by a symbol combining the point group identifier with the uppercase letters describing the lattice type. Translations within the lattice in the form of screw axes and glide planes are also noted, giving a complete crystallographic space group.

These are the Bravais lattices in three dimensions:

• P primitive
• I body centered (from the German Innenzentriert)
• F face centered (from the German Flächenzentriert)
• A centered on A faces only
• B centered on B faces only
• C centered on C faces only
• R rhombohedral

A reflection plane m within the point groups can be replaced by a glide plane, labeled as a, b, or c depending on which axis the glide is along. There is also the n glide, which is a glide along the half of a diagonal of a face, and the d glide, which is along a quarter of either a face or space diagonal of the unit cell. The d glide is often called the diamond glide plane as it features in the diamond structure.

• [math]\displaystyle{ a }[/math], [math]\displaystyle{ b }[/math], or [math]\displaystyle{ c }[/math]: glide translation along half the lattice vector of this face
• [math]\displaystyle{ n }[/math]: glide translation along half the diagonal of this face
• [math]\displaystyle{ d }[/math]: glide planes with translation along a quarter of a face diagonal
• [math]\displaystyle{ e }[/math]: two glides with the same glide plane and translation along two (different) half-lattice vectors.

A gyration point can be replaced by a screw axis denoted by a number, n, where the angle of rotation is [math]\displaystyle{ \color{Black}\tfrac{360^\circ}{n} }[/math]. The degree of translation is then added as a subscript showing how far along the axis the translation is, as a portion of the parallel lattice vector. For example, 2₁ is a 180° (twofold) rotation followed by a translation of ½ of the lattice vector. 3₁ is a 120° (threefold) rotation followed by a translation of ⅓ of the lattice vector. The possible screw axes are: 2₁, 3₁, 3₂, 4₁, 4₂, 4₃, 6₁, 6₂, 6₃, 6₄, and 6₅.

Wherever there is both a rotation or screw axis n and a mirror or glide plane m along the same crystallographic direction, they are represented as a fraction [math]\displaystyle{ \frac{n}{m} }[/math] or n/m. For example, 4₁/a means that the crystallographic axis in question contains both a 4₁ screw axis as well as a glide plane along a.

In Schoenflies notation, the symbol of a space group is represented by the symbol of corresponding point group with additional superscript. The superscript doesn't give any additional information about symmetry elements of the space group, but is instead related to the order in which Schoenflies derived the space groups. This is sometimes supplemented with a symbol of the form [math]\displaystyle{ \Gamma_x^y }[/math] which specifies the Bravais lattice. Here [math]\displaystyle{ x \in \{t, m, o, q, rh, h, c\} }[/math] is the lattice system, and [math]\displaystyle{ y \in \{\empty, b, v, f\} }[/math] is the centering type.

In Fedorov symbol, the type of space group is denoted as s (symmorphic ), h (hemisymmorphic), or a (asymmorphic). The number is related to the order in which Fedorov derived space groups. There are 73 symmorphic, 54 hemisymmorphic, and 103 asymmorphic space groups.

Symmorphic

The 73 symmorphic space groups can be obtained as combination of Bravais lattices with corresponding point group. These groups contain the same symmetry elements as the corresponding point groups, for example, the space groups P4/mmm ([math]\displaystyle{ P\tfrac{4}{m}\tfrac{2}{m}\tfrac{2}{m} }[/math], 36s) and I4/mmm ([math]\displaystyle{ I\tfrac{4}{m}\tfrac{2}{m}\tfrac{2}{m} }[/math], 37s).

Hemisymmorphic

The 54 hemisymmorphic space groups contain only axial combination of symmetry elements from the corresponding point groups. Hemisymmorphic space groups contain the axial combination 422, which are P4/mcc ([math]\displaystyle{ P\tfrac{4}{m}\tfrac{2}{c}\tfrac{2}{c} }[/math], 35h), P4/nbm ([math]\displaystyle{ P\tfrac{4}{n}\tfrac{2}{b}\tfrac{2}{m} }[/math], 36h), P4/nnc ([math]\displaystyle{ P\tfrac{4}{n}\tfrac{2}{n}\tfrac{2}{c} }[/math], 37h), and I4/mcm ([math]\displaystyle{ I\tfrac{4}{m}\tfrac{2}{c}\tfrac{2}{m} }[/math], 38h).

Asymmorphic

The remaining 103 space groups are asymmorphic, for example, those derived from the point group 4/mmm ([math]\displaystyle{ \tfrac{4}{m}\tfrac{2}{m}\tfrac{2}{m} }[/math]).

List of triclinic

Triclinic Bravais lattice

Triclinic crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Fibrifold
1	1	[math]\displaystyle{ 1 }[/math]	P1	P 1	[math]\displaystyle{ \Gamma_tC_1^1 }[/math]	1s	[math]\displaystyle{ (a/b/c)\cdot 1 }[/math]	[math]\displaystyle{ (\circ) }[/math]
2	1	[math]\displaystyle{ \times }[/math]	P1	P 1	[math]\displaystyle{ \Gamma_tC_i^1 }[/math]	2s	[math]\displaystyle{ (a/b/c)\cdot \tilde 2 }[/math]	[math]\displaystyle{ (2222) }[/math]

List of monoclinic

Monoclinic Bravais lattice

Simple (P)	Base (C)

Monoclinic crystal system

Number	Point group	Orbifold	Short name	Full name(s)		Schoenflies	Fedorov	Shubnikov	Fibrifold (primary)	Fibrifold (secondary)
3	2	[math]\displaystyle{ 22 }[/math]	P2	P 1 2 1	P 1 1 2	[math]\displaystyle{ \Gamma_mC_2^1 }[/math]	3s	[math]\displaystyle{ (b:(c/a)):2 }[/math]	[math]\displaystyle{ (2_02_02_02_0) }[/math]	[math]\displaystyle{ ({*}_0{*}_0) }[/math]
4			P21	P 1 21 1	P 1 1 21	[math]\displaystyle{ \Gamma_mC_2^2 }[/math]	1a	[math]\displaystyle{ (b:(c/a)):2_1 }[/math]	[math]\displaystyle{ (2_12_12_12_1) }[/math]	[math]\displaystyle{ (\bar{\times}\bar{\times}) }[/math]
5			C2	C 1 2 1	B 1 1 2	[math]\displaystyle{ \Gamma_m^bC_2^3 }[/math]	4s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}/b:(c/a)\right ) :2 }[/math]	[math]\displaystyle{ (2_02_02_12_1) }[/math]	[math]\displaystyle{ ({*}_1{*}_1) }[/math], [math]\displaystyle{ ({*}\bar{\times}) }[/math]
6	m	[math]\displaystyle{ * }[/math]	Pm	P 1 m 1	P 1 1 m	[math]\displaystyle{ \Gamma_mC_s^1 }[/math]	5s	[math]\displaystyle{ (b:(c/a))\cdot m }[/math]	[math]\displaystyle{ [\circ_0] }[/math]	[math]\displaystyle{ ({*}{\cdot}{*}{\cdot}) }[/math]
7			Pc	P 1 c 1	P 1 1 b	[math]\displaystyle{ \Gamma_mC_s^2 }[/math]	1h	[math]\displaystyle{ (b:(c/a))\cdot \tilde c }[/math]	[math]\displaystyle{ (\bar\circ_0) }[/math]	[math]\displaystyle{ ({*}{:}{*}{:}) }[/math], [math]\displaystyle{ ({\times}{\times}_0) }[/math]
8			Cm	C 1 m 1	B 1 1 m	[math]\displaystyle{ \Gamma_m^bC_s^3 }[/math]	6s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}/b:(c/a)\right ) \cdot m }[/math]	[math]\displaystyle{ [\circ_1] }[/math]	[math]\displaystyle{ ({*}{\cdot}{*}{:}) }[/math], [math]\displaystyle{ ({*}{\cdot}{\times}) }[/math]
9			Cc	C 1 c 1	B 1 1 b	[math]\displaystyle{ \Gamma_m^bC_s^4 }[/math]	2h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}/b:(c/a)\right ) \cdot \tilde c }[/math]	[math]\displaystyle{ (\bar\circ_1) }[/math]	[math]\displaystyle{ ({*}{:}{\times}) }[/math], [math]\displaystyle{ ({\times}{\times}_1) }[/math]
10	2/m	[math]\displaystyle{ 2* }[/math]	P2/m	P 1 2/m 1	P 1 1 2/m	[math]\displaystyle{ \Gamma_mC_{2h}^1 }[/math]	7s	[math]\displaystyle{ (b:(c/a))\cdot m:2 }[/math]	[math]\displaystyle{ [2_02_02_02_0] }[/math]	[math]\displaystyle{ [*2{\cdot}22{\cdot}2) }[/math]
11			P21/m	P 1 21/m 1	P 1 1 21/m	[math]\displaystyle{ \Gamma_mC_{2h}^2 }[/math]	2a	[math]\displaystyle{ (b:(c/a))\cdot m:2_1 }[/math]	[math]\displaystyle{ [2_12_12_12_1] }[/math]	[math]\displaystyle{ (22{*}{\cdot}) }[/math]
12			C2/m	C 1 2/m 1	B 1 1 2/m	[math]\displaystyle{ \Gamma_m^bC_{2h}^3 }[/math]	8s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}/b:(c/a)\right ) \cdot m:2 }[/math]	[math]\displaystyle{ [2_02_02_12_1] }[/math]	[math]\displaystyle{ (*2{\cdot}22{:}2) }[/math], [math]\displaystyle{ (2\bar{*}2{\cdot}2) }[/math]
13			P2/c	P 1 2/c 1	P 1 1 2/b	[math]\displaystyle{ \Gamma_mC_{2h}^4 }[/math]	3h	[math]\displaystyle{ (b:(c/a))\cdot \tilde c:2 }[/math]	[math]\displaystyle{ (2_02_022) }[/math]	[math]\displaystyle{ (*2{:}22{:}2) }[/math], [math]\displaystyle{ (22{*}_0) }[/math]
14			P21/c	P 1 21/c 1	P 1 1 21/b	[math]\displaystyle{ \Gamma_mC_{2h}^5 }[/math]	3a	[math]\displaystyle{ (b:(c/a))\cdot \tilde c:2_1 }[/math]	[math]\displaystyle{ (2_12_122) }[/math]	[math]\displaystyle{ (22{*}{:}) }[/math], [math]\displaystyle{ (22{\times}) }[/math]
15			C2/c	C 1 2/c 1	B 1 1 2/b	[math]\displaystyle{ \Gamma_m^bC_{2h}^6 }[/math]	4h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}/b:(c/a)\right ) \cdot \tilde c:2 }[/math]	[math]\displaystyle{ (2_02_122) }[/math]	[math]\displaystyle{ (2\bar{*}2{:}2) }[/math], [math]\displaystyle{ (22{*}_1) }[/math]

List of orthorhombic

Orthorhombic Bravais lattice

Simple (P)	Body (I)	Face (F)	Base (A or C)

Orthorhombic crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Fibrifold (primary)	Fibrifold (secondary)
16	222	[math]\displaystyle{ 222 }[/math]	P222	P 2 2 2	[math]\displaystyle{ \Gamma_oD_2^1 }[/math]	9s	[math]\displaystyle{ (c:a:b):2:2 }[/math]	[math]\displaystyle{ (*2_02_02_02_0) }[/math]
17			P2221	P 2 2 21	[math]\displaystyle{ \Gamma_oD_2^2 }[/math]	4a	[math]\displaystyle{ (c:a:b):2_1:2 }[/math]	[math]\displaystyle{ (*2_12_12_12_1) }[/math]	[math]\displaystyle{ (2_02_0{*}) }[/math]
18			P21212	P 21 21 2	[math]\displaystyle{ \Gamma_oD_2^3 }[/math]	7a	[math]\displaystyle{ (c:a:b):2 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (2_02_0\bar{\times}) }[/math]	[math]\displaystyle{ (2_12_1{*}) }[/math]
19			P212121	P 21 21 21	[math]\displaystyle{ \Gamma_oD_2^4 }[/math]	8a	[math]\displaystyle{ (c:a:b):2_1 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (2_12_1\bar{\times}) }[/math]
20			C2221	C 2 2 21	[math]\displaystyle{ \Gamma_o^bD_2^5 }[/math]	5a	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) :2_1:2 }[/math]	[math]\displaystyle{ (2_1{*}2_12_1) }[/math]	[math]\displaystyle{ (2_02_1{*}) }[/math]
21			C222	C 2 2 2	[math]\displaystyle{ \Gamma_o^bD_2^6 }[/math]	10s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) :2:2 }[/math]	[math]\displaystyle{ (2_0{*}2_02_0) }[/math]	[math]\displaystyle{ (*2_02_02_12_1) }[/math]
22			F222	F 2 2 2	[math]\displaystyle{ \Gamma_o^fD_2^7 }[/math]	12s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:c:a:b\right ) :2:2 }[/math]	[math]\displaystyle{ (*2_02_12_02_1) }[/math]
23			I222	I 2 2 2	[math]\displaystyle{ \Gamma_o^vD_2^8 }[/math]	11s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b\right ) :2:2 }[/math]	[math]\displaystyle{ (2_1{*}2_02_0) }[/math]
24			I212121	I 21 21 21	[math]\displaystyle{ \Gamma_o^vD_2^9 }[/math]	6a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b \right ) :2:2_1 }[/math]	[math]\displaystyle{ (2_0{*}2_12_1) }[/math]
25	mm2	[math]\displaystyle{ *22 }[/math]	Pmm2	P m m 2	[math]\displaystyle{ \Gamma_oC_{2v}^1 }[/math]	13s	[math]\displaystyle{ (c:a:b):m \cdot 2 }[/math]	[math]\displaystyle{ (*{\cdot}2{\cdot}2{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [{*}_0{\cdot}{*}_0{\cdot}] }[/math]
26			Pmc21	P m c 21	[math]\displaystyle{ \Gamma_oC_{2v}^2 }[/math]	9a	[math]\displaystyle{ (c:a:b): \tilde c \cdot 2_1 }[/math]	[math]\displaystyle{ (*{\cdot}2{:}2{\cdot}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}{\cdot}\bar{*}{\cdot}) }[/math], [math]\displaystyle{ [{\times_0}{\times_0}] }[/math]
27			Pcc2	P c c 2	[math]\displaystyle{ \Gamma_oC_{2v}^3 }[/math]	5h	[math]\displaystyle{ (c:a:b): \tilde c \cdot 2 }[/math]	[math]\displaystyle{ (*{:}2{:}2{:}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}_0\bar{*}_0) }[/math]
28			Pma2	P m a 2	[math]\displaystyle{ \Gamma_oC_{2v}^4 }[/math]	6h	[math]\displaystyle{ (c:a:b): \tilde a \cdot 2 }[/math]	[math]\displaystyle{ (2_02_0{*}{\cdot}) }[/math]	[math]\displaystyle{ [{*}_0{:}{*}_0{:}] }[/math], [math]\displaystyle{ (*{\cdot}{*}_0) }[/math]
29			Pca21	P c a 21	[math]\displaystyle{ \Gamma_oC_{2v}^5 }[/math]	11a	[math]\displaystyle{ (c:a:b): \tilde a \cdot 2_1 }[/math]	[math]\displaystyle{ (2_12_1{*}{:}) }[/math]	[math]\displaystyle{ (\bar{*}{:}\bar{*}{:}) }[/math]
30			Pnc2	P n c 2	[math]\displaystyle{ \Gamma_oC_{2v}^6 }[/math]	7h	[math]\displaystyle{ (c:a:b): \tilde c \odot 2 }[/math]	[math]\displaystyle{ (2_02_0{*}{:}) }[/math]	[math]\displaystyle{ (\bar{*}_1\bar{*}_1) }[/math], [math]\displaystyle{ ({*}_0{\times}_0) }[/math]
31			Pmn21	P m n 21	[math]\displaystyle{ \Gamma_oC_{2v}^7 }[/math]	10a	[math]\displaystyle{ (c:a:b): \widetilde{ac} \cdot 2_1 }[/math]	[math]\displaystyle{ (2_12_1{*}{\cdot}) }[/math]	[math]\displaystyle{ (*{\cdot}\bar{\times}) }[/math], [math]\displaystyle{ [{\times}_0{\times}_1] }[/math]
32			Pba2	P b a 2	[math]\displaystyle{ \Gamma_oC_{2v}^8 }[/math]	9h	[math]\displaystyle{ (c:a:b): \tilde a \odot 2 }[/math]	[math]\displaystyle{ (2_02_0{\times}_0) }[/math]	[math]\displaystyle{ (*{:}{*}_0) }[/math]
33			Pna21	P n a 21	[math]\displaystyle{ \Gamma_oC_{2v}^9 }[/math]	12a	[math]\displaystyle{ (c:a:b): \tilde a \odot 2_1 }[/math]	[math]\displaystyle{ (2_12_1{\times}) }[/math]	[math]\displaystyle{ (*{:}{\times}) }[/math], [math]\displaystyle{ ({\times}{\times}_1) }[/math]
34			Pnn2	P n n 2	[math]\displaystyle{ \Gamma_oC_{2v}^{10} }[/math]	8h	[math]\displaystyle{ (c:a:b): \widetilde{ac} \odot 2 }[/math]	[math]\displaystyle{ (2_02_0{\times}_1) }[/math]	[math]\displaystyle{ (*_0{\times}_1) }[/math]
35			Cmm2	C m m 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{11} }[/math]	14s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) :m \cdot 2 }[/math]	[math]\displaystyle{ (2_0{*}{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [*_0{\cdot}{*}_0{:}] }[/math]
36			Cmc21	C m c 21	[math]\displaystyle{ \Gamma_o^bC_{2v}^{12} }[/math]	13a	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) :\tilde c \cdot 2_1 }[/math]	[math]\displaystyle{ (2_1{*}{\cdot}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}{\cdot}\bar{*}{:}) }[/math], [math]\displaystyle{ [{\times}_1{\times}_1] }[/math]
37			Ccc2	C c c 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{13} }[/math]	10h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) : \tilde c \cdot 2 }[/math]	[math]\displaystyle{ (2_0{*}{:}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}_0\bar{*}_1) }[/math]
38			Amm2	A m m 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{14} }[/math]	15s	[math]\displaystyle{ \left ( \tfrac{b+c}{2}/c:a:b\right ):m \cdot 2 }[/math]	[math]\displaystyle{ (*{\cdot}2{\cdot}2{\cdot}2{:}2) }[/math]	[math]\displaystyle{ [{*}_1{\cdot}{*}_1{\cdot}] }[/math], [math]\displaystyle{ [*{\cdot}{\times}_0] }[/math]
39			Aem2	A b m 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{15} }[/math]	11h	[math]\displaystyle{ \left ( \tfrac{b+c}{2}/c:a:b\right ) :m \cdot 2_1 }[/math]	[math]\displaystyle{ (*{\cdot}2{:}2{:}2{:}2) }[/math]	[math]\displaystyle{ [{*}_1{:}{*}_1{:}] }[/math], [math]\displaystyle{ (\bar{*}{\cdot}\bar{*}_0) }[/math]
40			Ama2	A m a 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{16} }[/math]	12h	[math]\displaystyle{ \left ( \tfrac{b+c}{2}/c:a:b\right ) : \tilde a \cdot 2 }[/math]	[math]\displaystyle{ (2_02_1{*}{\cdot}) }[/math]	[math]\displaystyle{ (*{\cdot}{*}_1) }[/math], [math]\displaystyle{ [*{:}{\times}_1] }[/math]
41			Aea2	A b a 2	[math]\displaystyle{ \Gamma_o^bC_{2v}^{17} }[/math]	13h	[math]\displaystyle{ \left ( \tfrac{b+c}{2}/c:a:b\right ) : \tilde a \cdot 2_1 }[/math]	[math]\displaystyle{ (2_02_1{*}{:}) }[/math]	[math]\displaystyle{ (*{:}{*}_1) }[/math], [math]\displaystyle{ (\bar{*}{:}\bar{*}_1) }[/math]
42			Fmm2	F m m 2	[math]\displaystyle{ \Gamma_o^fC_{2v}^{18} }[/math]	17s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:c:a:b\right ) :m \cdot 2 }[/math]	[math]\displaystyle{ (*{\cdot}2{\cdot}2{:}2{:}2) }[/math]	[math]\displaystyle{ [{*}_1{\cdot}{*}_1{:}] }[/math]
43			Fdd2	F dd2	[math]\displaystyle{ \Gamma_o^fC_{2v}^{19} }[/math]	16h	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:c:a:b \right ) : \tfrac{1}{2} \widetilde{ac} \odot 2 }[/math]	[math]\displaystyle{ (2_02_1{\times}) }[/math]	[math]\displaystyle{ ({*}_1{\times}) }[/math]
44			Imm2	I m m 2	[math]\displaystyle{ \Gamma_o^vC_{2v}^{20} }[/math]	16s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b \right ) :m \cdot 2 }[/math]	[math]\displaystyle{ (2_1{*}{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [*{\cdot}{\times}_1] }[/math]
45			Iba2	I b a 2	[math]\displaystyle{ \Gamma_o^vC_{2v}^{21} }[/math]	15h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b \right ) : \tilde c \cdot 2 }[/math]	[math]\displaystyle{ (2_1{*}{:}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}{:}\bar{*}_0) }[/math]
46			Ima2	I m a 2	[math]\displaystyle{ \Gamma_o^vC_{2v}^{22} }[/math]	14h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b \right ) : \tilde a \cdot 2 }[/math]	[math]\displaystyle{ (2_0{*}{\cdot}2{:}2) }[/math]	[math]\displaystyle{ (\bar{*}{\cdot}\bar{*}_1) }[/math], [math]\displaystyle{ [*{:}{\times}_0] }[/math]
47	[math]\displaystyle{ \tfrac{2}{m}\tfrac{2}{m}\tfrac{2}{m} }[/math]	[math]\displaystyle{ *222 }[/math]	Pmmm	P 2/m 2/m 2/m	[math]\displaystyle{ \Gamma_oD_{2h}^1 }[/math]	18s	[math]\displaystyle{ \left ( c:a:b \right ) \cdot m:2 \cdot m }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{\cdot}2] }[/math]
48			Pnnn	P 2/n 2/n 2/n	[math]\displaystyle{ \Gamma_oD_{2h}^2 }[/math]	19h	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \widetilde{ab}:2 \odot \widetilde{ac} }[/math]	[math]\displaystyle{ (2\bar{*}_12_02_0 }[/math]
49			Pccm	P 2/c 2/c 2/m	[math]\displaystyle{ \Gamma_oD_{2h}^3 }[/math]	17h	[math]\displaystyle{ \left ( c:a:b \right ) \cdot m:2 \cdot \tilde c }[/math]	[math]\displaystyle{ [*{:}2{:}2{:}2{:}2] }[/math]	[math]\displaystyle{ (*2_02_02{\cdot}2) }[/math]
50			Pban	P 2/b 2/a 2/n	[math]\displaystyle{ \Gamma_oD_{2h}^4 }[/math]	18h	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \widetilde{ab}:2 \odot \tilde a }[/math]	[math]\displaystyle{ (2\bar{*}_02_02_0) }[/math]	[math]\displaystyle{ (*2_02_02{:}2) }[/math]
51			Pmma	P 21/m 2/m 2/a	[math]\displaystyle{ \Gamma_oD_{2h}^5 }[/math]	14a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a :2 \cdot m }[/math]	[math]\displaystyle{ [2_02_0{*}{\cdot}] }[/math]	[math]\displaystyle{ [*{\cdot}2{:}2{\cdot}2{:}2] }[/math], [math]\displaystyle{ [*2{\cdot}2{\cdot}2{\cdot}2] }[/math]
52			Pnna	P 2/n 21/n 2/a	[math]\displaystyle{ \Gamma_oD_{2h}^6 }[/math]	17a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a:2 \odot \widetilde{ac} }[/math]	[math]\displaystyle{ (2_02\bar{*}_1) }[/math]	[math]\displaystyle{ (2_0{*}2{:}2) }[/math], [math]\displaystyle{ (2\bar{*}2_12_1) }[/math]
53			Pmna	P 2/m 2/n 21/a	[math]\displaystyle{ \Gamma_oD_{2h}^7 }[/math]	15a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a:2_1 \cdot \widetilde{ac} }[/math]	[math]\displaystyle{ [2_02_0{*}{:}] }[/math]	[math]\displaystyle{ (*2_12_12{\cdot}2) }[/math], [math]\displaystyle{ (2_0{*}2{\cdot}2) }[/math]
54			Pcca	P 21/c 2/c 2/a	[math]\displaystyle{ \Gamma_oD_{2h}^8 }[/math]	16a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a:2 \cdot \tilde c }[/math]	[math]\displaystyle{ (2_02\bar{*}_0) }[/math]	[math]\displaystyle{ (*2{:}2{:}2{:}2) }[/math], [math]\displaystyle{ (*2_12_12{:}2) }[/math]
55			Pbam	P 21/b 21/a 2/m	[math]\displaystyle{ \Gamma_oD_{2h}^9 }[/math]	22a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot m:2 \odot \tilde a }[/math]	[math]\displaystyle{ [2_02_0{\times}_0] }[/math]	[math]\displaystyle{ (*2{\cdot}2{:}2{\cdot}2) }[/math]
56			Pccn	P 21/c 21/c 2/n	[math]\displaystyle{ \Gamma_oD_{2h}^{10} }[/math]	27a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \widetilde{ab}:2 \cdot \tilde c }[/math]	[math]\displaystyle{ (2\bar{*}{:}2{:}2) }[/math]	[math]\displaystyle{ (2_12\bar{*}_0) }[/math]
57			Pbcm	P 2/b 21/c 21/m	[math]\displaystyle{ \Gamma_oD_{2h}^{11} }[/math]	23a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot m:2_1 \odot \tilde c }[/math]	[math]\displaystyle{ (2_02\bar{*}{\cdot}) }[/math]	[math]\displaystyle{ (*2{:}2{\cdot}2{:}2) }[/math], [math]\displaystyle{ [2_12_1{*}{:}] }[/math]
58			Pnnm	P 21/n 21/n 2/m	[math]\displaystyle{ \Gamma_oD_{2h}^{12} }[/math]	25a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot m:2 \odot \widetilde{ac} }[/math]	[math]\displaystyle{ [2_02_0{\times}_1] }[/math]	[math]\displaystyle{ (2_1{*}2{\cdot}2) }[/math]
59			Pmmn	P 21/m 21/m 2/n	[math]\displaystyle{ \Gamma_oD_{2h}^{13} }[/math]	24a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \widetilde{ab}:2 \cdot m }[/math]	[math]\displaystyle{ (2\bar{*}{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [2_12_1{*}{\cdot}] }[/math]
60			Pbcn	P 21/b 2/c 21/n	[math]\displaystyle{ \Gamma_oD_{2h}^{14} }[/math]	26a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \widetilde{ab}:2_1 \odot \tilde c }[/math]	[math]\displaystyle{ (2_02\bar{*}{:}) }[/math]	[math]\displaystyle{ (2_1{*}2{:}2) }[/math], [math]\displaystyle{ (2_12\bar{*}_1) }[/math]
61			Pbca	P 21/b 21/c 21/a	[math]\displaystyle{ \Gamma_oD_{2h}^{15} }[/math]	29a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a:2_1 \odot \tilde c }[/math]	[math]\displaystyle{ (2_12\bar{*}{:}) }[/math]
62			Pnma	P 21/n 21/m 21/a	[math]\displaystyle{ \Gamma_oD_{2h}^{16} }[/math]	28a	[math]\displaystyle{ \left ( c:a:b \right ) \cdot \tilde a:2_1 \odot m }[/math]	[math]\displaystyle{ (2_12\bar{*}{\cdot}) }[/math]	[math]\displaystyle{ (2\bar{*}{\cdot}2{:}2) }[/math], [math]\displaystyle{ [2_12_1{\times}] }[/math]
63			Cmcm	C 2/m 2/c 21/m	[math]\displaystyle{ \Gamma_o^bD_{2h}^{17} }[/math]	18a	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot m:2_1 \cdot \tilde c }[/math]	[math]\displaystyle{ [2_02_1{*}{\cdot}] }[/math]	[math]\displaystyle{ (*2{\cdot}2{\cdot}2{:}2) }[/math], [math]\displaystyle{ [2_1{*}{\cdot}2{:}2] }[/math]
64			Cmce	C 2/m 2/c 21/a	[math]\displaystyle{ \Gamma_o^bD_{2h}^{18} }[/math]	19a	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot \tilde a :2_1 \cdot \tilde c }[/math]	[math]\displaystyle{ [2_02_1{*}{:}] }[/math]	[math]\displaystyle{ (*2{\cdot}2{:}2{:}2) }[/math], [math]\displaystyle{ (*2_12{\cdot}2{:}2) }[/math]
65			Cmmm	C 2/m 2/m 2/m	[math]\displaystyle{ \Gamma_o^bD_{2h}^{19} }[/math]	19s	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot m:2 \cdot m }[/math]	[math]\displaystyle{ [2_0{*}{\cdot}2{\cdot}2] }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{:}2] }[/math]
66			Cccm	C 2/c 2/c 2/m	[math]\displaystyle{ \Gamma_o^bD_{2h}^{20} }[/math]	20h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot m:2 \cdot \tilde c }[/math]	[math]\displaystyle{ [2_0{*}{:}2{:}2] }[/math]	[math]\displaystyle{ (*2_02_12{\cdot}2) }[/math]
67			Cmme	C 2/m 2/m 2/e	[math]\displaystyle{ \Gamma_o^bD_{2h}^{21} }[/math]	21h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot \tilde a :2 \cdot m }[/math]	[math]\displaystyle{ (*2_02{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [*{\cdot}2{:}2{:}2{:}2] }[/math]
68			Ccce	C 2/c 2/c 2/e	[math]\displaystyle{ \Gamma_o^bD_{2h}^{22} }[/math]	22h	[math]\displaystyle{ \left ( \tfrac{a+b}{2}:c:a:b\right ) \cdot \tilde a :2 \cdot \tilde c }[/math]	[math]\displaystyle{ (*2_02{:}2{:}2) }[/math]	[math]\displaystyle{ (*2_02_12{:}2) }[/math]
69			Fmmm	F 2/m 2/m 2/m	[math]\displaystyle{ \Gamma_o^fD_{2h}^{23} }[/math]	21s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:c:a:b\right ) \cdot m:2 \cdot m }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{:}2{:}2] }[/math]
70			Fddd	F 2/d 2/d 2/d	[math]\displaystyle{ \Gamma_o^fD_{2h}^{24} }[/math]	24h	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:c:a:b\right ) \cdot \tfrac{1}{2}\widetilde{ab}:2 \odot \tfrac{1}{2}\widetilde{ac} }[/math]	[math]\displaystyle{ (2\bar{*}2_02_1) }[/math]
71			Immm	I 2/m 2/m 2/m	[math]\displaystyle{ \Gamma_o^vD_{2h}^{25} }[/math]	20s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b\right ) \cdot m:2 \cdot m }[/math]	[math]\displaystyle{ [2_1{*}{\cdot}2{\cdot}2] }[/math]
72			Ibam	I 2/b 2/a 2/m	[math]\displaystyle{ \Gamma_o^vD_{2h}^{26} }[/math]	23h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b\right ) \cdot m:2 \cdot \tilde c }[/math]	[math]\displaystyle{ [2_1{*}{:}2{:}2] }[/math]	[math]\displaystyle{ (*2_02{\cdot}2{:}2) }[/math]
73			Ibca	I 2/b 2/c 2/a	[math]\displaystyle{ \Gamma_o^vD_{2h}^{27} }[/math]	21a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b\right ) \cdot \tilde a :2 \cdot \tilde c }[/math]	[math]\displaystyle{ (*2_12{:}2{:}2) }[/math]
74			Imma	I 2/m 2/m 2/a	[math]\displaystyle{ \Gamma_o^vD_{2h}^{28} }[/math]	20a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:b\right ) \cdot \tilde a :2 \cdot m }[/math]	[math]\displaystyle{ (*2_12{\cdot}2{\cdot}2) }[/math]	[math]\displaystyle{ [2_0{*}{\cdot}2{:}2] }[/math]

List of tetragonal

Tetragonal Bravais lattice

Simple (P)	Body (I)

Tetragonal crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Fibrifold
75	4	[math]\displaystyle{ 44 }[/math]	P4	P 4	[math]\displaystyle{ \Gamma_qC_4^1 }[/math]	22s	[math]\displaystyle{ (c:a:a):4 }[/math]	[math]\displaystyle{ (4_04_02_0) }[/math]
76			P41	P 41	[math]\displaystyle{ \Gamma_qC_4^2 }[/math]	30a	[math]\displaystyle{ (c:a:a) :4_1 }[/math]	[math]\displaystyle{ (4_14_12_1) }[/math]
77			P42	P 42	[math]\displaystyle{ \Gamma_qC_4^3 }[/math]	33a	[math]\displaystyle{ (c:a:a) :4_2 }[/math]	[math]\displaystyle{ (4_24_22_0) }[/math]
78			P43	P 43	[math]\displaystyle{ \Gamma_qC_4^4 }[/math]	31a	[math]\displaystyle{ (c:a:a) :4_3 }[/math]	[math]\displaystyle{ (4_14_12_1) }[/math]
79			I4	I 4	[math]\displaystyle{ \Gamma_q^vC_4^5 }[/math]	23s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4 }[/math]	[math]\displaystyle{ (4_24_02_1) }[/math]
80			I41	I 41	[math]\displaystyle{ \Gamma_q^vC_4^6 }[/math]	32a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4_1 }[/math]	[math]\displaystyle{ (4_34_12_0) }[/math]
81	4	[math]\displaystyle{ 2\times }[/math]	P4	P 4	[math]\displaystyle{ \Gamma_qS_4^1 }[/math]	26s	[math]\displaystyle{ (c:a:a):\tilde 4 }[/math]	[math]\displaystyle{ (442_0) }[/math]
82			I4	I 4	[math]\displaystyle{ \Gamma_q^vS_4^2 }[/math]	27s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :\tilde 4 }[/math]	[math]\displaystyle{ (442_1) }[/math]
83	4/m	[math]\displaystyle{ 4* }[/math]	P4/m	P 4/m	[math]\displaystyle{ \Gamma_qC_{4h}^1 }[/math]	28s	[math]\displaystyle{ (c:a:a)\cdot m:4 }[/math]	[math]\displaystyle{ [4_04_02_0] }[/math]
84			P42/m	P 42/m	[math]\displaystyle{ \Gamma_qC_{4h}^2 }[/math]	41a	[math]\displaystyle{ (c:a:a)\cdot m:4_2 }[/math]	[math]\displaystyle{ [4_24_22_0] }[/math]
85			P4/n	P 4/n	[math]\displaystyle{ \Gamma_qC_{4h}^3 }[/math]	29h	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4 }[/math]	[math]\displaystyle{ (44_02) }[/math]
86			P42/n	P 42/n	[math]\displaystyle{ \Gamma_qC_{4h}^4 }[/math]	42a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4_2 }[/math]	[math]\displaystyle{ (44_22) }[/math]
87			I4/m	I 4/m	[math]\displaystyle{ \Gamma_q^vC_{4h}^5 }[/math]	29s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot m:4 }[/math]	[math]\displaystyle{ [4_24_02_1] }[/math]
88			I41/a	I 41/a	[math]\displaystyle{ \Gamma_q^vC_{4h}^6 }[/math]	40a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot \tilde a :4_1 }[/math]	[math]\displaystyle{ (44_12) }[/math]
89	422	[math]\displaystyle{ 224 }[/math]	P422	P 4 2 2	[math]\displaystyle{ \Gamma_qD_4^1 }[/math]	30s	[math]\displaystyle{ (c:a:a):4:2 }[/math]	[math]\displaystyle{ (*4_04_02_0) }[/math]
90			P4212	P4212	[math]\displaystyle{ \Gamma_qD_4^2 }[/math]	43a	[math]\displaystyle{ (c:a:a):4 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (4_0{*}2_0) }[/math]
91			P4122	P 41 2 2	[math]\displaystyle{ \Gamma_qD_4^3 }[/math]	44a	[math]\displaystyle{ (c:a:a):4_1:2 }[/math]	[math]\displaystyle{ (*4_14_12_1) }[/math]
92			P41212	P 41 21 2	[math]\displaystyle{ \Gamma_qD_4^4 }[/math]	48a	[math]\displaystyle{ (c:a:a):4_1 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (4_1{*}2_1) }[/math]
93			P4222	P 42 2 2	[math]\displaystyle{ \Gamma_qD_4^5 }[/math]	47a	[math]\displaystyle{ (c:a:a):4_2:2 }[/math]	[math]\displaystyle{ (*4_24_22_0) }[/math]
94			P42212	P 42 21 2	[math]\displaystyle{ \Gamma_qD_4^6 }[/math]	50a	[math]\displaystyle{ (c:a:a):4_2 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (4_2{*}2_0) }[/math]
95			P4322	P 43 2 2	[math]\displaystyle{ \Gamma_qD_4^7 }[/math]	45a	[math]\displaystyle{ (c:a:a):4_3:2 }[/math]	[math]\displaystyle{ (*4_14_12_1) }[/math]
96			P43212	P 43 21 2	[math]\displaystyle{ \Gamma_qD_4^8 }[/math]	49a	[math]\displaystyle{ (c:a:a):4_3 }[/math] [math]\displaystyle{ 2_1 }[/math]	[math]\displaystyle{ (4_1{*}2_1) }[/math]
97			I422	I 4 2 2	[math]\displaystyle{ \Gamma_q^vD_4^9 }[/math]	31s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4:2 }[/math]	[math]\displaystyle{ (*4_24_02_1) }[/math]
98			I4122	I 41 2 2	[math]\displaystyle{ \Gamma_q^vD_4^{10} }[/math]	46a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4:2_1 }[/math]	[math]\displaystyle{ (*4_34_12_0) }[/math]
99	4mm	[math]\displaystyle{ *44 }[/math]	P4mm	P 4 m m	[math]\displaystyle{ \Gamma_qC_{4v}^1 }[/math]	24s	[math]\displaystyle{ (c:a:a):4\cdot m }[/math]	[math]\displaystyle{ (*{\cdot}4{\cdot}4{\cdot}2) }[/math]
100			P4bm	P 4 b m	[math]\displaystyle{ \Gamma_qC_{4v}^2 }[/math]	26h	[math]\displaystyle{ (c:a:a):4\odot \tilde a }[/math]	[math]\displaystyle{ (4_0{*}{\cdot}2) }[/math]
101			P42cm	P 42 c m	[math]\displaystyle{ \Gamma_qC_{4v}^3 }[/math]	37a	[math]\displaystyle{ (c:a:a):4_2\cdot \tilde c }[/math]	[math]\displaystyle{ (*{:}4{\cdot}4{:}2) }[/math]
102			P42nm	P 42 n m	[math]\displaystyle{ \Gamma_qC_{4v}^4 }[/math]	38a	[math]\displaystyle{ (c:a:a):4_2\odot \widetilde{ac} }[/math]	[math]\displaystyle{ (4_2{*}{\cdot}2) }[/math]
103			P4cc	P 4 c c	[math]\displaystyle{ \Gamma_qC_{4v}^5 }[/math]	25h	[math]\displaystyle{ (c:a:a):4\cdot \tilde c }[/math]	[math]\displaystyle{ (*{:}4{:}4{:}2) }[/math]
104			P4nc	P 4 n c	[math]\displaystyle{ \Gamma_qC_{4v}^6 }[/math]	27h	[math]\displaystyle{ (c:a:a):4\odot \widetilde{ac} }[/math]	[math]\displaystyle{ (4_0{*}{:}2) }[/math]
105			P42mc	P 42 m c	[math]\displaystyle{ \Gamma_qC_{4v}^7 }[/math]	36a	[math]\displaystyle{ (c:a:a):4_2\cdot m }[/math]	[math]\displaystyle{ (*{\cdot}4{:}4{\cdot}2) }[/math]
106			P42bc	P 42 b c	[math]\displaystyle{ \Gamma_qC_{4v}^8 }[/math]	39a	[math]\displaystyle{ (c:a:a):4\odot \tilde a }[/math]	[math]\displaystyle{ (4_2{*}{:}2) }[/math]
107			I4mm	I 4 m m	[math]\displaystyle{ \Gamma_q^vC_{4v}^9 }[/math]	25s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4\cdot m }[/math]	[math]\displaystyle{ (*{\cdot}4{\cdot}4{:}2) }[/math]
108			I4cm	I 4 c m	[math]\displaystyle{ \Gamma_q^vC_{4v}^{10} }[/math]	28h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4\cdot \tilde c }[/math]	[math]\displaystyle{ (*{\cdot}4{:}4{:}2) }[/math]
109			I41md	I 41 m d	[math]\displaystyle{ \Gamma_q^vC_{4v}^{11} }[/math]	34a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4_1\odot m }[/math]	[math]\displaystyle{ (4_1{*}{\cdot}2) }[/math]
110			I41cd	I 41 c d	[math]\displaystyle{ \Gamma_q^vC_{4v}^{12} }[/math]	35a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :4_1\odot \tilde c }[/math]	[math]\displaystyle{ (4_1{*}{:}2) }[/math]
111	42m	[math]\displaystyle{ 2{*}2 }[/math]	P42m	P 4 2 m	[math]\displaystyle{ \Gamma_qD_{2d}^1 }[/math]	32s	[math]\displaystyle{ (c:a:a):\tilde 4 :2 }[/math]	[math]\displaystyle{ (*4{\cdot}42_0) }[/math]
112			P42c	P 4 2 c	[math]\displaystyle{ \Gamma_qD_{2d}^2 }[/math]	30h	[math]\displaystyle{ (c:a:a):\tilde 4 }[/math] [math]\displaystyle{ 2 }[/math]	[math]\displaystyle{ (*4{:}42_0) }[/math]
113			P421m	P 4 21 m	[math]\displaystyle{ \Gamma_qD_{2d}^3 }[/math]	52a	[math]\displaystyle{ (c:a:a):\tilde 4 \cdot \widetilde{ab} }[/math]	[math]\displaystyle{ (4\bar{*}{\cdot}2) }[/math]
114			P421c	P 4 21 c	[math]\displaystyle{ \Gamma_qD_{2d}^4 }[/math]	53a	[math]\displaystyle{ (c:a:a):\tilde 4 \cdot \widetilde{abc} }[/math]	[math]\displaystyle{ (4\bar{*}{:}2) }[/math]
115			P4m2	P 4 m 2	[math]\displaystyle{ \Gamma_qD_{2d}^5 }[/math]	33s	[math]\displaystyle{ (c:a:a):\tilde 4 \cdot m }[/math]	[math]\displaystyle{ (*{\cdot}44{\cdot}2) }[/math]
116			P4c2	P 4 c 2	[math]\displaystyle{ \Gamma_qD_{2d}^6 }[/math]	31h	[math]\displaystyle{ (c:a:a):\tilde 4 \cdot \tilde c }[/math]	[math]\displaystyle{ (*{:}44{:}2) }[/math]
117			P4b2	P 4 b 2	[math]\displaystyle{ \Gamma_qD_{2d}^7 }[/math]	32h	[math]\displaystyle{ (c:a:a):\tilde 4 \odot \tilde a }[/math]	[math]\displaystyle{ (4\bar{*}_02_0) }[/math]
118			P4n2	P 4 n 2	[math]\displaystyle{ \Gamma_qD_{2d}^8 }[/math]	33h	[math]\displaystyle{ (c:a:a):\tilde 4 \cdot \widetilde{ac} }[/math]	[math]\displaystyle{ (4\bar{*}_12_0) }[/math]
119			I4m2	I 4 m 2	[math]\displaystyle{ \Gamma_q^vD_{2d}^9 }[/math]	35s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :\tilde 4 \cdot m }[/math]	[math]\displaystyle{ (*4{\cdot}42_1) }[/math]
120			I4c2	I 4 c 2	[math]\displaystyle{ \Gamma_q^vD_{2d}^{10} }[/math]	34h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :\tilde 4 \cdot \tilde c }[/math]	[math]\displaystyle{ (*4{:}42_1) }[/math]
121			I42m	I 4 2 m	[math]\displaystyle{ \Gamma_q^vD_{2d}^{11} }[/math]	34s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :\tilde 4 :2 }[/math]	[math]\displaystyle{ (*{\cdot}44{:}2) }[/math]
122			I42d	I 4 2 d	[math]\displaystyle{ \Gamma_q^vD_{2d}^{12} }[/math]	51a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) :\tilde 4 \odot \tfrac{1}{2}\widetilde{abc} }[/math]	[math]\displaystyle{ (4\bar{*}2_1) }[/math]
123	4/m 2/m 2/m	[math]\displaystyle{ *224 }[/math]	P4/mmm	P 4/m 2/m 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^1 }[/math]	36s	[math]\displaystyle{ (c:a:a)\cdot m:4\cdot m }[/math]	[math]\displaystyle{ [*{\cdot}4{\cdot}4{\cdot}2] }[/math]
124			P4/mcc	P 4/m 2/c 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^2 }[/math]	35h	[math]\displaystyle{ (c:a:a)\cdot m:4\cdot \tilde c }[/math]	[math]\displaystyle{ [*{:}4{:}4{:}2] }[/math]
125			P4/nbm	P 4/n 2/b 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^3 }[/math]	36h	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4\odot \tilde a }[/math]	[math]\displaystyle{ (*4_04{\cdot}2) }[/math]
126			P4/nnc	P 4/n 2/n 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^4 }[/math]	37h	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4\odot \widetilde{ac} }[/math]	[math]\displaystyle{ (*4_04{:}2) }[/math]
127			P4/mbm	P 4/m 21/b 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^5 }[/math]	54a	[math]\displaystyle{ (c:a:a)\cdot m:4\odot \tilde a }[/math]	[math]\displaystyle{ [4_0{*}{\cdot}2] }[/math]
128			P4/mnc	P 4/m 21/n 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^6 }[/math]	56a	[math]\displaystyle{ (c:a:a)\cdot m:4\odot \widetilde{ac} }[/math]	[math]\displaystyle{ [4_0{*}{:}2] }[/math]
129			P4/nmm	P 4/n 21/m 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^7 }[/math]	55a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4\cdot m }[/math]	[math]\displaystyle{ (*4{\cdot}4{\cdot}2) }[/math]
130			P4/ncc	P 4/n 21/c 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^8 }[/math]	57a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4\cdot \tilde c }[/math]	[math]\displaystyle{ (*4{:}4{:}2) }[/math]
131			P42/mmc	P 42/m 2/m 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^9 }[/math]	60a	[math]\displaystyle{ (c:a:a)\cdot m:4_2\cdot m }[/math]	[math]\displaystyle{ [*{\cdot}4{:}4{\cdot}2] }[/math]
132			P42/mcm	P 42/m 2/c 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^{10} }[/math]	61a	[math]\displaystyle{ (c:a:a)\cdot m:4_2\cdot \tilde c }[/math]	[math]\displaystyle{ [*{:}4{\cdot}4{:}2] }[/math]
133			P42/nbc	P 42/n 2/b 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^{11} }[/math]	63a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4_2\odot \tilde a }[/math]	[math]\displaystyle{ (*4_24{:}2) }[/math]
134			P42/nnm	P 42/n 2/n 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^{12} }[/math]	62a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4_2\odot \widetilde{ac} }[/math]	[math]\displaystyle{ (*4_24{\cdot}2) }[/math]
135			P42/mbc	P 42/m 21/b 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^{13} }[/math]	66a	[math]\displaystyle{ (c:a:a)\cdot m:4_2\odot \tilde a }[/math]	[math]\displaystyle{ [4_2{*}{:}2] }[/math]
136			P42/mnm	P 42/m 21/n 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^{14} }[/math]	65a	[math]\displaystyle{ (c:a:a)\cdot m:4_2\odot \widetilde{ac} }[/math]	[math]\displaystyle{ [4_2{*}{\cdot}2] }[/math]
137			P42/nmc	P 42/n 21/m 2/c	[math]\displaystyle{ \Gamma_qD_{4h}^{15} }[/math]	67a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4_2\cdot m }[/math]	[math]\displaystyle{ (*4{\cdot}4{:}2) }[/math]
138			P42/ncm	P 42/n 21/c 2/m	[math]\displaystyle{ \Gamma_qD_{4h}^{16} }[/math]	65a	[math]\displaystyle{ (c:a:a)\cdot \widetilde{ab}:4_2\cdot \tilde c }[/math]	[math]\displaystyle{ (*4{:}4{\cdot}2) }[/math]
139			I4/mmm	I 4/m 2/m 2/m	[math]\displaystyle{ \Gamma_q^vD_{4h}^{17} }[/math]	37s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot m:4\cdot m }[/math]	[math]\displaystyle{ [*{\cdot}4{\cdot}4{:}2] }[/math]
140			I4/mcm	I 4/m 2/c 2/m	[math]\displaystyle{ \Gamma_q^vD_{4h}^{18} }[/math]	38h	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot m:4\cdot \tilde c }[/math]	[math]\displaystyle{ [*{\cdot}4{:}4{:}2] }[/math]
141			I41/amd	I 41/a 2/m 2/d	[math]\displaystyle{ \Gamma_q^vD_{4h}^{19} }[/math]	59a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot \tilde a :4_1\odot m }[/math]	[math]\displaystyle{ (*4_14{\cdot}2) }[/math]
142			I41/acd	I 41/a 2/c 2/d	[math]\displaystyle{ \Gamma_q^vD_{4h}^{20} }[/math]	58a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/c:a:a\right ) \cdot \tilde a :4_1\odot \tilde c }[/math]	[math]\displaystyle{ (*4_14{:}2) }[/math]

List of trigonal

Trigonal Bravais lattice

Rhombohedral (R)	Hexagonal (P)

Trigonal crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Fibrifold
143	3	[math]\displaystyle{ 33 }[/math]	P3	P 3	[math]\displaystyle{ \Gamma_hC_3^1 }[/math]	38s	[math]\displaystyle{ (c:(a/a)):3 }[/math]	[math]\displaystyle{ (3_03_03_0) }[/math]
144			P31	P 31	[math]\displaystyle{ \Gamma_hC_3^2 }[/math]	68a	[math]\displaystyle{ (c:(a/a)):3_1 }[/math]	[math]\displaystyle{ (3_13_13_1) }[/math]
145			P32	P 32	[math]\displaystyle{ \Gamma_hC_3^3 }[/math]	69a	[math]\displaystyle{ (c:(a/a)):3_2 }[/math]	[math]\displaystyle{ (3_13_13_1) }[/math]
146			R3	R 3	[math]\displaystyle{ \Gamma_{rh}C_3^4 }[/math]	39s	[math]\displaystyle{ (a/a/a)/3 }[/math]	[math]\displaystyle{ (3_03_13_2) }[/math]
147	3	[math]\displaystyle{ 3\times }[/math]	P3	P 3	[math]\displaystyle{ \Gamma_hC_{3i}^1 }[/math]	51s	[math]\displaystyle{ (c:(a/a)):\tilde 6 }[/math]	[math]\displaystyle{ (63_02) }[/math]
148			R3	R 3	[math]\displaystyle{ \Gamma_{rh}C_{3i}^2 }[/math]	52s	[math]\displaystyle{ (a/a/a)/\tilde 6 }[/math]	[math]\displaystyle{ (63_12) }[/math]
149	32	[math]\displaystyle{ 223 }[/math]	P312	P 3 1 2	[math]\displaystyle{ \Gamma_hD_3^1 }[/math]	45s	[math]\displaystyle{ (c:(a/a)):2:3 }[/math]	[math]\displaystyle{ (*3_03_03_0) }[/math]
150			P321	P 3 2 1	[math]\displaystyle{ \Gamma_hD_3^2 }[/math]	44s	[math]\displaystyle{ (c:(a/a))\cdot 2:3 }[/math]	[math]\displaystyle{ (3_0{*}3_0) }[/math]
151			P3112	P 31 1 2	[math]\displaystyle{ \Gamma_hD_3^3 }[/math]	72a	[math]\displaystyle{ (c:(a/a)):2:3_1 }[/math]	[math]\displaystyle{ (*3_13_13_1) }[/math]
152			P3121	P 31 2 1	[math]\displaystyle{ \Gamma_hD_3^4 }[/math]	70a	[math]\displaystyle{ (c:(a/a))\cdot 2:3_1 }[/math]	[math]\displaystyle{ (3_1{*}3_1) }[/math]
153			P3212	P 32 1 2	[math]\displaystyle{ \Gamma_hD_3^5 }[/math]	73a	[math]\displaystyle{ (c:(a/a)):2:3_2 }[/math]	[math]\displaystyle{ (*3_13_13_1) }[/math]
154			P3221	P 32 2 1	[math]\displaystyle{ \Gamma_hD_3^6 }[/math]	71a	[math]\displaystyle{ (c:(a/a))\cdot 2:3_2 }[/math]	[math]\displaystyle{ (3_1{*}3_1) }[/math]
155			R32	R 3 2	[math]\displaystyle{ \Gamma_{rh}D_3^7 }[/math]	46s	[math]\displaystyle{ (a/a/a)/3:2 }[/math]	[math]\displaystyle{ (*3_03_13_2) }[/math]
156	3m	[math]\displaystyle{ *33 }[/math]	P3m1	P 3 m 1	[math]\displaystyle{ \Gamma_hC_{3v}^1 }[/math]	40s	[math]\displaystyle{ (c:(a/a)):m\cdot 3 }[/math]	[math]\displaystyle{ (*{\cdot}3{\cdot}3{\cdot}3) }[/math]
157			P31m	P 3 1 m	[math]\displaystyle{ \Gamma_hC_{3v}^2 }[/math]	41s	[math]\displaystyle{ (c:(a/a))\cdot m\cdot 3 }[/math]	[math]\displaystyle{ (3_0{*}{\cdot}3) }[/math]
158			P3c1	P 3 c 1	[math]\displaystyle{ \Gamma_hC_{3v}^3 }[/math]	39h	[math]\displaystyle{ (c:(a/a)):\tilde c:3 }[/math]	[math]\displaystyle{ (*{:}3{:}3{:}3) }[/math]
159			P31c	P 3 1 c	[math]\displaystyle{ \Gamma_hC_{3v}^4 }[/math]	40h	[math]\displaystyle{ (c:(a/a))\cdot\tilde c :3 }[/math]	[math]\displaystyle{ (3_0{*}{:}3) }[/math]
160			R3m	R 3 m	[math]\displaystyle{ \Gamma_{rh}C_{3v}^5 }[/math]	42s	[math]\displaystyle{ (a/a/a)/3\cdot m }[/math]	[math]\displaystyle{ (3_1{*}{\cdot}3) }[/math]
161			R3c	R 3 c	[math]\displaystyle{ \Gamma_{rh}C_{3v}^6 }[/math]	41h	[math]\displaystyle{ (a/a/a)/3\cdot\tilde c }[/math]	[math]\displaystyle{ (3_1{*}{:}3) }[/math]
162	3 2/m	[math]\displaystyle{ 2{*}3 }[/math]	P31m	P 3 1 2/m	[math]\displaystyle{ \Gamma_hD_{3d}^1 }[/math]	56s	[math]\displaystyle{ (c:(a/a))\cdot m\cdot\tilde 6 }[/math]	[math]\displaystyle{ (*{\cdot}63_02) }[/math]
163			P31c	P 3 1 2/c	[math]\displaystyle{ \Gamma_hD_{3d}^2 }[/math]	46h	[math]\displaystyle{ (c:(a/a))\cdot\tilde c \cdot\tilde 6 }[/math]	[math]\displaystyle{ (*{:}63_02) }[/math]
164			P3m1	P 3 2/m 1	[math]\displaystyle{ \Gamma_hD_{3d}^3 }[/math]	55s	[math]\displaystyle{ (c:(a/a)):m\cdot\tilde 6 }[/math]	[math]\displaystyle{ (*6{\cdot}3{\cdot}2) }[/math]
165			P3c1	P 3 2/c 1	[math]\displaystyle{ \Gamma_hD_{3d}^4 }[/math]	45h	[math]\displaystyle{ (c:(a/a)):\tilde c \cdot\tilde 6 }[/math]	[math]\displaystyle{ (*6{:}3{:}2) }[/math]
166			R3m	R 3 2/m	[math]\displaystyle{ \Gamma_{rh}D_{3d}^5 }[/math]	57s	[math]\displaystyle{ (a/a/a)/\tilde 6 \cdot m }[/math]	[math]\displaystyle{ (*{\cdot}63_12) }[/math]
167			R3c	R 3 2/c	[math]\displaystyle{ \Gamma_{rh}D_{3d}^6 }[/math]	47h	[math]\displaystyle{ (a/a/a)/\tilde 6 \cdot\tilde c }[/math]	[math]\displaystyle{ (*{:}63_12) }[/math]

List of hexagonal

Hexagonal Bravais lattice

Hexagonal crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Fibrifold
168	6	[math]\displaystyle{ 66 }[/math]	P6	P 6	[math]\displaystyle{ \Gamma_hC_6^1 }[/math]	49s	[math]\displaystyle{ (c:(a/a)):6 }[/math]	[math]\displaystyle{ (6_03_02_0) }[/math]
169			P61	P 61	[math]\displaystyle{ \Gamma_hC_6^2 }[/math]	74a	[math]\displaystyle{ (c:(a/a)):6_1 }[/math]	[math]\displaystyle{ (6_13_12_1) }[/math]
170			P65	P 65	[math]\displaystyle{ \Gamma_hC_6^3 }[/math]	75a	[math]\displaystyle{ (c:(a/a)):6_5 }[/math]	[math]\displaystyle{ (6_13_12_1) }[/math]
171			P62	P 62	[math]\displaystyle{ \Gamma_hC_6^4 }[/math]	76a	[math]\displaystyle{ (c:(a/a)):6_2 }[/math]	[math]\displaystyle{ (6_23_22_0) }[/math]
172			P64	P 64	[math]\displaystyle{ \Gamma_hC_6^5 }[/math]	77a	[math]\displaystyle{ (c:(a/a)):6_4 }[/math]	[math]\displaystyle{ (6_23_22_0) }[/math]
173			P63	P 63	[math]\displaystyle{ \Gamma_hC_6^6 }[/math]	78a	[math]\displaystyle{ (c:(a/a)):6_3 }[/math]	[math]\displaystyle{ (6_33_02_1) }[/math]
174	6	[math]\displaystyle{ 3* }[/math]	P6	P 6	[math]\displaystyle{ \Gamma_hC_{3h}^1 }[/math]	43s	[math]\displaystyle{ (c:(a/a)):3:m }[/math]	[math]\displaystyle{ [3_03_03_0] }[/math]
175	6/m	[math]\displaystyle{ 6* }[/math]	P6/m	P 6/m	[math]\displaystyle{ \Gamma_hC_{6h}^1 }[/math]	53s	[math]\displaystyle{ (c:(a/a))\cdot m :6 }[/math]	[math]\displaystyle{ [6_03_02_0] }[/math]
176			P63/m	P 63/m	[math]\displaystyle{ \Gamma_hC_{6h}^2 }[/math]	81a	[math]\displaystyle{ (c:(a/a))\cdot m :6_3 }[/math]	[math]\displaystyle{ [6_33_02_1] }[/math]
177	622	[math]\displaystyle{ 226 }[/math]	P622	P 6 2 2	[math]\displaystyle{ \Gamma_hD_6^1 }[/math]	54s	[math]\displaystyle{ (c:(a/a))\cdot 2 :6 }[/math]	[math]\displaystyle{ (*6_03_02_0) }[/math]
178			P6122	P 61 2 2	[math]\displaystyle{ \Gamma_hD_6^2 }[/math]	82a	[math]\displaystyle{ (c:(a/a))\cdot 2 :6_1 }[/math]	[math]\displaystyle{ (*6_13_12_1) }[/math]
179			P6522	P 65 2 2	[math]\displaystyle{ \Gamma_hD_6^3 }[/math]	83a	[math]\displaystyle{ (c:(a/a))\cdot 2 :6_5 }[/math]	[math]\displaystyle{ (*6_13_12_1) }[/math]
180			P6222	P 62 2 2	[math]\displaystyle{ \Gamma_hD_6^4 }[/math]	84a	[math]\displaystyle{ (c:(a/a))\cdot 2 :6_2 }[/math]	[math]\displaystyle{ (*6_23_22_0) }[/math]
181			P6422	P 64 2 2	[math]\displaystyle{ \Gamma_hD_6^5 }[/math]	85a	[math]\displaystyle{ (c:(a/a))\cdot 2 :6_4 }[/math]	[math]\displaystyle{ (*6_23_22_0) }[/math]
182			P6322	P 63 2 2	[math]\displaystyle{ \Gamma_hD_6^6 }[/math]	86a	[math]\displaystyle{ (c:(a/a))\cdot 2 :6_3 }[/math]	[math]\displaystyle{ (*6_33_02_1) }[/math]
183	6mm	[math]\displaystyle{ *66 }[/math]	P6mm	P 6 m m	[math]\displaystyle{ \Gamma_hC_{6v}^1 }[/math]	50s	[math]\displaystyle{ (c:(a/a)):m\cdot 6 }[/math]	[math]\displaystyle{ (*{\cdot}6{\cdot}3{\cdot}2) }[/math]
184			P6cc	P 6 c c	[math]\displaystyle{ \Gamma_hC_{6v}^2 }[/math]	44h	[math]\displaystyle{ (c:(a/a)):\tilde c \cdot 6 }[/math]	[math]\displaystyle{ (*{:}6{:}3{:}2) }[/math]
185			P63cm	P 63 c m	[math]\displaystyle{ \Gamma_hC_{6v}^3 }[/math]	80a	[math]\displaystyle{ (c:(a/a)):\tilde c \cdot 6_3 }[/math]	[math]\displaystyle{ (*{\cdot}6{:}3{:}2) }[/math]
186			P63mc	P 63 m c	[math]\displaystyle{ \Gamma_hC_{6v}^4 }[/math]	79a	[math]\displaystyle{ (c:(a/a)):m\cdot 6_3 }[/math]	[math]\displaystyle{ (*{:}6{\cdot}3{\cdot}2) }[/math]
187	6m2	[math]\displaystyle{ *223 }[/math]	P6m2	P 6 m 2	[math]\displaystyle{ \Gamma_hD_{3h}^1 }[/math]	48s	[math]\displaystyle{ (c:(a/a)):m\cdot 3:m }[/math]	[math]\displaystyle{ [*{\cdot}3{\cdot}3{\cdot}3] }[/math]
188			P6c2	P 6 c 2	[math]\displaystyle{ \Gamma_hD_{3h}^2 }[/math]	43h	[math]\displaystyle{ (c:(a/a)):\tilde c \cdot 3:m }[/math]	[math]\displaystyle{ [*{:}3{:}3{:}3] }[/math]
189			P62m	P 6 2 m	[math]\displaystyle{ \Gamma_hD_{3h}^3 }[/math]	47s	[math]\displaystyle{ (c:(a/a))\cdot m:3\cdot m }[/math]	[math]\displaystyle{ [3_0{*}{\cdot}3] }[/math]
190			P62c	P 6 2 c	[math]\displaystyle{ \Gamma_hD_{3h}^4 }[/math]	42h	[math]\displaystyle{ (c:(a/a))\cdot m:3\cdot \tilde c }[/math]	[math]\displaystyle{ [3_0{*}{:}3] }[/math]
191	6/m 2/m 2/m	[math]\displaystyle{ *226 }[/math]	P6/mmm	P 6/m 2/m 2/m	[math]\displaystyle{ \Gamma_hD_{6h}^1 }[/math]	58s	[math]\displaystyle{ (c:(a/a))\cdot m:6\cdot m }[/math]	[math]\displaystyle{ [*{\cdot}6{\cdot}3{\cdot}2] }[/math]
192			P6/mcc	P 6/m 2/c 2/c	[math]\displaystyle{ \Gamma_hD_{6h}^2 }[/math]	48h	[math]\displaystyle{ (c:(a/a))\cdot m:6\cdot\tilde c }[/math]	[math]\displaystyle{ [*{:}6{:}3{:}2] }[/math]
193			P63/mcm	P 63/m 2/c 2/m	[math]\displaystyle{ \Gamma_hD_{6h}^3 }[/math]	87a	[math]\displaystyle{ (c:(a/a))\cdot m:6_3\cdot\tilde c }[/math]	[math]\displaystyle{ [*{\cdot}6{:}3{:}2] }[/math]
194			P63/mmc	P 63/m 2/m 2/c	[math]\displaystyle{ \Gamma_hD_{6h}^4 }[/math]	88a	[math]\displaystyle{ (c:(a/a))\cdot m:6_3\cdot m }[/math]	[math]\displaystyle{ [*{:}6{\cdot}3{\cdot}2] }[/math]

List of cubic

Cubic Bravais lattice

Simple (P)	Body centered (I)	Face centered (F)

Example cubic structures

• 

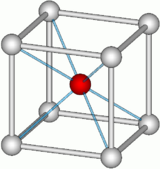
  (221) Caesium chloride. Different colors for the two atom types.

• 

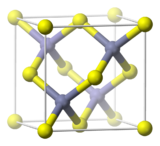
  (216) Sphalerite

• 

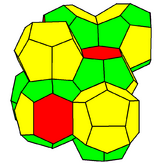
  (223) Weaire–Phelan structure

Cubic crystal system

Number	Point group	Orbifold	Short name	Full name	Schoenflies	Fedorov	Shubnikov	Conway	Fibrifold (preserving [math]\displaystyle{ z }[/math])	Fibrifold (preserving [math]\displaystyle{ x }[/math], [math]\displaystyle{ y }[/math], [math]\displaystyle{ z }[/math])
195	23	[math]\displaystyle{ 332 }[/math]	P23	P 2 3	[math]\displaystyle{ \Gamma_cT^1 }[/math]	59s	[math]\displaystyle{ \left ( a:a:a\right ) :2/3 }[/math]	[math]\displaystyle{ 2^\circ }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}3 }[/math]
196			F23	F 2 3	[math]\displaystyle{ \Gamma_c^fT^2 }[/math]	61s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :2/3 }[/math]	[math]\displaystyle{ 1^\circ }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}3 }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}3 }[/math]
197			I23	I 2 3	[math]\displaystyle{ \Gamma_c^vT^3 }[/math]	60s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :2/3 }[/math]	[math]\displaystyle{ 4^{\circ\circ} }[/math]	[math]\displaystyle{ (2_1{*}2_02_0){:}3 }[/math]	[math]\displaystyle{ (2_1{*}2_02_0){:}3 }[/math]
198			P213	P 21 3	[math]\displaystyle{ \Gamma_cT^4 }[/math]	89a	[math]\displaystyle{ \left ( a:a:a\right ) :2_1/3 }[/math]	[math]\displaystyle{ 1^\circ/4 }[/math]	[math]\displaystyle{ (2_12_1\bar{\times}){:}3 }[/math]	[math]\displaystyle{ (2_12_1\bar{\times}){:}3 }[/math]
199			I213	I 21 3	[math]\displaystyle{ \Gamma_c^vT^5 }[/math]	90a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :2_1/3 }[/math]	[math]\displaystyle{ 2^\circ/4 }[/math]	[math]\displaystyle{ (2_0{*}2_12_1){:}3 }[/math]	[math]\displaystyle{ (2_0{*}2_12_1){:}3 }[/math]
200	2/m 3	[math]\displaystyle{ 3{*}2 }[/math]	Pm3	P 2/m 3	[math]\displaystyle{ \Gamma_cT_h^1 }[/math]	62s	[math]\displaystyle{ \left ( a:a:a\right ) \cdot m/ \tilde 6 }[/math]	[math]\displaystyle{ 4^- }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{\cdot}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{\cdot}2]{:}3 }[/math]
201			Pn3	P 2/n 3	[math]\displaystyle{ \Gamma_cT_h^2 }[/math]	49h	[math]\displaystyle{ \left ( a:a:a\right ) \cdot \widetilde{ab} / \tilde 6 }[/math]	[math]\displaystyle{ 4^{\circ+} }[/math]	[math]\displaystyle{ (2\bar{*}_12_02_0){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}_12_02_0){:}3 }[/math]
202			Fm3	F 2/m 3	[math]\displaystyle{ \Gamma_c^fT_h^3 }[/math]	64s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) \cdot m/ \tilde 6 }[/math]	[math]\displaystyle{ 2^- }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{:}2{:}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{:}2{:}2]{:}3 }[/math]
203			Fd3	F 2/d 3	[math]\displaystyle{ \Gamma_c^fT_h^4 }[/math]	50h	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) \cdot \tfrac{1}{2}\widetilde{ab} / \tilde 6 }[/math]	[math]\displaystyle{ 2^{\circ+} }[/math]	[math]\displaystyle{ (2\bar{*}2_02_1){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}2_02_1){:}3 }[/math]
204			Im3	I 2/m 3	[math]\displaystyle{ \Gamma_c^vT_h^5 }[/math]	63s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) \cdot m/\tilde 6 }[/math]	[math]\displaystyle{ 8^{-\circ} }[/math]	[math]\displaystyle{ [2_1{*}{\cdot}2{\cdot}2]{:}3 }[/math]	[math]\displaystyle{ [2_1{*}{\cdot}2{\cdot}2]{:}3 }[/math]
205			Pa3	P 21/a 3	[math]\displaystyle{ \Gamma_cT_h^6 }[/math]	91a	[math]\displaystyle{ \left ( a:a:a\right ) \cdot \tilde a /\tilde 6 }[/math]	[math]\displaystyle{ 2^-/4 }[/math]	[math]\displaystyle{ (2_12\bar{*}{:}){:}3) }[/math]	[math]\displaystyle{ (2_12\bar{*}{:}){:}3) }[/math]
206			Ia3	I 21/a 3	[math]\displaystyle{ \Gamma_c^vT_h^7 }[/math]	92a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) \cdot \tilde a /\tilde 6 }[/math]	[math]\displaystyle{ 4^-/4 }[/math]	[math]\displaystyle{ (*2_12{:}2{:}2){:}3 }[/math]	[math]\displaystyle{ (*2_12{:}2{:}2){:}3 }[/math]
207	432	[math]\displaystyle{ 432 }[/math]	P432	P 4 3 2	[math]\displaystyle{ \Gamma_cO^1 }[/math]	68s	[math]\displaystyle{ \left ( a:a:a\right ) :4/3 }[/math]	[math]\displaystyle{ 4^{\circ-} }[/math]	[math]\displaystyle{ (*4_04_02_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}6 }[/math]
208			P4232	P 42 3 2	[math]\displaystyle{ \Gamma_cO^2 }[/math]	98a	[math]\displaystyle{ \left ( a:a:a\right ) :4_2//3 }[/math]	[math]\displaystyle{ 4^+ }[/math]	[math]\displaystyle{ (*4_24_22_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}6 }[/math]
209			F432	F 4 3 2	[math]\displaystyle{ \Gamma_c^fO^3 }[/math]	70s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4/3 }[/math]	[math]\displaystyle{ 2^{\circ-} }[/math]	[math]\displaystyle{ (*4_24_02_1){:}3 }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}6 }[/math]
210			F4132	F 41 3 2	[math]\displaystyle{ \Gamma_c^fO^4 }[/math]	97a	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4_1//3 }[/math]	[math]\displaystyle{ 2^+ }[/math]	[math]\displaystyle{ (*4_34_12_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}6 }[/math]
211			I432	I 4 3 2	[math]\displaystyle{ \Gamma_c^vO^5 }[/math]	69s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :4/3 }[/math]	[math]\displaystyle{ 8^{+\circ} }[/math]	[math]\displaystyle{ (4_24_02_1){:3} }[/math]	[math]\displaystyle{ (2_1{*}2_02_0){:}6 }[/math]
212			P4332	P 43 3 2	[math]\displaystyle{ \Gamma_cO^6 }[/math]	94a	[math]\displaystyle{ \left ( a:a:a\right ) :4_3//3 }[/math]	[math]\displaystyle{ 2^+/4 }[/math]	[math]\displaystyle{ (4_1{*}2_1){:}3 }[/math]	[math]\displaystyle{ (2_12_1\bar{\times}){:}6 }[/math]
213			P4132	P 41 3 2	[math]\displaystyle{ \Gamma_cO^7 }[/math]	95a	[math]\displaystyle{ \left ( a:a:a\right ) :4_1//3 }[/math]	[math]\displaystyle{ 2^+/4 }[/math]	[math]\displaystyle{ (4_1{*}2_1){:}3 }[/math]	[math]\displaystyle{ (2_12_1\bar{\times}){:}6 }[/math]
214			I4132	I 41 3 2	[math]\displaystyle{ \Gamma_c^vO^8 }[/math]	96a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/:a:a:a\right ) :4_1//3 }[/math]	[math]\displaystyle{ 4^+/4 }[/math]	[math]\displaystyle{ (*4_34_12_0){:}3 }[/math]	[math]\displaystyle{ (2_0{*}2_12_1){:}6 }[/math]
215	43m	[math]\displaystyle{ *332 }[/math]	P43m	P 4 3 m	[math]\displaystyle{ \Gamma_cT_d^1 }[/math]	65s	[math]\displaystyle{ \left ( a:a:a\right ) :\tilde 4 /3 }[/math]	[math]\displaystyle{ 2^\circ{:}2 }[/math]	[math]\displaystyle{ (*4{\cdot}42_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}6 }[/math]
216			F43m	F 4 3 m	[math]\displaystyle{ \Gamma_c^fT_d^2 }[/math]	67s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :\tilde 4 /3 }[/math]	[math]\displaystyle{ 1^\circ{:}2 }[/math]	[math]\displaystyle{ (*4{\cdot}42_1){:}3 }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}6 }[/math]
217			I43m	I 4 3 m	[math]\displaystyle{ \Gamma_c^vT_d^3 }[/math]	66s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :\tilde 4 /3 }[/math]	[math]\displaystyle{ 4^\circ{:}2 }[/math]	[math]\displaystyle{ (*{\cdot}44{:}2){:}3 }[/math]	[math]\displaystyle{ (2_1{*}2_02_0){:}6 }[/math]
218			P43n	P 4 3 n	[math]\displaystyle{ \Gamma_cT_d^4 }[/math]	51h	[math]\displaystyle{ \left ( a:a:a\right ) :\tilde 4 //3 }[/math]	[math]\displaystyle{ 4^\circ }[/math]	[math]\displaystyle{ (*4{:}42_0){:}3 }[/math]	[math]\displaystyle{ (*2_02_02_02_0){:}6 }[/math]
219			F43c	F 4 3 c	[math]\displaystyle{ \Gamma_c^fT_d^5 }[/math]	52h	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :\tilde 4 //3 }[/math]	[math]\displaystyle{ 2^{\circ\circ} }[/math]	[math]\displaystyle{ (*4{:}42_1){:}3 }[/math]	[math]\displaystyle{ (*2_02_12_02_1){:}6 }[/math]
220			I43d	I 4 3 d	[math]\displaystyle{ \Gamma_c^vT_d^6 }[/math]	93a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :\tilde 4 //3 }[/math]	[math]\displaystyle{ 4^\circ/4 }[/math]	[math]\displaystyle{ (4\bar{*}2_1){:}3 }[/math]	[math]\displaystyle{ (2_0{*}2_12_1){:}6 }[/math]
221	4/m 3 2/m	[math]\displaystyle{ *432 }[/math]	Pm3m	P 4/m 3 2/m	[math]\displaystyle{ \Gamma_cO_h^1 }[/math]	71s	[math]\displaystyle{ \left ( a:a:a\right ) :4/\tilde 6 \cdot m }[/math]	[math]\displaystyle{ 4^-{:}2 }[/math]	[math]\displaystyle{ [*{\cdot}4{\cdot}4{\cdot}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{\cdot}2]{:}6 }[/math]
222			Pn3n	P 4/n 3 2/n	[math]\displaystyle{ \Gamma_cO_h^2 }[/math]	53h	[math]\displaystyle{ \left ( a:a:a\right ) :4/\tilde 6 \cdot \widetilde{abc} }[/math]	[math]\displaystyle{ 8^{\circ\circ} }[/math]	[math]\displaystyle{ (*4_04{:}2){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}_12_02_0){:}6 }[/math]
223			Pm3n	P 42/m 3 2/n	[math]\displaystyle{ \Gamma_cO_h^3 }[/math]	102a	[math]\displaystyle{ \left ( a:a:a\right ) :4_2//\tilde 6 \cdot \widetilde{abc} }[/math]	[math]\displaystyle{ 8^\circ }[/math]	[math]\displaystyle{ [*{\cdot}4{:}4{\cdot}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{\cdot}2{\cdot}2]{:}6 }[/math]
224			Pn3m	P 42/n 3 2/m	[math]\displaystyle{ \Gamma_cO_h^4 }[/math]	103a	[math]\displaystyle{ \left ( a:a:a\right ) :4_2//\tilde 6 \cdot m }[/math]	[math]\displaystyle{ 4^+{:}2 }[/math]	[math]\displaystyle{ (*4_24{\cdot}2){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}_12_02_0){:}6 }[/math]
225			Fm3m	F 4/m 3 2/m	[math]\displaystyle{ \Gamma_c^fO_h^5 }[/math]	73s	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4/\tilde 6 \cdot m }[/math]	[math]\displaystyle{ 2^-{:}2 }[/math]	[math]\displaystyle{ [*{\cdot}4{\cdot}4{:}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{:}2{:}2]{:}6 }[/math]
226			Fm3c	F 4/m 3 2/c	[math]\displaystyle{ \Gamma_c^fO_h^6 }[/math]	54h	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4/\tilde 6 \cdot \tilde c }[/math]	[math]\displaystyle{ 4^{--} }[/math]	[math]\displaystyle{ [*{\cdot}4{:}4{:}2]{:}3 }[/math]	[math]\displaystyle{ [*{\cdot}2{\cdot}2{:}2{:}2]{:}6 }[/math]
227			Fd3m	F 41/d 3 2/m	[math]\displaystyle{ \Gamma_c^fO_h^7 }[/math]	100a	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4_1//\tilde 6 \cdot m }[/math]	[math]\displaystyle{ 2^+{:}2 }[/math]	[math]\displaystyle{ (*4_14{\cdot}2){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}2_02_1){:}6 }[/math]
228			Fd3c	F 41/d 3 2/c	[math]\displaystyle{ \Gamma_c^fO_h^8 }[/math]	101a	[math]\displaystyle{ \left ( \tfrac{a+c}{2}/\tfrac{b+c}{2}/\tfrac{a+b}{2}:a:a:a\right ) :4_1//\tilde 6 \cdot \tilde c }[/math]	[math]\displaystyle{ 4^{++} }[/math]	[math]\displaystyle{ (*4_14{:}2){:}3 }[/math]	[math]\displaystyle{ (2\bar{*}2_02_1){:}6 }[/math]
229			Im3m	I 4/m 3 2/m	[math]\displaystyle{ \Gamma_c^vO_h^9 }[/math]	72s	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :4/\tilde 6 \cdot m }[/math]	[math]\displaystyle{ 8^\circ{:}2 }[/math]	[math]\displaystyle{ [*{\cdot}4{\cdot}4{:}2]{:}3 }[/math]	[math]\displaystyle{ [2_1{*}{\cdot}2{\cdot}2]{:}6 }[/math]
230			Ia3d	I 41/a 3 2/d	[math]\displaystyle{ \Gamma_c^vO_h^{10} }[/math]	99a	[math]\displaystyle{ \left ( \tfrac{a+b+c}{2}/a:a:a\right ) :4_1//\tilde 6 \cdot \tfrac{1}{2}\widetilde{abc} }[/math]	[math]\displaystyle{ 8^\circ/4 }[/math]	[math]\displaystyle{ (*4_14{:}2){:}3 }[/math]	[math]\displaystyle{ (*2_12{:}2{:}2){:}6 }[/math]

External links

• International Union of Crystallography
• Point Groups and Bravais Lattices
• Full list of 230 crystallographic space groups
• Conway et al. on fibrifold notation