Malkus waterwheel
File:Malkus Waterwheel side by side.webm
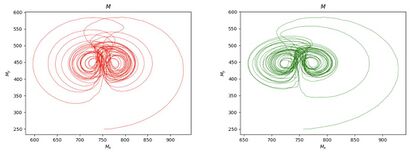
The Malkus waterwheel, also referred to as the Lorenz waterwheel or chaotic waterwheel,[1] is a mechanical model that exhibits chaotic dynamics. Its motion is governed by the Lorenz equations. While classical waterwheels rotate in one direction at a constant speed, the Malkus waterwheel exhibits chaotic motion where its rotation will speed up, slow down, stop, change directions, and oscillate back and forth between combinations of such behaviours in an unpredictable manner.
This variant waterwheel was developed by Willem Malkus in the 1960s.[2] As a pedagogic tool, the Malkus waterwheel became a paradigmatic realization of a chaotic system, and is widely used in the teaching of chaos theory.[3]
In addition to its pedagogic use, the Malkus waterwheel has been actively studied by researchers in dynamical systems and chaos.[4][5][6][7]
In the Malkus waterwheel, a constant flow of water pours in at the top bucket of a simple circular symmetrical waterwheel and the base of each bucket is perforated to allow the outflow of water. At low rates of inflow, the wheel rolls permanently in the same direction. At higher rates of inflow, the waterwheel enters a chaotic regime where it reverses its directionality, accelerates and decelerates in an apparently unpredictable way. A further increase in the incoming flow makes the waterwheel return to a periodic state, where it oscillates back and forth at fixed intervals. Since it was first proposed, many experimental and real-world applications have been related to the waterwheel dynamics. These include instances like the dynamics for electro-rotation, haline oceanic flow, and Rayleigh–Benard convection.[8]
References
- ↑ Strogatz, Steven (2001). Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry and Engineering. Westview Press. ISBN 978-0738204536. https://archive.org/details/nonlineardynamic00stro.
- ↑ MIT News (June 8, 2016). "Willem Malkus, professor emeritus of mathematics, dies at 92". http://news.mit.edu/2016/willem-malkus-professor-emeritus-mathematics-dies-0608.
- ↑ Lorenz, Edward (1995). The Essence Of Chaos. University of Washington press.
- ↑ Kolář, Miroslav; Gumbs, Godfrey (1992). "Theory for the experimental observation of chaos in a rotating waterwheel". Physical Review A 45 (2): 626. doi:10.1103/PhysRevA.45.626. PMID 9907027.
- ↑ Mishra, Aashwin; Sanghi, Sanjeev (2006). "A study of the asymmetric Malkus waterwheel: The biased Lorenz equations". Chaos: An Interdisciplinary Journal of Nonlinear Science 16 (1): 013114. doi:10.1063/1.2154792. PMID 16599745.
- ↑ Matson, Leslie (2007). "The Malkus–Lorenz water wheel revisited". American Journal of Physics 75 (12): 1114–1122. doi:10.1119/1.2785209.
- ↑ Akinlar, Mehmet; Tchier, Fairouz; Mustafa, Inc (2020). "Chaos control and solutions of fractional-order Malkus waterwheel model". Chaos, Solitons & Fractals 135 (2): 109746. doi:10.1016/j.chaos.2020.109746.
- ↑ Alonso, David Becerra (2010). Deterministic chaos in Malkus' waterwheel (PhD). University of the West of Scotland.
 |

