Physics:Transmittance
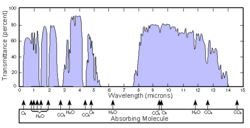
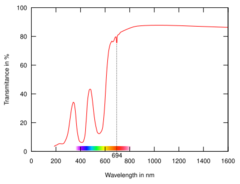
In optical physics, transmittance of the surface of a material is its effectiveness in transmitting radiant energy. It is the fraction of incident electromagnetic power that is transmitted through a sample, in contrast to the transmission coefficient, which is the ratio of the transmitted to incident electric field.[2]
Internal transmittance refers to energy loss by absorption, whereas (total) transmittance is that due to absorption, scattering, reflection, etc.
Mathematical definitions
Hemispherical transmittance
Hemispherical transmittance of a surface, denoted T, is defined as[3]
- [math]\displaystyle{ T = \frac{\Phi_\mathrm{e}^\mathrm{t}}{\Phi_\mathrm{e}^\mathrm{i}}, }[/math]
where
- Φet is the radiant flux transmitted by that surface;
- Φei is the radiant flux received by that surface.
Spectral hemispherical transmittance
Spectral hemispherical transmittance in frequency and spectral hemispherical transmittance in wavelength of a surface, denoted Tν and Tλ respectively, are defined as[3]
- [math]\displaystyle{ T_\nu = \frac{\Phi_{\mathrm{e},\nu}^\mathrm{t}}{\Phi_{\mathrm{e},\nu}^\mathrm{i}}, }[/math]
- [math]\displaystyle{ T_\lambda = \frac{\Phi_{\mathrm{e},\lambda}^\mathrm{t}}{\Phi_{\mathrm{e},\lambda}^\mathrm{i}}, }[/math]
where
- Φe,νt is the spectral radiant flux in frequency transmitted by that surface;
- Φe,νi is the spectral radiant flux in frequency received by that surface;
- Φe,λt is the spectral radiant flux in wavelength transmitted by that surface;
- Φe,λi is the spectral radiant flux in wavelength received by that surface.
Directional transmittance
Directional transmittance of a surface, denoted TΩ, is defined as[3]
- [math]\displaystyle{ T_\Omega = \frac{L_{\mathrm{e},\Omega}^\mathrm{t}}{L_{\mathrm{e},\Omega}^\mathrm{i}}, }[/math]
where
- Le,Ωt is the radiance transmitted by that surface;
- Le,Ωi is the radiance received by that surface.
Spectral directional transmittance
Spectral directional transmittance in frequency and spectral directional transmittance in wavelength of a surface, denoted Tν,Ω and Tλ,Ω respectively, are defined as[3]
- [math]\displaystyle{ T_{\nu,\Omega} = \frac{L_{\mathrm{e},\Omega,\nu}^\mathrm{t}}{L_{\mathrm{e},\Omega,\nu}^\mathrm{i}}, }[/math]
- [math]\displaystyle{ T_{\lambda,\Omega} = \frac{L_{\mathrm{e},\Omega,\lambda}^\mathrm{t}}{L_{\mathrm{e},\Omega,\lambda}^\mathrm{i}}, }[/math]
where
- Le,Ω,νt is the spectral radiance in frequency transmitted by that surface;
- Le,Ω,νi is the spectral radiance received by that surface;
- Le,Ω,λt is the spectral radiance in wavelength transmitted by that surface;
- Le,Ω,λi is the spectral radiance in wavelength received by that surface.
Beer–Lambert law
By definition, internal transmittance is related to optical depth and to absorbance as
- [math]\displaystyle{ T = e^{-\tau} = 10^{-A}, }[/math]
where
- τ is the optical depth;
- A is the absorbance.
The Beer–Lambert law states that, for N attenuating species in the material sample,
- [math]\displaystyle{ T = e^{-\sum_{i = 1}^N \sigma_i \int_0^\ell n_i(z)\mathrm{d}z} = 10^{-\sum_{i = 1}^N \varepsilon_i \int_0^\ell c_i(z)\mathrm{d}z}, }[/math]
or equivalently that
- [math]\displaystyle{ \tau = \sum_{i = 1}^N \tau_i = \sum_{i = 1}^N \sigma_i \int_0^\ell n_i(z)\,\mathrm{d}z, }[/math]
- [math]\displaystyle{ A = \sum_{i = 1}^N A_i = \sum_{i = 1}^N \varepsilon_i \int_0^\ell c_i(z)\,\mathrm{d}z, }[/math]
where
- σi is the attenuation cross section of the attenuating species i in the material sample;
- ni is the number density of the attenuating species i in the material sample;
- εi is the molar attenuation coefficient of the attenuating species i in the material sample;
- ci is the amount concentration of the attenuating species i in the material sample;
- ℓ is the path length of the beam of light through the material sample.
Attenuation cross section and molar attenuation coefficient are related by
- [math]\displaystyle{ \varepsilon_i = \frac{\mathrm{N_A}}{\ln{10}}\,\sigma_i, }[/math]
and number density and amount concentration by
- [math]\displaystyle{ c_i = \frac{n_i}{\mathrm{N_A}}, }[/math]
where NA is the Avogadro constant.
In case of uniform attenuation, these relations become[4]
- [math]\displaystyle{ T = e^{-\sum_{i = 1}^N \sigma_i n_i\ell} = 10^{-\sum_{i = 1}^N \varepsilon_i c_i\ell}, }[/math]
or equivalently
- [math]\displaystyle{ \tau = \sum_{i = 1}^N \sigma_i n_i\ell, }[/math]
- [math]\displaystyle{ A = \sum_{i = 1}^N \varepsilon_i c_i\ell. }[/math]
Cases of non-uniform attenuation occur in atmospheric science applications and radiation shielding theory for instance.
Other radiometric coefficients
See also
References
- ↑ "Electronic warfare and radar systems engineering handbook". http://ewhdbks.mugu.navy.mil/EO-IR.htm#transmission.
- ↑ IUPAC, Compendium of Chemical Terminology, 2nd ed. (the "Gold Book") (1997). Online corrected version: (2006–) "Transmittance". doi:10.1351/goldbook.T06484
- ↑ Jump up to: 3.0 3.1 3.2 3.3 "Thermal insulation — Heat transfer by radiation — Physical quantities and definitions". ISO 9288:1989. ISO catalogue. 1989. http://www.iso.org/iso/home/store/catalogue_tc/catalogue_detail.htm?csnumber=16943.
- ↑ IUPAC, Compendium of Chemical Terminology, 2nd ed. (the "Gold Book") (1997). Online corrected version: (2006–) "Beer–Lambert law". doi:10.1351/goldbook.B00626
 |

