Steiner conic

1. Definition of the Steiner generation of a conic section

The Steiner conic or more precisely Steiner's generation of a conic, named after the Swiss mathematician Jakob Steiner, is an alternative method to define a non-degenerate projective conic section in a projective plane over a field.

The usual definition of a conic uses a quadratic form (see Quadric (projective geometry)). Another alternative definition of a conic uses a hyperbolic polarity. It is due to K. G. C. von Staudt and sometimes called a von Staudt conic. The disadvantage of von Staudt's definition is that it only works when the underlying field has odd characteristic (i.e., [math]\displaystyle{ Char\ne2 }[/math]).

Definition of a Steiner conic

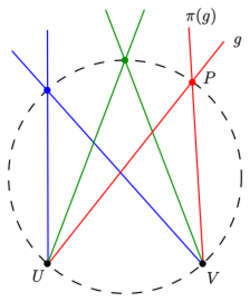
• Given two pencils [math]\displaystyle{ B(U),B(V) }[/math] of lines at two points [math]\displaystyle{ U,V }[/math] (all lines containing [math]\displaystyle{ U }[/math] and [math]\displaystyle{ V }[/math] resp.) and a projective but not perspective mapping [math]\displaystyle{ \pi }[/math] of [math]\displaystyle{ B(U) }[/math] onto [math]\displaystyle{ B(V) }[/math]. Then the intersection points of corresponding lines form a non-degenerate projective conic section (figure 1)

2. Perspective mapping between lines

A perspective mapping [math]\displaystyle{ \pi }[/math] of a pencil [math]\displaystyle{ B(U) }[/math] onto a pencil [math]\displaystyle{ B(V) }[/math] is a bijection (1-1 correspondence) such that corresponding lines intersect on a fixed line [math]\displaystyle{ a }[/math], which is called the axis of the perspectivity [math]\displaystyle{ \pi }[/math] (figure 2).

A projective mapping is a finite product of perspective mappings.

Simple example: If one shifts in the first diagram point [math]\displaystyle{ U }[/math] and its pencil of lines onto [math]\displaystyle{ V }[/math] and rotates the shifted pencil around [math]\displaystyle{ V }[/math] by a fixed angle [math]\displaystyle{ \varphi }[/math] then the shift (translation) and the rotation generate a projective mapping [math]\displaystyle{ \pi }[/math] of the pencil at point [math]\displaystyle{ U }[/math] onto the pencil at [math]\displaystyle{ V }[/math]. From the inscribed angle theorem one gets: The intersection points of corresponding lines form a circle.

Examples of commonly used fields are the real numbers [math]\displaystyle{ \R }[/math], the rational numbers [math]\displaystyle{ \Q }[/math] or the complex numbers [math]\displaystyle{ \C }[/math]. The construction also works over finite fields, providing examples in finite projective planes.

Remark: The fundamental theorem for projective planes states, that a projective mapping in a projective plane over a field (pappian plane) is uniquely determined by prescribing the images of three lines. That means that, for the Steiner generation of a conic section, besides two points [math]\displaystyle{ U,V }[/math] only the images of 3 lines have to be given. These 5 items (2 points, 3 lines) uniquely determine the conic section.

Remark: The notation "perspective" is due to the dual statement: The projection of the points on a line [math]\displaystyle{ a }[/math] from a center [math]\displaystyle{ Z }[/math] onto a line [math]\displaystyle{ b }[/math] is called a perspectivity (see below).

3. Example of a Steiner generation: generation of a point

Example

For the following example the images of the lines [math]\displaystyle{ a,u,w }[/math] (see picture) are given: [math]\displaystyle{ \pi(a)=b, \pi(u)=w, \pi(w)=v }[/math]. The projective mapping [math]\displaystyle{ \pi }[/math] is the product of the following perspective mappings [math]\displaystyle{ \pi_b,\pi_a }[/math]: 1) [math]\displaystyle{ \pi_b }[/math] is the perspective mapping of the pencil at point [math]\displaystyle{ U }[/math] onto the pencil at point [math]\displaystyle{ O }[/math] with axis [math]\displaystyle{ b }[/math]. 2) [math]\displaystyle{ \pi_a }[/math] is the perspective mapping of the pencil at point [math]\displaystyle{ O }[/math] onto the pencil at point [math]\displaystyle{ V }[/math] with axis [math]\displaystyle{ a }[/math]. First one should check that [math]\displaystyle{ \pi=\pi_a\pi_b }[/math] has the properties: [math]\displaystyle{ \pi(a)=b, \pi(u)=w, \pi(w)=v }[/math]. Hence for any line [math]\displaystyle{ g }[/math] the image [math]\displaystyle{ \pi(g)=\pi_a\pi_b(g) }[/math] can be constructed and therefore the images of an arbitrary set of points. The lines [math]\displaystyle{ u }[/math] and [math]\displaystyle{ v }[/math] contain only the conic points [math]\displaystyle{ U }[/math] and [math]\displaystyle{ V }[/math] resp.. Hence [math]\displaystyle{ u }[/math] and [math]\displaystyle{ v }[/math] are tangent lines of the generated conic section.

A proof that this method generates a conic section follows from switching to the affine restriction with line [math]\displaystyle{ w }[/math] as the line at infinity, point [math]\displaystyle{ O }[/math] as the origin of a coordinate system with points [math]\displaystyle{ U,V }[/math] as points at infinity of the x- and y-axis resp. and point [math]\displaystyle{ E=(1,1) }[/math]. The affine part of the generated curve appears to be the hyperbola [math]\displaystyle{ y=1/x }[/math].

Remark:

1. The Steiner generation of a conic section provides simple methods for the construction of ellipses, parabolas and hyperbolas which are commonly called the parallelogram methods.
2. The figure that appears while constructing a point (figure 3) is the 4-point-degeneration of Pascal's theorem.

Steiner generation of a dual conic

dual ellipse

Steiner generation of a dual conic

definition of a perspective mapping

Definitions and the dual generation

Dualizing (see duality (projective geometry)) a projective plane means exchanging the points with the lines and the operations intersection and connecting. The dual structure of a projective plane is also a projective plane. The dual plane of a pappian plane is pappian and can also be coordinatized by homogeneous coordinates. A nondegenerate dual conic section is analogously defined by a quadratic form.

A dual conic can be generated by Steiner's dual method:

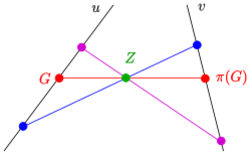
• Given the point sets of two lines [math]\displaystyle{ u,v }[/math] and a projective but not perspective mapping [math]\displaystyle{ \pi }[/math] of [math]\displaystyle{ u }[/math] onto [math]\displaystyle{ v }[/math]. Then the lines connecting corresponding points form a dual non-degenerate projective conic section.

A perspective mapping [math]\displaystyle{ \pi }[/math] of the point set of a line [math]\displaystyle{ u }[/math] onto the point set of a line [math]\displaystyle{ v }[/math] is a bijection (1-1 correspondence) such that the connecting lines of corresponding points intersect at a fixed point [math]\displaystyle{ Z }[/math], which is called the centre of the perspectivity [math]\displaystyle{ \pi }[/math] (see figure).

A projective mapping is a finite sequence of perspective mappings.

It is usual, when dealing with dual and common conic sections, to call the common conic section a point conic and the dual conic a line conic.

In the case that the underlying field has [math]\displaystyle{ \operatorname{Char} =2 }[/math] all the tangents of a point conic intersect in a point, called the knot (or nucleus) of the conic. Thus, the dual of a non-degenerate point conic is a subset of points of a dual line and not an oval curve (in the dual plane). So, only in the case that [math]\displaystyle{ \operatorname{Char}\ne2 }[/math] is the dual of a non-degenerate point conic a non-degenerate line conic.

Examples

Dual Steiner conic defined by two perspectivities [math]\displaystyle{ \pi_A, \pi_B }[/math]

example of a Steiner generation of a dual conic

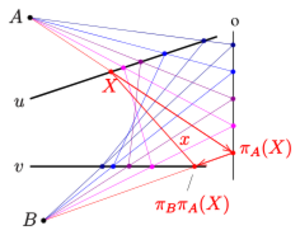
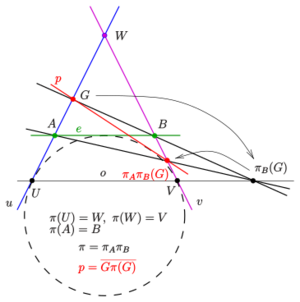
(1) Projectivity given by two perspectivities:
Two lines [math]\displaystyle{ u,v }[/math] with intersection point [math]\displaystyle{ W }[/math] are given and a projectivity [math]\displaystyle{ \pi }[/math] from [math]\displaystyle{ u }[/math] onto [math]\displaystyle{ v }[/math] by two perspectivities [math]\displaystyle{ \pi_A,\pi_B }[/math] with centers [math]\displaystyle{ A,B }[/math]. [math]\displaystyle{ \pi_A }[/math] maps line [math]\displaystyle{ u }[/math] onto a third line [math]\displaystyle{ o }[/math], [math]\displaystyle{ \pi_B }[/math] maps line [math]\displaystyle{ o }[/math] onto line [math]\displaystyle{ v }[/math] (see diagram). Point [math]\displaystyle{ W }[/math] must not lie on the lines [math]\displaystyle{ \overline{AB},o }[/math]. Projectivity [math]\displaystyle{ \pi }[/math] is the composition of the two perspectivities: [math]\displaystyle{ \ \pi=\pi_B\pi_A }[/math]. Hence a point [math]\displaystyle{ X }[/math] is mapped onto [math]\displaystyle{ \pi(X)=\pi_B\pi_A(X) }[/math] and the line [math]\displaystyle{ x=\overline{X\pi(X)} }[/math] is an element of the dual conic defined by [math]\displaystyle{ \pi }[/math].
(If [math]\displaystyle{ W }[/math] would be a fixpoint, [math]\displaystyle{ \pi }[/math] would be perspective.)

(2) Three points and their images are given:
The following example is the dual one given above for a Steiner conic.
The images of the points [math]\displaystyle{ A,U,W }[/math] are given: [math]\displaystyle{ \pi(A)=B, \, \pi(U)=W,\, \pi(W)=V }[/math]. The projective mapping [math]\displaystyle{ \pi }[/math] can be represented by the product of the following perspectivities [math]\displaystyle{ \pi_B,\pi_A }[/math]:

1. [math]\displaystyle{ \pi_B }[/math] is the perspectivity of the point set of line [math]\displaystyle{ u }[/math] onto the point set of line [math]\displaystyle{ o }[/math] with centre [math]\displaystyle{ B }[/math].
2. [math]\displaystyle{ \pi_A }[/math] is the perspectivity of the point set of line [math]\displaystyle{ o }[/math] onto the point set of line [math]\displaystyle{ v }[/math] with centre [math]\displaystyle{ A }[/math].

One easily checks that the projective mapping [math]\displaystyle{ \pi=\pi_A\pi_B }[/math] fulfills [math]\displaystyle{ \pi(A)=B,\, \pi(U)=W, \, \pi(W)=V }[/math]. Hence for any arbitrary point [math]\displaystyle{ G }[/math] the image [math]\displaystyle{ \pi(G)=\pi_A\pi_B(G) }[/math] can be constructed and line [math]\displaystyle{ \overline{G\pi(G)} }[/math] is an element of a non degenerate dual conic section. Because the points [math]\displaystyle{ U }[/math] and [math]\displaystyle{ V }[/math] are contained in the lines [math]\displaystyle{ u }[/math], [math]\displaystyle{ v }[/math] resp.,the points [math]\displaystyle{ U }[/math] and [math]\displaystyle{ V }[/math] are points of the conic and the lines [math]\displaystyle{ u,v }[/math] are tangents at [math]\displaystyle{ U,V }[/math].

Intrinsic conics in a linear incidence geometry

The Steiner construction defines the conics in a planar linear incidence geometry (two points determine at most one line and two lines intersect in at most one point) intrinsically, that is, using only the collineation group. Specifically, [math]\displaystyle{ E(T,P) }[/math] is the conic at point [math]\displaystyle{ P }[/math] afforded by the collineation [math]\displaystyle{ T }[/math], consisting of the intersections of [math]\displaystyle{ L }[/math] and [math]\displaystyle{ T(L) }[/math] for all lines [math]\displaystyle{ L }[/math] through [math]\displaystyle{ P }[/math]. If [math]\displaystyle{ T(P)=P }[/math] or [math]\displaystyle{ T(L)=L }[/math] for some [math]\displaystyle{ L }[/math] then the conic is degenerate. For example, in the real coordinate plane, the affine type (ellipse, parabola, hyperbola) of [math]\displaystyle{ E(T,P) }[/math] is determined by the trace and determinant of the matrix component of [math]\displaystyle{ T }[/math], independent of [math]\displaystyle{ P }[/math].

By contrast, the collineation group of the real hyperbolic plane [math]\displaystyle{ \mathbb{H}^2 }[/math]consists of isometries. Consequently, the intrinsic conics comprise a small but varied subset of the general conics, curves obtained from the intersections of projective conics with a hyperbolic domain. Further, unlike the Euclidean plane, there is no overlap between the direct [math]\displaystyle{ E(T,P); }[/math] [math]\displaystyle{ T }[/math] preserves orientation – and the opposite [math]\displaystyle{ E(T,P); }[/math] [math]\displaystyle{ T }[/math] reverses orientation. The direct case includes central (two perpendicular lines of symmetry) and non-central conics, whereas every opposite conic is central. Even though direct and opposite central conics cannot be congruent, they are related by a quasi-symmetry defined in terms of complementary angles of parallelism. Thus, in any inversive model of [math]\displaystyle{ \mathbb{H}^2 }[/math], each direct central conic is birationally equivalent to an opposite central conic. In fact, the central conics represent all genus 1 curves with real shape invariant [math]\displaystyle{ j\geq1 }[/math]. A minimal set of representatives is obtained from the central direct conics with common center and axis of symmetry, whereby the shape invariant is a function of the eccentricity, defined in terms of the distance between [math]\displaystyle{ P }[/math] and [math]\displaystyle{ T(P) }[/math]. The orthogonal trajectories of these curves represent all genus 1 curves with [math]\displaystyle{ j\leq1 }[/math], which manifest as either irreducible cubics or bi-circular quartics. Using the elliptic curve addition law on each trajectory, every general central conic in [math]\displaystyle{ \mathbb{H}^2 }[/math]decomposes uniquely as the sum of two intrinsic conics by adding pairs of points where the conics intersect each trajectory.

References

• Coxeter, H. S. M. (1993), The Real Projective Plane, Springer Science & Business Media 
• Hartmann, Erich, Planar Circle Geometries, an Introduction to Moebius-, Laguerre- and Minkowski Planes, http://www.mathematik.tu-darmstadt.de/~ehartmann/circlegeom.pdf, retrieved 20 September 2014  (PDF; 891 kB).
• Merserve, Bruce E. (1983) [1959], Fundamental Concepts of Geometry, Dover, ISBN 0-486-63415-9