Wong graph
From HandWiki
Revision as of 09:40, 8 March 2021 by imported>Jslovo (over-write)
| Wong graph | |
|---|---|
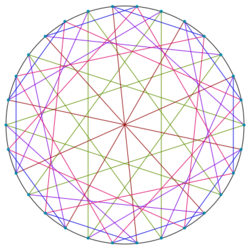 | |
| Named after | Pak-Ken Wong |
| Vertices | 30 |
| Edges | 75 |
| Radius | 3 |
| Diameter | 3 |
| Girth | 5 |
| Automorphisms | 96 |
| Chromatic number | 4 |
| Chromatic index | 5 |
| Properties | Cage |
| Table of graphs and parameters | |
In the mathematical field of graph theory, the Wong graph is a 5-regular undirected graph with 30 vertices and 75 edges.[1][2] It is one of the four (5,5)-cage graphs, the others being the Foster cage, the Meringer graph, and the Robertson–Wegner graph.
Like the unrelated Harries–Wong graph, it is named after Pak-Ken Wong.[3]
It has chromatic number 4, diameter 3, and is 5-vertex-connected.
Algebraic properties
The characteristic polynomial of the Wong graph is
- [math]\displaystyle{ (x-5)(x+1)^2(x^2-5)^3(x-1)^5(x^2+x-5)^8. }[/math]
References
- ↑ Weisstein, Eric W.. "Wong Graph". http://mathworld.wolfram.com/WongGraph.html.
- ↑ Meringer, Markus (1999), "Fast generation of regular graphs and construction of cages", Journal of Graph Theory 30 (2): 137–146, doi:10.1002/(SICI)1097-0118(199902)30:2<137::AID-JGT7>3.0.CO;2-G.
- ↑ Wong, P. K. "Cages--A Survey." J. Graph Th. 6, 1-22, 1982.
 |

