Medicine:Windkessel effect
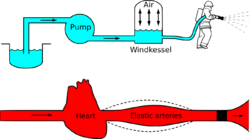
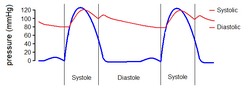
Windkessel effect is a term used in medicine to account for the shape of the arterial blood pressure waveform in terms of the interaction between the stroke volume and the compliance of the aorta and large elastic arteries (Windkessel vessels) and the resistance of the smaller arteries and arterioles. Windkessel when loosely translated from German to English means 'air chamber',[1][2] but is generally taken to imply an elastic reservoir.[3] The walls of large elastic arteries (e.g. aorta, common carotid, subclavian, and pulmonary arteries and their larger branches) contain elastic fibers, formed of elastin. These arteries distend when the blood pressure rises during systole and recoil when the blood pressure falls during diastole. Since the rate of blood entering these elastic arteries exceeds that leaving them via the peripheral resistance, there is a net storage of blood in the aorta and large arteries during systole, which discharges during diastole. The compliance (or distensibility) of the aorta and large elastic arteries is therefore analogous to a capacitor (employing the hydraulic analogy); to put it another way, these arteries collectively act as a hydraulic accumulator.
The Windkessel effect helps in damping the fluctuation in blood pressure (pulse pressure) over the cardiac cycle and assists in the maintenance of organ perfusion during diastole when cardiac ejection ceases. The idea of the Windkessel was alluded to by Giovanni Borelli, although Stephen Hales articulated the concept more clearly and drew the analogy with an air chamber used in fire engines in the 18th century.[4] Otto Frank, an influential German physiologist, developed the concept and provided a firm mathematical foundation.[2] Frank's model is sometimes called a two-element Windkessel to distinguish it from more recent and more elaborate Windkessel models (e.g. three- or four-element and non-linear Windkessel models).[5][6]
Model types
Modeling of a Windkessel
Windkessel physiology remains a relevant yet dated description of important clinical interest. The historic mathematical definition of systole and diastole in the model are obviously not novel but are here elementally staged to four degrees. Reaching five would be original work.[citation needed]
Two-element
It is assumed that the ratio of pressure to volume is constant and that outflow from the Windkessel is proportional to the fluid pressure. Volumetric inflow must equal the sum of the volume stored in the capacitive element and volumetric outflow through the resistive element. This relationship is described by a differential equation:[citation needed]
[math]\displaystyle{ I(t)={P(t)\over R}+C{dP(t)\over dt} }[/math]
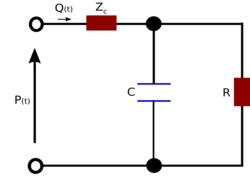
I(t) is volumetric inflow due to the pump (heart) and is measured in volume per unit time, while P(t) is the pressure with respect to time measured in force per unit area, C is the ratio of volume to pressure for the Windkessel, and R is the resistance relating outflow to fluid pressure. This model is identical to the relationship between current, I(t), and electrical potential, P(t), in an electrical circuit equivalent of the two-element Windkessel model.[citation needed]
In the blood circulation, the passive elements in the circuit are assumed to represent elements in the cardiovascular system. The resistor, R, represents the total peripheral resistance and the capacitor, C, represents total arterial compliance.[7]
During diastole there is no blood inflow since the aortic (or pulmonary valve) is closed, so the Windkessel can be solved for P(t) since I(t) = 0:
[math]\displaystyle{ P(t)=P(t_d)e^{-(t-t_d)\over (RC)} }[/math]
where td is the time of the start of diastole and P(td) is the blood pressure at the start of diastole. This model is only a rough approximation of the arterial circulation; more realistic models incorporate more elements, provide more realistic estimates of the blood pressure waveform and are discussed below.
Three-element
The three-element Windkessel improves on the two-element model by incorporating another resistive element to simulate resistance to blood flow due to the characteristic resistance of the aorta (or pulmonary artery). The differential equation for the 3-element model is:[citation needed]
[math]\displaystyle{ (1+{R_1\over R_2})I(t)+CR_1{dI(t)\over dt}= {P(t)\over R_2}+C{dP(t)\over dt} }[/math]
where R1 is the characteristic resistance (this is assumed to be equivalent to the characteristic impedance),[7] while R2 represents the peripheral resistance. This model is widely used as an acceptable model of the circulation.[5] For example it has been employed to evaluate blood pressure and flow in the aorta of a chick embryo [8] and the pulmonary artery in a pig[8] as well as providing the basis for construction of physical models of the circulation providing realistic loads for experimental studies of isolated hearts.[9]
Four-element
The three-element model overestimates the compliance and underestimates the characteristic impedance of the circulation.[7] The four-element model includes an inductor, L, which has units of mass per length, ([math]\displaystyle{ {M\over l^4} }[/math]), into the proximal component of the circuit to account for the inertia of blood flow. This is neglected in the two- and three- element models. The relevant equation is:
[math]\displaystyle{ (1+{R_1\over R_2})I(t)+(R_1C+{L\over R_2}){dI(t)\over dt}+LC{d^2I(t)\over dt^2}={P(t)\over R_2}+C{dP(t)\over dt} }[/math]
Applications
These models relate blood flow to blood pressure through parameters of R, C (and, in the case of the four-element model, L). These equations can be easily solved (e.g. by employing MATLAB and its supplement SIMULINK) to either find the values of pressure given flow and R, C, L parameters, or find values of R, C, L given flow and pressure. An example for the two-element model is shown below, where I(t) is depicted as an input signal during systole and diastole. Systole is represented by the sin function, while flow during diastole is zero. s represents the duration of the cardiac cycle, while Ts represents the duration of systole, and Td represents the duration of diastole (e.g. in seconds).[citation needed]
[math]\displaystyle{ I(t)=I_o\sin[{(\pi*{t\over s})\over Ts}] \text{ for } {t\over s}\leq Ts }[/math]
[math]\displaystyle{ I(t)=0\text{ for } Ts\lt (Td+Ts) }[/math]
In physiology and disease
The 'Windkessel effect' becomes diminished with age as the elastic arteries become less compliant, termed hardening of the arteries or arteriosclerosis, probably secondary to fragmentation and loss of elastin.[10] The reduction in the Windkessel effect results in increased pulse pressure for a given stroke volume. The increased pulse pressure results in elevated systolic pressure (hypertension) which increases the risk of myocardial infarction, stroke, heart failure and a variety of other cardiovascular diseases.[11]
Limitations
Although the Windkessel is a simple and convenient concept, it has been largely superseded by more modern approaches that interpret arterial pressure and flow waveforms in terms of wave propagation and reflection.[12] Recent attempts to integrate wave propagation and Windkessel approaches through a reservoir concept,[13] have been criticized[14][15] and a recent consensus document highlighted the wave-like nature of the reservoir.[16]
See also
- Physics:Hydraulic accumulator – Reservoir to store and stabilise fluid pressure
References
- ↑ "Translation of Otto Frank's paper "Die Grundform des Arteriellen Pulses" Zeitschrift für Biologie 37: 483-526 (1899)". Journal of Molecular and Cellular Cardiology 22 (3): 253–4. March 1990. doi:10.1016/0022-2828(90)91459-K. PMID 2192068.
- ↑ 2.0 2.1 "The basic shape of the arterial pulse. First treatise: mathematical analysis. 1899". Journal of Molecular and Cellular Cardiology 22 (3): 255–77. March 1990. doi:10.1016/0022-2828(90)91460-O. PMID 21438422.
- ↑ Review of Medical Physiology (Twenty-Second ed.). The McGraw-Hill Companies, Inc.. 2005. p. 587. ISBN 9780071440400.
- ↑ Hales, Stephen (1733). Statical Essays: Haemastaticks.
- ↑ 5.0 5.1 "The arterial Windkessel". Medical & Biological Engineering & Computing 47 (2): 131–41. February 2009. doi:10.1007/s11517-008-0359-2. PMID 18543011.
- ↑ "Identification of the three-element windkessel model incorporating a pressure-dependent compliance". Annals of Biomedical Engineering 23 (2): 164–77. March 1995. doi:10.1007/bf02368323. PMID 7605053.
- ↑ 7.0 7.1 7.2 Snapshots of hemodynamics : an aid for clinical research and graduate education (2nd ed.). New York: Springer. 2010. ISBN 9781441963635. OCLC 676701119.
- ↑ 8.0 8.1 Kerner, Daniel R.. "Solving Windkessel Models with MLAB". Civilized Software, Inc.. http://www.civilized.com/mlabexamples/windkesmodel.htmld/.
- ↑ "An artificial arterial system for pumping hearts". Journal of Applied Physiology 31 (5): 776–81. November 1971. doi:10.1152/jappl.1971.31.5.776. PMID 5117196.
- ↑ "Ageing of the conduit arteries". The Journal of Pathology 211 (2): 157–72. January 2007. doi:10.1002/path.2101. PMID 17200940.
- ↑ "Age-specific relevance of usual blood pressure to vascular mortality: a meta-analysis of individual data for one million adults in 61 prospective studies". Lancet 360 (9349): 1903–13. December 2002. doi:10.1016/S0140-6736(02)11911-8. PMID 12493255.
- ↑ McDonald's Blood Flow in Arteries: Theoretical, Experimental and Clinical Principles (5th ed.). Hodder Arnold Publication. 2005. ISBN 9780340809419.
- ↑ "Wave intensity analysis and the development of the reservoir-wave approach". Medical & Biological Engineering & Computing 47 (2): 221–32. February 2009. doi:10.1007/s11517-008-0430-z. PMID 19189147.
- ↑ "Reservations on the reservoir". Journal of Hypertension 30 (4): 676–8. April 2012. doi:10.1097/HJH.0b013e32835077be. PMID 22418902.
- ↑ "Wave Separation, Wave Intensity, the Reservoir-Wave Concept, and the Instantaneous Wave-Free Ratio: Presumptions and Principles". Hypertension 66 (1): 93–8. July 2015. doi:10.1161/HYPERTENSIONAHA.115.05567. PMID 26015448.
- ↑ "Towards a consensus on the understanding and analysis of the pulse waveform: Results from the 2016 Workshop on Arterial Hemodynamics: Past, present and future". Artery Research 18: 75–80. June 2017. doi:10.1016/j.artres.2017.03.004. PMID 28626494.
 |