Physics:Reduced dimensions form

From HandWiki
Revision as of 02:36, 5 February 2024 by Wincert (talk | contribs) (change)
(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)

In biophysics and related fields, reduced dimension forms (RDFs) are unique on-off mechanisms for random walks that generate two-state trajectories (see Fig. 1 for an example of a RDF and Fig. 2 for an example of a two-state trajectory). It has been shown that RDFs solve two-state trajectories, since only one RDF can be constructed from the data,[1] where this property does not hold for on-off kinetic schemes, where many kinetic schemes can be constructed from a particular two-state trajectory (even from an ideal on-off trajectory). Two-state time trajectories are very common in measurements in chemistry, physics, and the biophysics of individual molecules[2][3] (e.g. measurements of protein dynamics and DNA and RNA dynamics,[4][5][6][7][8][9][10] activity of ion channels,[11][12][13] enzyme activity,[14][15][16][17][18][19][20][21][22][23][24] quantum dots [25][26][27][28][29][30][31][32]), thus making RDFs an important tool in the analysis of data in these fields.

Since RDFs are uniquely obtained from the data,[33][34] they have many advantages over other mathematical and statistical methods that were developed for solving two-state trajectories.[35][36][37][38][39][40][41][42][43][44][45][46][47]

Figure 1 A 3on3 RDF
Figure 2 Two-state trajectories

Description of RDF

A RDF is a lattice of substates, each substate represents either the on state or the off state, and has a particular number (see Figure 1). The connections are only among substates of different states. A simulation of an on-off trajectory from a RDF is made with a generalized Gillespie algorithm, where here a random jumping time is first taken from density functions that are (usually) not exponential using the rejection method, and then the specific next substate is chosen according to the jumping probabilities that are determined from the jumping time probability density functions. A RDF can have irreversible connections, yet, it generates an on-off trajectory that has the property of microscopic reversibility, meaning that the physical system fluctuates around equilibrium.

Two-state trajectories

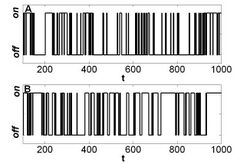
A two-state trajectory is a fluctuating signal made of on periods and off periods; an on period, and then an off period, and so on (see, Fig. 2). In most cases where this signal appears in applications in science, the trajectory is random; that is, the length of the on and off periods changes, and is a random quantity. There may be correlations in the trajectory; e.g., when we see a short off period and the next on period is relatively long (that is, long with a large probability), we say that there are off-on correlations. In principle, there are 4 independent types of correlations in two-state trajectories: on-on, on-off, off-on, and off-off. Two-state trajectories can be obtained from on-off kinetic schemes, RDFs, or any other stochastic equation of motion (with a clear on-off definition). In experiments from individual molecules, two-state trajectories are common, where from the trajectory we aim at finding the right model of the process.[48]

Using RDFs in solving two-state trajectories

Properties of RDFs in solving two-state trajectories

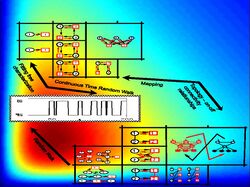
Figure3 A two-state trajectory, RDFs and kinetic schemes, and the relations among these.

It was shown in Ref. 1[1] that RDFs are unique is the sense that a particular RDF generates a particular time trajectory (in a statistical sense), and a time trajectory is associated with only one RDF. This property does not hold for on-off kinetic schemes, where from a trajectory several kinetic schemes can be constructed ; see for example,.[1] RDFs are also constructed more reliably from the data than kinetic schemes.[33] Figure 3 illustrates RDFs, kinetic schemes and two-state trajectories, and the relations among these. Given a two-state trajectory (generated from any mechanism), it is safer to go from the data and construct a RDF, rather than trying to construct the kinetic scheme from the data directly. With the constructed RDF, one can find several possible kinetic schemes very accurately (usually, one eventually tries constructing a kinetic scheme from the data), where these kinetic schemes are all equivalent (with regard to the data).

The software RDF

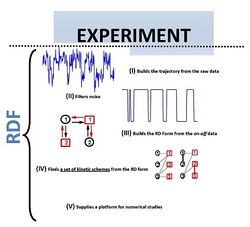
  • Based on RDFs, software for deducing the correct mechanisms from real data (e.g. two-state trajectories) is designed.[49] See Figure 4 for an illustration of the aims of the software. The software is named RDF.
Figure 4 Here, shown are the stages of the software: first, take a noisy trajectory and clean it; then, find the RDF from the cleaned data. Finally, suggest a set of kinetic schemes that may be related with the found RDF. The software should also present the possibility of numerical simulations of noisy time trajectories.

References

  1. ↑ 1.0 1.1 1.2 Flomenbom, O.; Silbey, R. J. (2006-07-10). "Utilizing the information content in two-state trajectories". Proceedings of the National Academy of Sciences 103 (29): 10907–10910. doi:10.1073/pnas.0604546103. ISSN 0027-8424. PMID 16832051. Bibcode: 2006PNAS..10310907F. 
  2. ↑ Moerner, W. E. (1999-03-12). "Illuminating Single Molecules in Condensed Matter". Science (American Association for the Advancement of Science (AAAS)) 283 (5408): 1670–1676. doi:10.1126/science.283.5408.1670. ISSN 0036-8075. PMID 10073924. Bibcode: 1999Sci...283.1670M. 
  3. ↑ Weiss, S. (1999-03-12). "Fluorescence Spectroscopy of Single Biomolecules". Science (American Association for the Advancement of Science (AAAS)) 283 (5408): 1676–1683. doi:10.1126/science.283.5408.1676. ISSN 0036-8075. PMID 10073925. Bibcode: 1999Sci...283.1676W. 
  4. ↑ Schuler, Benjamin; Lipman, Everett A.; Eaton, William A. (2002). "Probing the free-energy surface for protein folding with single-molecule fluorescence spectroscopy". Nature (Springer Nature) 419 (6908): 743–747. doi:10.1038/nature01060. ISSN 0028-0836. PMID 12384704. Bibcode: 2002Natur.419..743S. https://zenodo.org/record/1233253. 
  5. ↑ Yang, H. (2003-10-10). "Protein Conformational Dynamics Probed by Single-Molecule Electron Transfer". Science (American Association for the Advancement of Science (AAAS)) 302 (5643): 262–266. doi:10.1126/science.1086911. ISSN 0036-8075. PMID 14551431. Bibcode: 2003Sci...302..262Y. 
  6. ↑ Min, Wei; Luo, Guobin; Cherayil, Binny J.; Kou, S. C.; Xie, X. Sunney (2005-05-18). "Observation of a Power-Law Memory Kernel for Fluctuations within a Single Protein Molecule". Physical Review Letters (American Physical Society (APS)) 94 (19): 198302. doi:10.1103/physrevlett.94.198302. ISSN 0031-9007. PMID 16090221. Bibcode: 2005PhRvL..94s8302M. 
  7. ↑ Rhoades, E.; Gussakovsky, E.; Haran, G. (2003-02-28). "Watching proteins fold one molecule at a time". Proceedings of the National Academy of Sciences 100 (6): 3197–3202. doi:10.1073/pnas.2628068100. ISSN 0027-8424. PMID 12612345. Bibcode: 2003PNAS..100.3197R. 
  8. ↑ Zhuang, X. (2002-05-24). "Correlating Structural Dynamics and Function in Single Ribozyme Molecules". Science (American Association for the Advancement of Science (AAAS)) 296 (5572): 1473–1476. doi:10.1126/science.1069013. ISSN 0036-8075. PMID 12029135. Bibcode: 2002Sci...296.1473Z. 
  9. ↑ Barsegov, V.; Thirumalai, D. (2005-10-10). "Probing Protein-Protein Interactions by Dynamic Force Correlation Spectroscopy". Physical Review Letters (American Physical Society (APS)) 95 (16): 168302. doi:10.1103/physrevlett.95.168302. ISSN 0031-9007. PMID 16241846. Bibcode: 2005PhRvL..95p8302B. 
  10. ↑ Kolomeisky, Anatoly B.; Fisher, Michael E. (2000-12-22). "Extended kinetic models with waiting-time distributions: Exact results". The Journal of Chemical Physics (AIP Publishing) 113 (24): 10867–10877. doi:10.1063/1.1326912. ISSN 0021-9606. Bibcode: 2000JChPh.11310867K. 
  11. ↑ NEHER, ERWIN; SAKMANN, BERT (1976). "Single-channel currents recorded from membrane of denervated frog muscle fibres". Nature (Springer Science and Business Media LLC) 260 (5554): 799–802. doi:10.1038/260799a0. ISSN 0028-0836. PMID 1083489. Bibcode: 1976Natur.260..799N. 
  12. ↑ Kasianowicz, J. J.; Brandin, E.; Branton, D.; Deamer, D. W. (1996-11-26). "Characterization of individual polynucleotide molecules using a membrane channel". Proceedings of the National Academy of Sciences (Proceedings of the National Academy of Sciences USA) 93 (24): 13770–13773. doi:10.1073/pnas.93.24.13770. ISSN 0027-8424. PMID 8943010. Bibcode: 1996PNAS...9313770K. 
  13. ↑ Kullman, Lisen; Gurnev, Philip A.; Winterhalter, Mathias; Bezrukov, Sergey M. (2006-01-23). "Functional Subconformations in Protein Folding: Evidence from Single-Channel Experiments". Physical Review Letters (American Physical Society (APS)) 96 (3): 038101–038104. doi:10.1103/physrevlett.96.038101. ISSN 0031-9007. PMID 16486775. Bibcode: 2006PhRvL..96c8101K. 
  14. ↑ Lu, H. P.; Xun, L.; Xie, X. S. (1998-12-04). "Single-Molecule Enzymatic Dynamics". Science (American Association for the Advancement of Science (AAAS)) 282 (5395): 1877–1882. doi:10.1126/science.282.5395.1877. PMID 9836635. 
  15. ↑ Edman, Lars; Földes-Papp, Zeno; Wennmalm, Stefan; Rigler, Rudolf (1999). "The fluctuating enzyme: a single molecule approach". Chemical Physics (Elsevier BV) 247 (1): 11–22. doi:10.1016/s0301-0104(99)00098-1. ISSN 0301-0104. Bibcode: 1999CP....247...11E. 
  16. ↑ Velonia, Kelly; Flomenbom, Ophir; Loos, Davey; Masuo, Sadahiro; Cotlet, Mircea; Engelborghs, Yves; Hofkens, Johan; Rowan, Alan E. et al. (2005-01-14). "Single-Enzyme Kinetics of CALB-Catalyzed Hydrolysis". Angewandte Chemie International Edition (Wiley) 44 (4): 560–564. doi:10.1002/anie.200460625. ISSN 1433-7851. PMID 15619259. 
  17. ↑ Flomenbom, O.; Velonia, K.; Loos, D.; Masuo, S.; Cotlet, M. et al. (2005-02-04). "Stretched exponential decay and correlations in the catalytic activity of fluctuating single lipase molecules". Proceedings of the National Academy of Sciences 102 (7): 2368–2372. doi:10.1073/pnas.0409039102. ISSN 0027-8424. PMID 15695587. Bibcode: 2005PNAS..102.2368F. 
  18. ↑ English, Brian P; Min, Wei; van Oijen, Antoine M; Lee, Kang Taek; Luo, Guobin et al. (2005-12-25). "Ever-fluctuating single enzyme molecules: Michaelis-Menten equation revisited". Nature Chemical Biology (Springer Science and Business Media LLC) 2 (2): 87–94. doi:10.1038/nchembio759. ISSN 1552-4450. PMID 16415859. 
  19. ↑ Agmon, Noam (2000). "Conformational Cycle of a Single Working Enzyme". The Journal of Physical Chemistry B (American Chemical Society (ACS)) 104 (32): 7830–7834. doi:10.1021/jp0012911. ISSN 1520-6106. 
  20. ↑ Qian, Hong; L. Elson, Elliot (2002). "Single-molecule enzymology: stochastic Michaelis–Menten kinetics". Biophysical Chemistry (Elsevier BV) 101-102: 565–576. doi:10.1016/s0301-4622(02)00145-x. ISSN 0301-4622. PMID 12488027. 
  21. ↑ Kou, S. C.; Cherayil, Binny J.; Min, Wei; English, Brian P.; Xie, X. Sunney (2005). "Single-Molecule Michaelis−Menten Equations". The Journal of Physical Chemistry B (American Chemical Society (ACS)) 109 (41): 19068–19081. doi:10.1021/jp051490q. ISSN 1520-6106. PMID 16853459. 
  22. ↑ Sung, Jaeyoung; Silbey, Robert J. (2005). "Counting statistics of single molecule reaction events and reaction dynamics of a single molecule". Chemical Physics Letters (Elsevier BV) 415 (1–3): 10–14. doi:10.1016/j.cplett.2005.08.057. ISSN 0009-2614. Bibcode: 2005CPL...415...10S. 
  23. ↑ Shaevitz, Joshua W.; Block, Steven M.; Schnitzer, Mark J. (2005). "Statistical Kinetics of Macromolecular Dynamics". Biophysical Journal (Elsevier BV) 89 (4): 2277–2285. doi:10.1529/biophysj.105.064295. ISSN 0006-3495. PMID 16040752. Bibcode: 2005BpJ....89.2277S. 
  24. ↑ Goychuk, Igor; Hänggi, Peter (2004-11-24). "Fractional diffusion modeling of ion channel gating". Physical Review E 70 (5): 051915. doi:10.1103/physreve.70.051915. ISSN 1539-3755. PMID 15600664. Bibcode: 2004PhRvE..70e1915G. 
  25. ↑ Nie, S; Chiu, D.; Zare, R. (1994-11-11). "Probing individual molecules with confocal fluorescence microscopy". Science (American Association for the Advancement of Science (AAAS)) 266 (5187): 1018–1021. doi:10.1126/science.7973650. ISSN 0036-8075. PMID 7973650. Bibcode: 1994Sci...266.1018N. 
  26. ↑ Shusterman, Roman; Alon, Sergey; Gavrinyov, Tatyana; Krichevsky, Oleg (2004-01-29). "Monomer Dynamics in Double- and Single-Stranded DNA Polymers". Physical Review Letters (American Physical Society (APS)) 92 (4): 048303. doi:10.1103/physrevlett.92.048303. ISSN 0031-9007. PMID 14995414. Bibcode: 2004PhRvL..92d8303S. 
  27. ↑ Zumofen, Gert; Hohlbein, Johannes; Hübner, Christian G. (2004-12-20). "Recurrence and Photon Statistics in Fluorescence Fluctuation Spectroscopy". Physical Review Letters (American Physical Society (APS)) 93 (26): 260601. doi:10.1103/physrevlett.93.260601. ISSN 0031-9007. PMID 15697961. Bibcode: 2004PhRvL..93z0601Z. 
  28. ↑ Cohen, A. E.; Moerner, W. E. (2006-03-14). "Suppressing Brownian motion of individual biomolecules in solution". Proceedings of the National Academy of Sciences 103 (12): 4362–4365. doi:10.1073/pnas.0509976103. ISSN 0027-8424. PMID 16537418. Bibcode: 2006PNAS..103.4362C. 
  29. ↑ Dickson, Robert M.; Cubitt, Andrew B.; Tsien, Roger Y.; Moerner, W. E. (1997). "On/off blinking and switching behaviour of single molecules of green fluorescent protein". Nature (Springer Nature) 388 (6640): 355–358. doi:10.1038/41048. ISSN 0028-0836. PMID 9237752. Bibcode: 1997Natur.388..355D. 
  30. ↑ Chung, Inhee; Bawendi, Moungi G. (2004-10-11). "Relationship between single quantum-dot intermittency and fluorescence intensity decays from collections of dots". Physical Review B (American Physical Society (APS)) 70 (16): 165304. doi:10.1103/physrevb.70.165304. ISSN 1098-0121. Bibcode: 2004PhRvB..70p5304C. 
  31. ↑ Barkai, Eli; Jung, YounJoon; Silbey, Robert (2004). "THEORY OF SINGLE-MOLECULE SPECTROSCOPY: Beyond the Ensemble Average". Annual Review of Physical Chemistry (Annual Reviews) 55 (1): 457–507. doi:10.1146/annurev.physchem.55.111803.143246. ISSN 0066-426X. PMID 15117260. Bibcode: 2004ARPC...55..457B. 
  32. ↑ Tang, Jau; Marcus, R. A. (2005-11-22). "Single particle versus ensemble average: From power-law intermittency of a single quantum dot to quasistretched exponential fluorescence decay of an ensemble". The Journal of Chemical Physics (AIP Publishing) 123 (20): 204511. doi:10.1063/1.2128409. ISSN 0021-9606. PMID 16351285. Bibcode: 2005JChPh.123t4511T. https://resolver.caltech.edu/CaltechAUTHORS:TANjcp05b. 
  33. ↑ 33.0 33.1 Flomenbom, O.; Silbey, R. J. (2008-12-15). "Toolbox for analyzing finite two-state trajectories". Physical Review E 78 (6): 066105. doi:10.1103/physreve.78.066105. ISSN 1539-3755. PMID 19256903. Bibcode: 2008PhRvE..78f6105F. 
  34. ↑ O Flomenbom, Adv. Chem. Phys., in press (2011).
  35. ↑ Horn, R.; Lange, K. (1983). "Estimating kinetic constants from single channel data". Biophysical Journal (Elsevier BV) 43 (2): 207–223. doi:10.1016/s0006-3495(83)84341-0. ISSN 0006-3495. PMID 6311301. Bibcode: 1983BpJ....43..207H. 
  36. ↑ Qin, Feng; Auerbach, Anthony; Sachs, Frederick (2000). "A Direct Optimization Approach to Hidden Markov Modeling for Single Channel Kinetics". Biophysical Journal (Elsevier BV) 79 (4): 1915–1927. doi:10.1016/s0006-3495(00)76441-1. ISSN 0006-3495. PMID 11023897. Bibcode: 2000BpJ....79.1915Q. 
  37. ↑ Bruno, W. J.; Yang, J.; Pearson, J. E. (2005-04-20). "Using independent open-to-closed transitions to simplify aggregated Markov models of ion channel gating kinetics". Proceedings of the National Academy of Sciences 102 (18): 6326–6331. doi:10.1073/pnas.0409110102. ISSN 0027-8424. PMID 15843461. Bibcode: 2005PNAS..102.6326B. 
  38. ↑ Bauer, R.J.; Bowman, B.F.; Kenyon, J.L. (1987). "Theory of the kinetic analysis of patch-clamp data". Biophysical Journal (Elsevier BV) 52 (6): 961–978. doi:10.1016/s0006-3495(87)83289-7. ISSN 0006-3495. PMID 2447973. Bibcode: 1987BpJ....52..961B. 
  39. ↑ Kienker, P. (1989-04-22). "Equivalence of Aggregated Markov Models of Ion-Channel Gating". Proceedings of the Royal Society B: Biological Sciences (The Royal Society) 236 (1284): 269–309. doi:10.1098/rspb.1989.0024. ISSN 0962-8452. PMID 2471201. Bibcode: 1989RSPSB.236..269K. 
  40. ↑ Fredkin, Donald R.; Rice, John A. (1986). "On aggregated Markov processes". Journal of Applied Probability (Cambridge University Press (CUP)) 23 (1): 208–214. doi:10.2307/3214130. ISSN 0021-9002. 
  41. ↑ Colquhoun, D.; Hawkes, A. G. (1982-12-24). "On the Stochastic Properties of Bursts of Single Ion Channel Openings and of Clusters of Bursts". Philosophical Transactions of the Royal Society B: Biological Sciences (The Royal Society) 300 (1098): 1–59. doi:10.1098/rstb.1982.0156. ISSN 0962-8436. PMID 6131450. Bibcode: 1982RSPTB.300....1C. 
  42. ↑ Song, L.; Magleby, K.L. (1994). "Testing for microscopic reversibility in the gating of maxi K+ channels using two-dimensional dwell-time distributions". Biophysical Journal (Elsevier BV) 67 (1): 91–104. doi:10.1016/s0006-3495(94)80458-8. ISSN 0006-3495. PMID 7919030. Bibcode: 1994BpJ....67...91S. 
  43. ↑ Cao, Jianshu (2000). "Event-averaged measurements of single-molecule kinetics". Chemical Physics Letters (Elsevier BV) 327 (1–2): 38–44. doi:10.1016/s0009-2614(00)00809-5. ISSN 0009-2614. Bibcode: 2000CPL...327...38C. 
  44. ↑ Vlad, M. O.; Moran, F.; Schneider, F. W.; Ross, J. (2002-09-12). "Memory effects and oscillations in single-molecule kinetics". Proceedings of the National Academy of Sciences USA (Proceedings of the National Academy of Sciences) 99 (20): 12548–12555. doi:10.1073/pnas.192439099. ISSN 0027-8424. PMID 12228729. Bibcode: 2002PNAS...9912548V. 
  45. ↑ Yang, Shilong; Cao, Jianshu (2002-12-22). "Direct measurements of memory effects in single-molecule kinetics". The Journal of Chemical Physics (AIP Publishing) 117 (24): 10996–11009. doi:10.1063/1.1521155. ISSN 0021-9606. Bibcode: 2002JChPh.11710996Y. 
  46. ↑ Šanda, F., & Mukamel, S. (2006) J. Chem. Phys. 108, 124103-1-15.
  47. ↑ Allegrini, Paolo; Aquino, Gerardo; Grigolini, Paolo; Palatella, Luigi; Rosa, Angelo (2003-11-25). "Generalized master equation via aging continuous-time random walks". Physical Review E 68 (5): 056123. doi:10.1103/physreve.68.056123. ISSN 1063-651X. PMID 14682862. Bibcode: 2003PhRvE..68e6123A. https://digital.library.unt.edu/ark:/67531/metadc67635/. 
  48. ↑ Flomenbom, Ophir; Klafter, Joseph; Szabo, Attila (2005). "What Can One Learn from Two-State Single-Molecule Trajectories?". Biophysical Journal 88 (6): 3780–3783. doi:10.1529/biophysj.104.055905. ISSN 0006-3495. PMID 15764653. Bibcode: 2005BpJ....88.3780F. 
  49. ↑ Please see, http://www.flomenbom.net/codes.html



Retrieved from "https://handwiki.org/wiki/index.php?title=Physics:Reduced_dimensions_form&oldid=3275905"