Foias constant

Evolution of the sequence [math]\displaystyle{ x_{n+1} = (1 + 1/x_n)^n }[/math] for several values of [math]\displaystyle{ x_1 }[/math], around the Foias constant [math]\displaystyle{ \alpha }[/math]. Evolution for [math]\displaystyle{ x_1 = \alpha }[/math] is in green. Other initial values lead to two accumulation points, 1 and [math]\displaystyle{ \infty }[/math]. A logarithmic scale is used.

In mathematical analysis, the Foias constant is a real number named after Ciprian Foias.

It is defined in the following way: for every real number x₁ > 0, there is a sequence defined by the recurrence relation

[math]\displaystyle{ x_{n+1} = \left( 1 + \frac{1}{x_n} \right)^n }[/math]

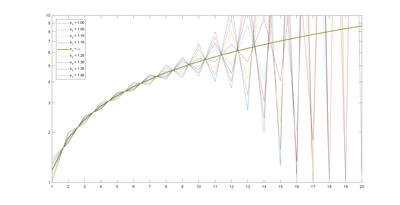
for n = 1, 2, 3, .... The Foias constant is the unique choice α such that if x₁ = α then the sequence diverges to infinity. For all other values of x₁, the sequence is divergent as well, but it has two accumulation points: 1 and infinity. Numerically, it is

[math]\displaystyle{ \alpha = 1.187452351126501\ldots }[/math].

No closed form for the constant is known.

When x₁ = α then the growth rate of the sequence (x_n) is given by the limit

[math]\displaystyle{ \lim_{n\to\infty} x_n \frac{\log n}n = 1, }[/math]

where "log" denotes the natural logarithm.

The same methods used in the proof of the uniqueness of the Foias constant may also be applied to other similar recursive sequences.

See also

• Mathematical constant

Notes and references

• S. R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 430. ISBN 0-521-818-052. https://archive.org/details/mathematicalcons0000finc. "Foias constant."