Physics:Thermal equation of state of solids

From HandWiki
Short description: none


In physics, the thermal equation of state is a mathematical expression of pressure P, temperature T, and, volume V. The thermal equation of state for ideal gases is the ideal gas law, expressed as PV=nRT (where R is the gas constant and n the amount of substance), while the thermal equation of state for solids is expressed as:
[math]\displaystyle{ P(V, T) = P(V, T_0) + P_\text{th}(V, T) }[/math] where [math]\displaystyle{ P(V, T_0) }[/math] is the volume dependence of pressure at room temperature (isothermal), and [math]\displaystyle{ P_\text{th}(V, T) }[/math] is the temperature dependence of pressure at constant volume (isochoric), known as thermal pressure.

For the ideal gas at high pressure-temperature (high P-T), the soft gas is filled in a solid firm container, and the gas is restrained inside the container; while for a solid at high P-T, a solid is loaded inside the soft medium, and the solid can expands/shrinks in the soft medium when heated and compressed. Therefore, the compression/heating process of the gas could be either constant temperature (isothermal), constant pressure (isobaric) or constant volume (isochoric). Though the compression/heating process of solids can be constant temperature (isothermal), and constant pressure (isobaric), it can not be a constant volume (isochoric),

Wiki Fig 1 Gif Cartoon.gif

At high P-T, the pressure for the ideal gas is calculated by the force divided by the area, while the pressure for the solid is calculated from bulk modulus (K, or B) and volume at room temperature, or from Eq (1) at high P-T. A pressure gauge's bulk modulus is known, and its thermal equation of state is well known. To study a solid with unknown bulk modulus, it has to be loaded with a pressure gauge, and its pressure will be determined from its pressure gauge.

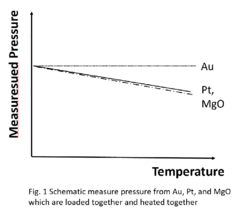
The most common pressure gauges are Au, Pt, Cu, and MgO, etc. When two or more pressure gauges are loaded together at high P-T, their pressure readings should be the same. However, large discrepancies have been reported in pressure determination using different pressure gauges or different thermal equations of state for the same pressure gauge[1] [2][3].Fig.1 is a schematic plot showing the discrepancy in paper.[4]

Out of the total pressure in Eq.(1), the first term pressures on the right side of Ag, Cu, Mo, Pd at room temperature are consistent in a wide pressure range, according to the Mao ruby scale up to 1 Mba.[5] In addition, the first term pressure of Ag, Cu, and MgO are consistent according to third-order Birch–Murnaghan equation of state.[6] Therefore, the discrepancy of the total pressure, P(V, T), should be from the second term in Eq. 1, which is the thermal pressure Pth(V, T) at high P-T.

Thermal pressure

Anderson thermal pressure model

In 1968, Anderson developed (∂T/∂P)v=(αK)-1 for the thermal gradient,[7] and its reciprocal correlate the thermal pressure and temperature in a constant volume heating process by (∂P/∂T)v=αK .[8] Note, thermal pressure is the pressure change in a constant volume heating process, and expressed by integration of αK.

Anderson thermal pressure model is the first thermal pressure model and it is the most common thermal pressure model as well.

Experimental

The thermal pressure is the pressure change in a constant volume heating. In the section above, there are large discrepancies in pressure determination using different pressure gauges or different thermal equations of state for the same pressure gauge, however, the pressure determination in the heating process need to be reliable to measure the thermal pressure in experiments. In addition, to measure the thermal pressure in experiments, the heating process has to be a volume constant (isochoric) process. According to the first section above, an heating for a solid can not be a isochoric, so the pressure change in a non-isochoric heating process is not exactly the thermal pressure.

When a solid is loaded with a pressure gauge, and heated/compressed together at high P-T, the thermal pressure of the solid does not equal that of its gauge. The pressure is a state variable, while the thermal pressure is a process variable. A solid is subject to the same pressure as its gauge, In a heating process from T1 to T2, if the solid's volume is kept constant by compression, most likely its pressure gauge's volume will not be constant in the same heating process. In paper,[9] the authors demonstrate that, αsample≠αgauge, and Ksample≠Kgauge, so

[math]\displaystyle{ \int\alpha_{\text{sample}}K_{\text{sample}}\mathrm d T\neq\int \alpha_{\text{gauge}}K_{\text{gauge}}\mathrm d T, }[/math]

which means the thermal pressure of a solid doesn't equal that of its gauge.

Determination from models

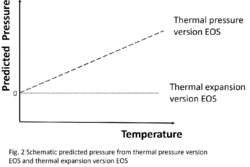
Fig. 2 thermal pressure version vs thermal expansion version.gif

According to the Anderson model, thermal pressure is the integration of the product thermal expansion αp and bulk modulus KT, i.e. Pth=∫αKdt. In this model, both αp and KT are pressure dependent and temperature dependent, so integrating the αp and KT in an isochoric process over temperature is not straight forward. To bypass this issue, the P-T dependent αp and KT are assumed to constant α0 and K0.[10][11] But authors in publication[9] demonstrated that the model predicted pressure of Au and MgO from constant α0 and K0 at ambient pressure deviate from its experimental values, and the higher temperature, the higher deviation. A cartoon plot for the pressures predicted from thermal pressure version equation of state in paper[9] is shown in Fig. 2 here.

Authors in paper[12] proposed an altenate way to make the integration of αpKT) possible. They assumes the thermal expansion to be pressure independent, and reduce the P-T dependent αp and KT to only temperature dependent. But in a preprint paper[13][unreliable source?], author proved that pressure independent thermal expansion leads to the bulk modulus to be temperature independent, which again reduce the P-T dependent αp and KT to constant α0 and K0.

There are various other thermal pressure models, but accurately determined thermal pressures are required to prove these models.

Pressure-dependent thermal expansion equation of state

It was explained that the thermal pressure can not be accurately determined in experiments in section "The thermal pressure from an experiment" above, and the thermal pressure can't accurately calculated from Anderson model above.Thermal expansion equation of state has been proposed before[14], [15], [16],[17],which consists of an thermal expansion at ambient pressure and followed by an isothermal compression at high temperature. In this model, there is no thermal pressure term, but accurate pressure determination high P-T and temperature dependent KT are of big challenge at the present. In paper,[9] the authors proposed a different thermal expansion equation of state, which consists an isothermal compression at room temperature, following by thermal expansion at high pressure. To distinguish these two thermal expansion equations of state, the latter one is called pressure-dependent thermal expansion equation of state.
To deveop the pressure-dependent thermal expansion equation of state, at room temperature, a general form of pressure in an compression process from V0 to V1 is expressed as

Wiki Fig 3 Gif-Caartoon.gif
[math]\displaystyle{ P = f_{-1} (T_0,V_1,V_0,K_0,K_0') }[/math]

The authors [9] established the relation between V1 and final volume V in an isobaric heating through the thermal expansion, and yield the general form of thermal equation of state as

[math]\displaystyle{ P=f_{-1} (T_0,V_M,V_0,K_0,K_0') }[/math]

While VM= V·exp(-∫αp·dx) in an isobaric heating process, and V is the volume after the isobaric heating. In paper,[9] authors explained in detail how to develop Eq (3), and took the third order Birch-Murnagha equation as an example to explain it.

To partially prove the pressure-dependent thermal expansion equation of state, the authors[9] collected a set of MgO x-ray diffraction data at various temperatures at ambient pressure. At ambient pressure, P=0 GPA is known, so, the volume, pressure, and temperature are all given. Then, authors[9] predict the pressure value from the given (V, T) from pressure-dependent thermal expansion equation of state. The predicted pressures match with the known experimental value of 0 GPa, see in Figure 2. In addition of MgO, the authors[9] demonstrate that the Au has a similar trend as well. In the future, the validation of the pressure-dependent thermal expansion equation of state at high P-T conditions is required.

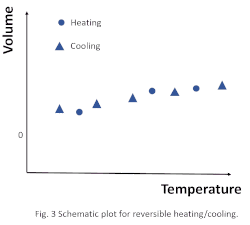
The pressure dependent αp has to be determined from an isobaric heating process. It has been reported that the heating in DAC with membrane at high P-T were isobaric. Authors in the paper[4] propose a reversible isobaric heating concept, in which the plotted heating data points and cooling data points line on the same curve. Authors[4] consider this heating and cooling process very close to the ideal isobaric. A cartoon plot of reversible heating/cooling proposed in paper[4] is shown as Fig. 3.

In paper,[4] the autors demonstrated the reversible isobaric heating concept by MgO at 9.5 GPa. In a reversible heating process, no pressure determination at high P-T is required, thus, avoid the difficulty of accurately determining the pressure at high P-T.

References

  1. ↑ Y. Fei, J. Li, K. Hirose, W. Minarik, J. V. Orman, C. Sanloup, W. V. Westrenen, T. Komabayashi, K. Funakosh, Physics of the Earth and Planetary Interiors 143-144, 515 (2004).
  2. ↑ K. Hirose, S. Karato, V.F. Cormier, J.P. Brodholt, D.A. Yuen, Geophysical Research Letters 33, L12S01 (2006).
  3. ↑ G. Fiquet, J.P. Itie, P. Richet, D. Hausermann, M. Hanfland, D. Andrault, European Journal of Mineralogy 10, 931 (1998).
  4. ↑ 4.0 4.1 4.2 4.3 4.4 Yan, Jinyuan; Yang, Shizhong (February 2022). "Pressure-Dependent Thermal Expansion Coefficient by a Diamond Anvil Cell" (in en). International Journal of Thermophysics 43 (2): 17. doi:10.1007/s10765-021-02945-3. ISSN 0195-928X. Bibcode: 2022IJT....43...17Y. https://link.springer.com/10.1007/s10765-021-02945-3. 
  5. ↑ Mao, H. K.; Bell, P. M.; Shaner, J. W.; Steinberg, D. J. (1978-06-01). "Specific volume measurements of Cu, Mo, Pd, and Ag and calibration of the ruby R 1 fluorescence pressure gauge from 0.06 to 1 Mbar" (in en). Journal of Applied Physics 49 (6): 3276–3283. doi:10.1063/1.325277. ISSN 0021-8979. Bibcode: 1978JAP....49.3276M. https://pubs.aip.org/jap/article/49/6/3276/9739/Specific-volume-measurements-of-Cu-Mo-Pd-and-Ag. 
  6. ↑ Fei, Yingwei; Ricolleau, Angele; Frank, Mark; Mibe, Kenji; Shen, Guoyin; Prakapenka, Vitali (2007-05-29). "Toward an internally consistent pressure scale" (in en). Proceedings of the National Academy of Sciences 104 (22): 9182–9186. doi:10.1073/pnas.0609013104. ISSN 0027-8424. PMID 17483460. 
  7. ↑ Anderson, Orson L.; Schreiber, Edward; Liebermann, Robert C.; Soga, Naohiro (1968). "Some elastic constant data on minerals relevant to geophysics" (in en). Reviews of Geophysics 6 (4): 491. doi:10.1029/RG006i004p00491. ISSN 8755-1209. Bibcode: 1968RvGSP...6..491A. http://doi.wiley.com/10.1029/RG006i004p00491. 
  8. ↑ Anderson, Orson L.; Isaak, Donald; Oda, Hitoshi (1992). "High-temperature elastic constant data on minerals relevant to geophysics" (in en). Reviews of Geophysics 30 (1): 57. doi:10.1029/91RG02810. ISSN 8755-1209. Bibcode: 1992RvGeo..30...57A. http://doi.wiley.com/10.1029/91RG02810. 
  9. ↑ 9.0 9.1 9.2 9.3 9.4 9.5 9.6 9.7 9.8 Yan, Jinyuan; Yang, Shizhong (2022-11-01). "Thermal Pressure in the Thermal Equation of State for Solid and a Proposed Substitute" (in en). International Journal of Thermophysics 43 (11): 169. doi:10.1007/s10765-022-03089-8. ISSN 0195-928X. Bibcode: 2022IJT....43..169Y. 
  10. ↑ Anderson, Orson L. (1995). Equations of state of solids for geophysics and ceramic science. Oxford monographs on geology and geophysics. New York: Oxford University Press. ISBN 978-0-19-505606-8. 
  11. ↑ Kroll, Herbert; Kirfel, Armin; Heinemann, Rolf; Barbier, Bruno (2012-11-16). "Volume thermal expansion and related thermophysical parameters in the Mg, Fe olivine solid-solution series" (in en). European Journal of Mineralogy 24 (6): 935–956. doi:10.1127/0935-1221/2012/0024-2235. ISSN 0935-1221. Bibcode: 2012EJMin..24..935K. http://www.schweizerbart.de/papers/ejm/detail/24/78902/Volume_thermal_expansion_and_related_thermophysica?af=crossref. 
  12. ↑ Anzellini, Simone; Errandonea, Daniel; Burakovsky, Leonid; Proctor, John E.; Turnbull, Robin; Beavers, Christine M. (2022-04-25). "Characterization of the high-pressure and high-temperature phase diagram and equation of state of chromium" (in en). Scientific Reports 12 (1): 6727. doi:10.1038/s41598-022-10523-2. ISSN 2045-2322. PMID 35468934. Bibcode: 2022NatSR..12.6727A. 
  13. ↑ Yan, Jinyuan (2023-04-25). Pressure-independent thermal expansion and temperature-dependent bulk modulus. doi:10.21203/rs.3.rs-2749229/v1. https://www.researchsquare.com/article/rs-2749229/v1. 
  14. ↑ Y. Fei, Thermal expansion, In: T. J. Ahrens (ed) Mineral Physics and Crystallography, A Handbook of Physical Constants. Am Geophys Union, Washington, DC, (1995).
  15. ↑ L. Dubrovinsky, Thermal Expansion and Equation of State, Encyclopedia of Materials: Science and Technology, Elsevier, 2002,Pages 1-4
  16. ↑ Thomas S. Duffy, Yanbin Wang; Pressure-volume-temperature equations of state. Reviews in Mineralogy and Geochemistry 1998;; 37 (1): 425–457.
  17. ↑ Angel, R.J., Alvaro, M. and Gonzalez-Platas, J., 2014. EosFit7c and a Fortran module (library) for equation of state calculations. Zeitschrift für Kristallographie-Crystalline Materials, 229(5), pp.405-419.



Retrieved from "https://handwiki.org/wiki/index.php?title=Physics:Thermal_equation_of_state_of_solids&oldid=3278467"