Coshc function

The cardinal hyperbolic cosine function coshc(z) plotted in the complex plane from -2-2i to 2+2i

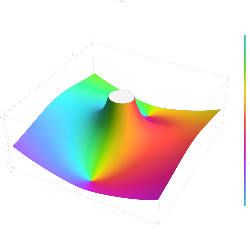
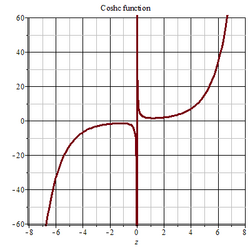
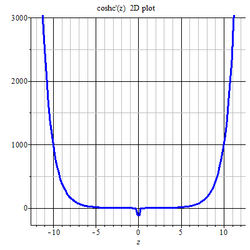
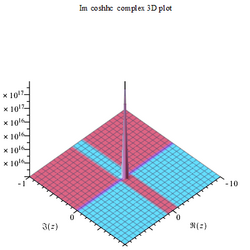
In mathematics, the coshc function appears frequently in papers about optical scattering, Heisenberg spacetime and hyperbolic geometry. For $z\ne 0$, it is defined as $$\mathrm{coshc}(z)=\frac{\cosh (z)}{z}$$

It is a solution of the following differential equation: $$w(z)z-2\frac{d}{dz}w(z)-z\frac{d^2}{d{z}^2}w(z)=0$$

Coshc 2D plot

Coshc'(z) 2D plot

Properties

The first-order derivative is given by

$\frac{\sinh (z)}{z}-\frac{\cosh (z)}{z^2}$

The Taylor series expansion is$$\mathrm{coshc}z\approx (z^{-1}+\frac{1}{2}z+\frac{1}{24}{z}^3+\frac{1}{720}{z}^5+\frac{1}{40320}{z}^7+\frac{1}{3628800}{z}^9+\frac{1}{479001600}{z}^{11}+\frac{1}{87178291200}{z}^{13}+O(z^{15}))$$

The Padé approximant is$$\mathrm{Coshc}\left(z\right)=\frac{23594700729600+11275015752000z^2+727718024880z^4+13853547000z^6+80737373z^8}{147173z^9-39328920z^7+5772800880z^5-522334612800z^3+23594700729600z}$$

In terms of other special functions

• $\mathrm{coshc}(z)=\frac{(iz+1/2\pi )\mathrm{M}(1,2,i\pi -2z)}{e^{(i/2)\pi -z}z}$, where $\mathrm{M}(a,b,z)$ is Kummer's confluent hypergeometric function.
• $\mathrm{coshc}(z)=\frac{1}{2}\frac{(2iz+\pi )\mathrm{HeunB}(2,0,0,0,\sqrt{2}\sqrt{1/2i\pi -z})}{e^{1/2i\pi -z}z}$, where $\mathrm{H}\mathrm{e}\mathrm{u}\mathrm{n}\mathrm{B}(q,\alpha ,\gamma ,\delta ,ϵ,z)$ is the biconfluent Heun function.
• $\mathrm{coshc}(z)=\frac{-i(2iz+\pi )\mathbf{𝐖}\mathrm{h}\mathrm{i}\mathrm{t}\mathrm{t}\mathrm{a}\mathrm{k}\mathrm{e}\mathrm{r}\mathrm{M}(0,1/2,i\pi -2z)}{(4iz+2\pi )z}$, where $\mathrm{W}\mathrm{h}\mathrm{i}\mathrm{t}\mathrm{t}\mathrm{a}\mathrm{k}\mathrm{e}\mathrm{r}\mathrm{M}(a,b,z)$ is a Whittaker function.

Gallery

Coshc abs complex 3D

Coshc Im complex 3D plot

Coshc Re complex 3D plot

Coshc'(z) Im complex 3D plot

Coshc'(z) Re complex 3D plot

Coshc'(z) abs complex 3D plot

Coshc'(x) abs density plot

Coshc'(x) Im density plot

Coshc'(x) Re density plot

See also

• Tanc function
• Tanhc function
• Sinhc function