Physics:Rayleigh length
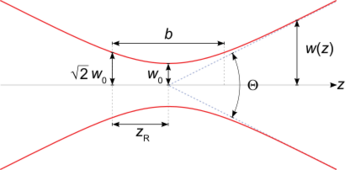
In optics and especially laser science, the Rayleigh length or Rayleigh range, [math]\displaystyle{ z_\mathrm{R} }[/math], is the distance along the propagation direction of a beam from the waist to the place where the area of the cross section is doubled.[1] A related parameter is the confocal parameter, b, which is twice the Rayleigh length.[2] The Rayleigh length is particularly important when beams are modeled as Gaussian beams.
Explanation
For a Gaussian beam propagating in free space along the [math]\displaystyle{ \hat{z} }[/math] axis with wave number [math]\displaystyle{ k = 2\pi/\lambda }[/math], the Rayleigh length is given by[2]
- [math]\displaystyle{ z_\mathrm{R} = \frac{\pi w_0^2}{\lambda} = \frac{1}{2} k w_0^2 }[/math]
where [math]\displaystyle{ \lambda }[/math] is the wavelength (the vacuum wavelength divided by [math]\displaystyle{ n }[/math], the index of refraction) and [math]\displaystyle{ w_0 }[/math] is the beam waist, the radial size of the beam at its narrowest point. This equation and those that follow assume that the waist is not extraordinarily small; [math]\displaystyle{ w_0 \ge 2\lambda/\pi }[/math].[3]
The radius of the beam at a distance [math]\displaystyle{ z }[/math] from the waist is[4]
- [math]\displaystyle{ w(z) = w_0 \, \sqrt{ 1+ {\left( \frac{z}{z_\mathrm{R}} \right)}^2 } . }[/math]
The minimum value of [math]\displaystyle{ w(z) }[/math] occurs at [math]\displaystyle{ w(0) = w_0 }[/math], by definition. At distance [math]\displaystyle{ z_\mathrm{R} }[/math] from the beam waist, the beam radius is increased by a factor [math]\displaystyle{ \sqrt{2} }[/math] and the cross sectional area by 2.
Related quantities
The total angular spread of a Gaussian beam in radians is related to the Rayleigh length by[1]
- [math]\displaystyle{ \Theta_{\mathrm{div}} \simeq 2\frac{w_0}{z_R}. }[/math]
The diameter of the beam at its waist (focus spot size) is given by
- [math]\displaystyle{ D = 2\,w_0 \simeq \frac{4\lambda}{\pi\, \Theta_{\mathrm{div}}} }[/math].
These equations are valid within the limits of the paraxial approximation. For beams with much larger divergence the Gaussian beam model is no longer accurate and a physical optics analysis is required.
See also
- Beam divergence
- Beam parameter product
- Gaussian function
- Electromagnetic wave equation
- John Strutt, 3rd Baron Rayleigh
- Robert Strutt, 4th Baron Rayleigh
- Depth of field
References
- ↑ 1.0 1.1 Siegman, A. E. (1986). Lasers. University Science Books. pp. 664–669. ISBN 0-935702-11-3. https://archive.org/details/lasers0000sieg.
- ↑ 2.0 2.1 Damask, Jay N. (2004). Polarization Optics in Telecommunications. Springer. pp. 221–223. ISBN 0-387-22493-9. https://archive.org/details/polarizationopti00dama.
- ↑ Siegman (1986) p. 630.
- ↑ Meschede, Dieter (2007). Optics, Light and Lasers: The Practical Approach to Modern Aspects of Photonics and Laser Physics. Wiley-VCH. pp. 46–48. ISBN 978-3-527-40628-9. https://archive.org/details/opticslightlaser00mesc_263.
- Rayleigh length RP Photonics Encyclopedia of Optics
 |

