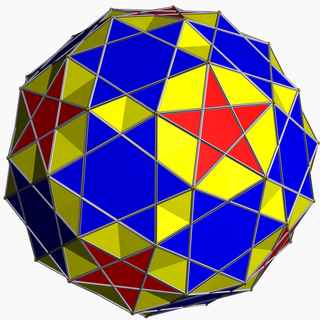
Small snub icosicosidodecahedron
| Small snub icosicosidodecahedron | |
|---|---|
| |
| Type | Uniform star polyhedron |
| Elements | F = 112, E = 180 V = 60 (χ = −8) |
| Faces by sides | (40+60){3}+12{5/2} |
| Wythoff symbol | | 5/2 3 3 |
| Symmetry group | Ih, [5,3], *532 |
| Index references | U32, C41, W110 |
| Dual polyhedron | Small hexagonal hexecontahedron |
| Vertex figure |  35.5/2 |
| Bowers acronym | Seside |
File:Small snub icosicosidododecahedron.stl
In geometry, the small snub icosicosidodecahedron or snub disicosidodecahedron is a uniform star polyhedron, indexed as U32. It has 112 faces (100 triangles and 12 pentagrams), 180 edges, and 60 vertices. Its stellation core is a truncated pentakis dodecahedron. It also called a holosnub icosahedron, ß{3,5}.
The 40 non-snub triangular faces form 20 coplanar pairs, forming star hexagons that are not quite regular. Unlike most snub polyhedra, it has reflection symmetries.
Convex hull
Its convex hull is a nonuniform truncated icosahedron.
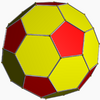 Truncated icosahedron (regular faces) |
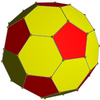 Convex hull (isogonal hexagons) |
 Small snub icosicosidodecahedron |
Cartesian coordinates
Cartesian coordinates for the vertices of a small snub icosicosidodecahedron are all the even permutations of [math]\displaystyle{ \begin{array}{clllc} \Bigl(& \pm \bigl[1-\varphi+\alpha\bigr],& \ \ \ \, 0,& \pm \bigl[3+\varphi\alpha\bigr] &\Bigr) \\ \Bigl(& \pm \bigl[\varphi-1+\alpha\bigr],& \pm\,2,& \pm \bigl[2\varphi-1+\varphi\alpha\bigr] &\Bigr) \\ \Bigl(& \pm \bigl[\varphi+1+\alpha\bigr],& \pm\,2\bigl[\varphi-1\bigr],& \pm \bigl[1+\varphi\alpha\bigr] &\Bigr) \end{array} }[/math]
where [math]\displaystyle{ \varphi = \tfrac{1+ \sqrt 5}{2} }[/math] is the golden ratio and [math]\displaystyle{ \alpha = \sqrt{3\varphi - 2}. }[/math]
See also
External links
- Weisstein, Eric W.. "Small snub icosicosidodecahedron". http://mathworld.wolfram.com/SmallSnubIcosicosidodecahedron.html.
- Klitzing, Richard. "3D star small snub icosicosidodecahedron". https://bendwavy.org/klitzing/dimensions/../incmats/seside.htm.
 |

