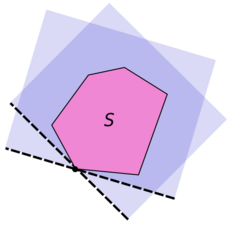
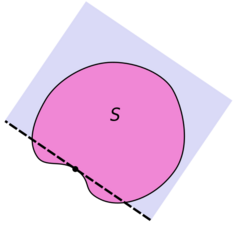
Supporting hyperplane

In geometry, a supporting hyperplane of a set [math]\displaystyle{ S }[/math] in Euclidean space [math]\displaystyle{ \mathbb R^n }[/math] is a hyperplane that has both of the following two properties:[1]
- [math]\displaystyle{ S }[/math] is entirely contained in one of the two closed half-spaces bounded by the hyperplane,
- [math]\displaystyle{ S }[/math] has at least one boundary-point on the hyperplane.
Here, a closed half-space is the half-space that includes the points within the hyperplane.
Supporting hyperplane theorem
This theorem states that if [math]\displaystyle{ S }[/math] is a convex set in the topological vector space [math]\displaystyle{ X=\mathbb{R}^n, }[/math] and [math]\displaystyle{ x_0 }[/math] is a point on the boundary of [math]\displaystyle{ S, }[/math] then there exists a supporting hyperplane containing [math]\displaystyle{ x_0. }[/math] If [math]\displaystyle{ x^* \in X^* \backslash \{0\} }[/math] ([math]\displaystyle{ X^* }[/math] is the dual space of [math]\displaystyle{ X }[/math], [math]\displaystyle{ x^* }[/math] is a nonzero linear functional) such that [math]\displaystyle{ x^*\left(x_0\right) \geq x^*(x) }[/math] for all [math]\displaystyle{ x \in S }[/math], then
- [math]\displaystyle{ H = \{x \in X: x^*(x) = x^*\left(x_0\right)\} }[/math]
defines a supporting hyperplane.[2]
Conversely, if [math]\displaystyle{ S }[/math] is a closed set with nonempty interior such that every point on the boundary has a supporting hyperplane, then [math]\displaystyle{ S }[/math] is a convex set, and is the intersection of all its supporting closed half-spaces.[2]
The hyperplane in the theorem may not be unique, as noticed in the second picture on the right. If the closed set [math]\displaystyle{ S }[/math] is not convex, the statement of the theorem is not true at all points on the boundary of [math]\displaystyle{ S, }[/math] as illustrated in the third picture on the right.
The supporting hyperplanes of convex sets are also called tac-planes or tac-hyperplanes.[3]
The forward direction can be proved as a special case of the separating hyperplane theorem (see the page for the proof). For the converse direction,
Define [math]\displaystyle{ T }[/math] to be the intersection of all its supporting closed half-spaces. Clearly [math]\displaystyle{ S \subset T }[/math]. Now let [math]\displaystyle{ y\not \in S }[/math], show [math]\displaystyle{ y \not\in T }[/math].
Let [math]\displaystyle{ x\in \mathrm{int}(S) }[/math], and consider the line segment [math]\displaystyle{ [x, y] }[/math]. Let [math]\displaystyle{ t }[/math] be the largest number such that [math]\displaystyle{ [x, t(y-x) + x] }[/math] is contained in [math]\displaystyle{ S }[/math]. Then [math]\displaystyle{ t\in (0, 1) }[/math].
Let [math]\displaystyle{ b = t(y-x) + x }[/math], then [math]\displaystyle{ b\in \partial S }[/math]. Draw a supporting hyperplane across [math]\displaystyle{ b }[/math]. Let it be represented as a nonzero linear functional [math]\displaystyle{ f: \R^n \to \R }[/math] such that [math]\displaystyle{ \forall a\in S, f(a) \geq f(b) }[/math]. Then since [math]\displaystyle{ x\in \mathrm{int}(S) }[/math], we have [math]\displaystyle{ f(x) \gt f(b) }[/math]. Thus by [math]\displaystyle{ \frac{f(y) - f(b)}{1-t} = \frac{f(b) - f(x)}{t} }[/math], we have [math]\displaystyle{ f(y) \lt f(b) }[/math], so [math]\displaystyle{ y \not\in T }[/math].
See also
- Support function
- Supporting line (supporting hyperplanes in [math]\displaystyle{ \mathbb{R}^2 }[/math])
Notes
- ↑ Luenberger, David G. (1969). Optimization by Vector Space Methods. New York: John Wiley & Sons. p. 133. ISBN 978-0-471-18117-0. https://books.google.com/books?id=lZU0CAH4RccC&pg=PA133.
- ↑ Jump up to: 2.0 2.1 Boyd, Stephen P.; Vandenberghe, Lieven (2004) (pdf). Convex Optimization. Cambridge University Press. pp. 50–51. ISBN 978-0-521-83378-3. https://web.stanford.edu/~boyd/cvxbook/bv_cvxbook.pdf#page=64. Retrieved October 15, 2011.
- ↑ Cassels, John W. S. (1997), An Introduction to the Geometry of Numbers, Springer Classics in Mathematics (reprint of 1959[3] and 1971 Springer-Verlag ed.), Springer-Verlag.
References & further reading
- Ostaszewski, Adam (1990). Advanced mathematical methods. Cambridge; New York: Cambridge University Press. p. 129. ISBN 0-521-28964-5. https://archive.org/details/advancedmathemat0000osta.
- Giaquinta, Mariano; Hildebrandt, Stefan (1996). Calculus of variations. Berlin; New York: Springer. p. 57. ISBN 3-540-50625-X.
- Goh, C. J.; Yang, X.Q. (2002). Duality in optimization and variational inequalities. London; New York: Taylor & Francis. p. 13. ISBN 0-415-27479-6.
- Soltan, V. (2021). Support and separation properties of convex sets in finite dimension. Extracta Math. Vol. 36, no. 2, 241-278.
 |