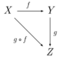Amnestic functor

In the mathematical field of category theory, an amnestic functor F : A → B is a functor for which an A-isomorphism ƒ is an identity whenever Fƒ is an identity. An example of a functor which is not amnestic is the forgetful functor Met_c→Top from the category of metric spaces with continuous functions for morphisms to the category of topological spaces. If [math]\displaystyle{ d_1 }[/math] and [math]\displaystyle{ d_2 }[/math] are equivalent metrics on a space [math]\displaystyle{ X }[/math] then [math]\displaystyle{ \operatorname{id}\colon(X, d_1)\to(X, d_2) }[/math] is an isomorphism that covers the identity, but is not an identity morphism (its domain and codomain are not equal).