Nonconvex great rhombicosidodecahedron
| Nonconvex great rhombicosidodecahedron | |
|---|---|
| |
| Type | Uniform star polyhedron |
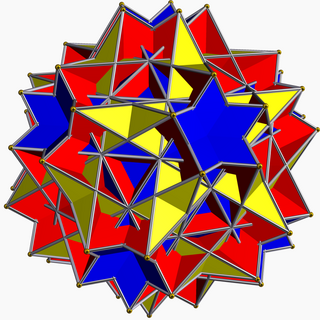
| Elements | F = 62, E = 120 V = 60 (χ = 2) |
| Faces by sides | 20{3}+30{4}+12{5/2} |
| Wythoff symbol | 5/3 3 | 2 5/2 3/2 | 2 |
| Symmetry group | Ih, [5,3], *532 |
| Index references | U67, C84, W105 |
| Dual polyhedron | Great deltoidal hexecontahedron |
| Vertex figure |  3.4.5/3.4 |
| Bowers acronym | Qrid |
File:Nonconvex great rhombicosidodecahedron.stl In geometry, the nonconvex great rhombicosidodecahedron is a nonconvex uniform polyhedron, indexed as U67. It has 62 faces (20 triangles, 30 squares and 12 pentagrams), 120 edges, and 60 vertices.[1] It is also called the quasirhombicosidodecahedron. It is given a Schläfli symbol rr{5⁄3,3}. Its vertex figure is a crossed quadrilateral.
This model shares the name with the convex great rhombicosidodecahedron, also known as the truncated icosidodecahedron.
Cartesian coordinates
Cartesian coordinates for the vertices of a nonconvex great rhombicosidodecahedron are all the even permutations of [math]\displaystyle{ \begin{array}{ccclc} \Bigl(& \pm\,\frac{1}{\varphi^2},& 0,& \pm \bigl[2-\frac{1}{\varphi}\bigr] &\Bigr) \\ \Bigl(& \pm\,1,& \pm\,\frac{1}{\varphi^3},& \pm\,1 &\Bigr) \\ \Bigl(& \pm\,\frac{1}{\varphi},& \pm\,\frac{1}{\varphi^2},& \pm\,\frac{2}{\varphi} &\Bigr) \end{array} }[/math]
where [math]\displaystyle{ \varphi = \tfrac{1+\sqrt 5}{2} }[/math] is the golden ratio.
Related polyhedra
It shares its vertex arrangement with the truncated great dodecahedron, and with the uniform compounds of 6 or 12 pentagonal prisms. It additionally shares its edge arrangement with the great dodecicosidodecahedron (having the triangular and pentagrammic faces in common), and the great rhombidodecahedron (having the square faces in common).
 Nonconvex great rhombicosidodecahedron |
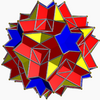 Great dodecicosidodecahedron |
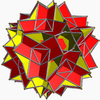 Great rhombidodecahedron |
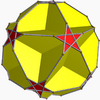 Truncated great dodecahedron |
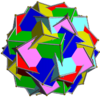 Compound of six pentagonal prisms |
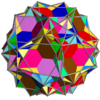 Compound of twelve pentagonal prisms |
Great deltoidal hexecontahedron
| Great deltoidal hexecontahedron | |
|---|---|

| |
| Type | Star polyhedron |
| Face | 
|
| Elements | F = 60, E = 120 V = 62 (χ = 2) |
| Symmetry group | Ih, [5,3], *532 |
| Index references | DU67 |
| dual polyhedron | Nonconvex great rhombicosidodecahedron |
File:Great deltoidal hexecontahedron.stl The great deltoidal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the nonconvex great rhombicosidodecahedron. It is visually identical to the great rhombidodecacron. It has 60 intersecting cross quadrilateral faces, 120 edges, and 62 vertices.
It is also called a great strombic hexecontahedron.
See also
References
- Wenninger, Magnus (1983), Dual Models, Cambridge University Press, doi:10.1017/CBO9780511569371, ISBN 978-0-521-54325-5
External links
- Weisstein, Eric W.. "Great rhombicosidodecahedron". http://mathworld.wolfram.com/UniformGreatRhombicosidodecahedron.html.
- Weisstein, Eric W.. "Great deltoidal hexecontahedron". http://mathworld.wolfram.com/GreatDeltoidalHexecontahedron.html.
- Uniform polyhedra and duals
 |

