Physics:Harmonic generation
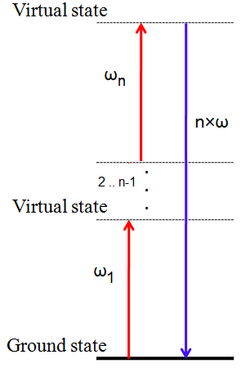
Harmonic generation (HG, also called multiple harmonic generation) is a nonlinear optical process in which [math]\displaystyle{ n }[/math] photons with the same frequency interact with a nonlinear material, are "combined", and generate a new photon with [math]\displaystyle{ n }[/math] times the energy of the initial photons (equivalently, [math]\displaystyle{ n }[/math] times the frequency and the wavelength divided by [math]\displaystyle{ n }[/math]).
General process
In a medium having a substantial nonlinear susceptibility, harmonic generation is possible. Note that for even orders ([math]\displaystyle{ n = 2,4,\dots }[/math]), the medium must have no center of symmetry (non-centrosymmetrical).[1]
Because the process requires that many photons are present at the same time and at the same place, the generation process has a low probability to occur, and this probability decreases with the order [math]\displaystyle{ n }[/math]. To generate efficiently, the symmetry of the medium must allow the signal to be amplified (through phase matching, for instance), and the light source must be intense and well-controlled spatially (with a collimated laser) and temporally (more signal if the laser has short pulses).[2]
Sum-frequency generation (SFG)
A special case in which the number of photons in the interaction is [math]\displaystyle{ n = 2 }[/math], but with two different photons at frequencies [math]\displaystyle{ \omega_1 }[/math] and [math]\displaystyle{ \omega_2 }[/math].
Second-harmonic generation (SHG)
A special case in which the number of photons in the interaction is [math]\displaystyle{ n = 2 }[/math]. Also a special case of sum-frequency generation in which both photons are at the same frequency [math]\displaystyle{ \omega }[/math].
Third-harmonic generation (THG)
A special case in which the number of photons in the interaction is [math]\displaystyle{ n = 3 }[/math], if all the photons have the same frequency [math]\displaystyle{ \omega }[/math]. If they have different frequency, the general term of four-wave mixing is preferred. This process involves the 3rd order nonlinear susceptibility [math]\displaystyle{ \chi^{(3)} }[/math].[3]
Unlike SHG, it is a volumetric process[4] and has been shown in liquids.[5] However, it is enhanced at interfaces.[6]
Materials used for THG
Nonlinear crystals such as BBO (β-BaB2O4) or LBO can convert THG, otherwise THG can be generated from membranes in microscopy.[7]
Fourth-harmonic generation (FHG or 4HG)
A special case in which the number of photons in interaction is [math]\displaystyle{ n = 4 }[/math]. Reported around the year 2000,[8] powerful lasers now enable efficient FHG. This process involves the 4th order nonlinear susceptibility [math]\displaystyle{ \chi^{(4)} }[/math].
Materials used for FHG
Some BBO (β-BaB2O4) are used for FHG.[9]
Harmonic generation for [math]\displaystyle{ n \gt 4 }[/math]
Harmonic generation for [math]\displaystyle{ n = 5 }[/math] (5HG) or more is theoretically possible, but the interaction requires a very high number of photons to interact and has therefore a low probability to happen: the signal at higher harmonics will be very low, and requires very intense lasers to be generated. To generate high harmonics (like [math]\displaystyle{ n = 30 }[/math] and so on), the substantially different process of high harmonic generation can be used.
Sources
- Boyd, R.W. (2007) (in en). Nonlinear optics (third ed.). ISBN 9780123694706. https://books.google.com/books?id=uoRUi1Yb7ooC&q=Nonlinear+optics.
- Sutherland, Richard L. (2003) (in en). Handbook of Nonlinear Optics (2nd ed.). ISBN 9780824742430. https://books.google.com/books?id=ccXo3WrHp2UC&q=Handbook+of+Nonlinear+Optics.
- Hecht, Eugene (2002) (in en). Optics (4th ed.). Addison-Wesley. ISBN 978-0805385663.
- Zernike, Frits; Midwinter, John E. (2006) (in en). Applied Nonlinear Optics. Dover Publications. ISBN 978-0486453606. https://books.google.com/books?id=Y3Wsyo7TgdkC&q=Applied+Nonlinear+Optics.
See also
References
- ↑ Boyd, R. (2007). "The Nonlinear Optical Susceptibility" (in en). Nonlinear optics (third ed.). pp. 1–67. doi:10.1016/B978-0-12-369470-6.00001-0. ISBN 9780123694706. https://archive.org/details/nonlinearopticst00boyd.
- ↑ Sutherland, Richard L. (2003) (in en). Handbook of Nonlinear Optics (2nd ed.). ISBN 9780824742430.
- ↑ Boyd, R.W. (2007) (in en). Nonlinear optics (third ed.). ISBN 9780123694706. https://books.google.com/books?id=uoRUi1Yb7ooC&q=Nonlinear+optics.
- ↑ Moreaux, Laurent; Sandre, Olivier; Charpak, Serge; Blanchard-Desce, Mireille; Mertz, Jerome (2001). "Coherent Scattering in Multi-Harmonic Light Microscopy". Biophysical Journal 80 (3): 1568–1574. doi:10.1016/S0006-3495(01)76129-2. ISSN 0006-3495. PMID 11222317. Bibcode: 2001BpJ....80.1568M.
- ↑ Kajzar, F.; Messier, J. (1985). "Third-harmonic generation in liquids". Physical Review A 32 (4): 2352–2363. doi:10.1103/PhysRevA.32.2352. ISSN 0556-2791. PMID 9896350. Bibcode: 1985PhRvA..32.2352K.
- ↑ Cheng, Ji-Xin; Xie, X. Sunney (2002). "Green's function formulation for third-harmonic generation microscopy". Journal of the Optical Society of America B 19 (7): 1604. doi:10.1364/JOSAB.19.001604. ISSN 0740-3224. Bibcode: 2002JOSAB..19.1604C.
- ↑ Pavone, Francesco S.; Campagnola, Paul J. (2016). Second Harmonic Generation Imaging, 2nd edition. CRC Taylor&Francis. ISBN 978-1-4398-4914-9. https://books.google.com/books?id=EiTOBQAAQBAJ&q=Second+Harmonic+Generation+Imaging+Pavone+Francesco.
- ↑ Kojima, Tetsuo; Konno, Susumu; Fujikawa, Shuichi; Yasui, Koji; Yoshizawa, Kenji; Mori, Yusuke; Sasaki, Takatomo; Tanaka, Mitsuhiro et al. (2000). "20-W ultraviolet-beam generation by fourth-harmonic generation of an all-solid-state laser". Optics Letters 25 (1): 58–60. doi:10.1364/OL.25.000058. ISSN 0146-9592. PMID 18059781. Bibcode: 2000OptL...25...58K.
- ↑ "BBO for FHG". http://raicol.com/bbo/bbo-for-fhg.
 |