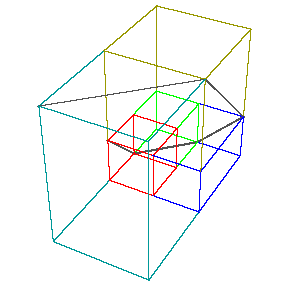
Padovan cuboid spiral
In mathematics the Padovan cuboid spiral is the spiral created by joining the diagonals of faces of successive cuboids added to a unit cube. The cuboids are added sequentially so that the resulting cuboid has dimensions that are successive Padovan numbers.[1][2][3]
The first cuboid is 1x1x1. The second is formed by adding to this a 1x1x1 cuboid to form a 1x1x2 cuboid. To this is added a 1x1x2 cuboid to form a 1x2x2 cuboid. This pattern continues, forming in succession a 2x2x3 cuboid, a 2x3x4 cuboid etc.[1][2][3] Joining the diagonals of the exposed end of each new added cuboid creates a spiral (seen as the black line in the figure). The points on this spiral all lie in the same plane.[1]
The cuboids are added in a sequence that adds to the face in the positive y direction, then the positive x direction, then the positive z direction. This is followed by cuboids added in the negative y, negative x and negative z directions. Each new cuboid added has a length and width that matches the length and width of the face being added to. The height of the nth added cuboid is the nth Padovan number.[1][3]
Connecting alternate points where the spiral bends creates a series of triangles, where each triangle has two sides that are successive Padovan numbers and that has an obtuse angle of 120 degrees between these two sides.
References
- ↑ 1.0 1.1 1.2 1.3 Darling, David (2004), The Universal Book of Mathematics: From Abracadabra to Zeno's Paradoxes, John Wiley & Sons, p. 245, ISBN 9780471270478, https://books.google.com/books?id=nnpChqstvg0C&pg=PA245.
- ↑ 2.0 2.1 Sharp, John (2000), "Beyond the Golden Section – the Golden tip of the iceberg", Bridges: Mathematical Connections in Art, Music, and Science, pp. 87–98, http://archive.bridgesmathart.org/2000/bridges2000-87.pdf. See in particular pp. 96–97.
- ↑ 3.0 3.1 3.2 Stewart, Ian (2004), Math Hysteria: Fun and games with mathematics, Oxford University Press, p. 73, ISBN 9780191647451, https://books.google.com/books?id=xnViTAYeblkC&pg=PT73.
External links
- Padovan Spiral Numbers, Robert Dickau, Wolfram Demonstrations Project
 |