Cuboid

Short description: Convex polyhedron with six sides with four edges each

In geometry, a cuboid is a hexahedron, a six-faced solid. Its faces are quadrilaterals. Cuboid means "like a cube", in the sense that by adjusting the lengths of the edges or the angles between faces, a cuboid can be transformed into a cube. In mathematical language a cuboid is a convex polyhedron whose polyhedral graph is the same as that of a cube.

A special case of a cuboid is a rectangular cuboid, with six rectangles as faces and adjacent faces meeting at right angles. A cube is a special case of a rectangular cuboid, with six square faces meeting at right angles.^[1]^[2]

By Euler's formula the numbers of faces $F$ , of vertices $V$ , and of edges $E$ of any convex polyhedron are related by the formula [math]\displaystyle{ F + V - E = 2. }[/math] In the case of a cuboid this gives 6 + 8 – 12 = 2; that is, like a cube, a cuboid has six faces, eight vertices, and twelve edges. Along with the rectangular cuboids, any parallelepiped is a cuboid of this type, as is a square frustum (the shape formed by truncation of the apex of a square pyramid).

Quadrilaterally-faced hexahedron (cuboid) 6 faces, 12 edges, 8 vertices

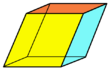
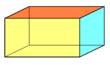
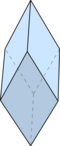
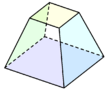
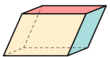
Cube (square)	Rectangular cuboid (three pairs of rectangles)	Trigonal trapezohedron (congruent rhombi)	Trigonal trapezohedron (congruent quadrilaterals)	Quadrilateral frustum (apex-truncated square pyramid)	Parallelepiped (three pairs of parallelograms)	Rhombohedron (three pairs of rhombi)
$O h, [4,3], (*432)$ order 48	$D 2h, [2,2], (*222)$ order 8	$D 3 d, [2 +,6], (2*3)$ order 12	$D 3, [2,3] +, (223)$ order 6	$C 4v, [4], (*44)$ order 8	$C i, [2 +,2 +], (\times)$ order 2