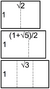
Gauss's constant

From HandWiki
Revision as of 06:24, 18 May 2023 by Scavis (talk | contribs) (add)
(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)

User:RMCD bot/subject notice

Lemniscate of Bernoulli

In mathematics, the lemniscate constant ϖ is a transcendental mathematical constant that is the ratio of the perimeter of Bernoulli's lemniscate to its diameter, analogous to the definition of π for the circle. It is approximately equal to 2.62205755.[1][2][3]

Gauss's constant, denoted by G, is equal to ϖ /π ≈ 0.8346268.

John Todd named two more lemniscate constants, the first lemniscate constant L = ϖ/2 ≈ 1.3110287771 and the second lemniscate constant M = π/(2ϖ) ≈ 0.5990701173.[4][5][6]

Sometimes the quantities 2ϖ or L are referred to as the lemniscate constant.[7][8] Throughout this article, we strictly follow Gauss' definition for the lemniscate constant.

History

Gauss's constant is named after Carl Friedrich Gauss, who calculated it via the arithmetic–geometric mean as [math]\displaystyle{ 1/M(1,\sqrt{2}) }[/math].[1] By 1799, Gauss had two proofs of the theorem that [math]\displaystyle{ M(1,\sqrt{2})=\frac{\pi}{\varpi} }[/math] where [math]\displaystyle{ \varpi }[/math] is the lemniscate constant.[lower-alpha 1]

The lemniscate constant and first lemniscate constant [math]\displaystyle{ L }[/math] were proven transcendental by Theodor Schneider in 1937 and the second lemniscate constant [math]\displaystyle{ M }[/math]and Gauss's constant [math]\displaystyle{ G }[/math] were proven transcendental by Theodor Schneider in 1941.[4][9][lower-alpha 2] In 1975, Gregory Chudnovsky proved that the set [math]\displaystyle{ \{\pi,\varpi\} }[/math] is algebraically independent over [math]\displaystyle{ \mathbb{Q} }[/math], which implies that [math]\displaystyle{ L }[/math] and [math]\displaystyle{ M }[/math] are algebraically independent as well.[10][11] But the set [math]\displaystyle{ \{\pi,M(1,1/\sqrt{2}),M'(1,1/\sqrt{2})\} }[/math] (where the prime denotes the derivative with respect to the second variable) is not algebraically independent over [math]\displaystyle{ \mathbb{Q} }[/math]. In fact,[12]

[math]\displaystyle{ \pi=2\sqrt{2}\frac{M^3(1,1/\sqrt{2})}{M'(1,1/\sqrt{2})}=\frac{1}{G^3 M'(1,1/\sqrt{2})}. }[/math]

Forms

Usually, [math]\displaystyle{ \varpi }[/math] is defined by the first equality below.

[math]\displaystyle{ \begin{aligned} \varpi &= 2\int_0^1\frac{\mathrm{d}t}{\sqrt{1-t^4}} = \sqrt2\int_0^\infty\frac{\mathrm{d}t}{\sqrt{1+t^4}} = \int_0^1\frac{\mathrm dt}{\sqrt{t-t^3}} \\[6mu] &= 4\int_0^\infty\Bigl(\sqrt[4]{1+t^{4}}-t\Bigr)\,\mathrm{d}t = 2\sqrt2\int_0^1 \sqrt[4]{1-t^{4}}\mathop{\mathrm{d}t} =3\int_0^1 \sqrt{1-t^4}\,\mathrm dt\\[2mu] &= 2K(i) = \tfrac{1}{2}\Beta\bigl( \tfrac14, \tfrac12\bigr) = \frac{\Gamma (1/4)^2}{2\sqrt{2\pi}} = \sqrt{\pi}e^{\beta '(0)} = \frac{2-\sqrt{2}}{4}\frac{\zeta(3/4)^2}{\zeta(1/4)^2}\\[5mu] &= 2.62205\;75542\;92119\;81046\;48395\;89891\;11941\ldots, \end{aligned} }[/math]

where K is the complete elliptic integral of the first kind with modulus k, Β is the beta function, Γ is the gamma function, β' is the derivative of the Dirichlet beta function and ζ is the Riemann zeta function.

The lemniscate constant can also be computed by the arithmetic–geometric mean [math]\displaystyle{ \operatorname{M} }[/math],

[math]\displaystyle{ \varpi=\frac{\pi}{\operatorname{M}(1,\sqrt{2})}. }[/math]

Gauss's constant is typically defined as the reciprocal of the arithmetic–geometric mean of 1 and the square root of 2, after his calculation of [math]\displaystyle{ \operatorname{M}\left(1, \sqrt{2}\right) }[/math] published in 1800:[13]

[math]\displaystyle{ G = \frac{1}{\operatorname{M}\left(1, \sqrt{2}\right)} }[/math]

Gauss's constant is equal to

[math]\displaystyle{ G = \frac{1}{2\pi}\Beta\bigl( \tfrac14, \tfrac12\bigr) }[/math]

where Β denotes the beta function. A formula for G in terms of Jacobi theta functions is given by

[math]\displaystyle{ G = \vartheta_{01}^2\left(e^{-\pi}\right) }[/math]

Gauss's constant may be computed from the gamma function at argument 1/4:

[math]\displaystyle{ G = \frac{\Gamma\bigl( \tfrac{1}{4}\bigr){}^2}{2\sqrt{ 2\pi^3}} }[/math]

John Todd's lemniscate constants may be given in terms of the beta function B: [math]\displaystyle{ \begin{aligned} L &= \tfrac12\pi G = \tfrac12\varpi = \tfrac14 \Beta \bigl(\tfrac14,\tfrac12\bigr), \\[3mu] M &= \frac{1}{2G} =\tfrac14\Beta \bigl(\tfrac12,\tfrac34\bigr). \end{aligned} }[/math]

Series

Viète's formula for π can be written:

[math]\displaystyle{ \frac2\pi = \sqrt\frac12 \cdot \sqrt{\frac12 + \frac12\sqrt\frac12} \cdot \sqrt{\frac12 + \frac12\sqrt{\frac12 + \frac12\sqrt\frac12}} \cdots }[/math]

An analogous formula for ϖ is:[14]

[math]\displaystyle{ \frac2\varpi = \sqrt\frac12 \cdot \sqrt{\frac12 + \frac12 \bigg/ \!\sqrt\frac12} \cdot \sqrt{\frac12 + \frac12 \Bigg/ \!\sqrt{\frac12 + \frac12 \bigg/ \!\sqrt\frac12}} \cdots }[/math]

The Wallis product for π is:

[math]\displaystyle{ \frac{\pi}{2} = \prod_{n=1}^\infty \left(1+\frac{1}{n}\right)^{(-1)^{n+1}}=\prod_{n=1}^{\infty} \left(\frac{2n}{2n-1} \cdot \frac{2n}{2n+1}\right) = \biggl(\frac{2}{1} \cdot \frac{2}{3}\biggr) \biggl(\frac{4}{3} \cdot \frac{4}{5}\biggr) \biggl(\frac{6}{5} \cdot \frac{6}{7}\biggr) \cdots }[/math]

An analogous formula for ϖ is:[15]

[math]\displaystyle{ \frac{\varpi}{2} = \prod_{n=1}^\infty \left(1+\frac{1}{2n}\right)^{(-1)^{n+1}}=\prod_{n=1}^{\infty} \left(\frac{4n-1}{4n-2} \cdot \frac{4n}{4n+1}\right) = \biggl(\frac{3}{2} \cdot \frac{4}{5}\biggr) \biggl(\frac{7}{6} \cdot \frac{8}{9}\biggr) \biggl(\frac{11}{10} \cdot \frac{12}{13}\biggr) \cdots }[/math]

A related result for Gauss's constant ([math]\displaystyle{ G=\varpi / \pi }[/math]) is:

[math]\displaystyle{ G = \prod_{n=1}^{\infty} \left(\frac{4n-1}{4n} \cdot \frac{4n+2}{4n+1}\right) = \biggl(\frac{3}{4} \cdot \frac{6}{5}\biggr) \biggl(\frac{7}{8} \cdot \frac{10}{9}\biggr) \biggl(\frac{11}{12} \cdot \frac{14}{13}\biggr) \cdots }[/math]

An infinite series of Gauss's constant discovered by Gauss is:[16]

[math]\displaystyle{ G = \sum_{n=0}^\infty (-1)^n \prod_{k=1}^n \frac{(2k-1)^2}{(2k)^2} = 1 - \frac{1^2}{2^2} + \frac{1^2\cdot3^2}{2^2\cdot4^2} - \frac{1^2\cdot3^2\cdot5^2}{2^2\cdot4^2\cdot6^2} + \cdots }[/math]

The Machin formula for π is [math]\displaystyle{ \tfrac14\pi = 4 \arctan \tfrac15 - \arctan \tfrac1{239}, }[/math] and several similar formulas for π can be developed using trigonometric angle sum identities, e.g. Euler's formula [math]\displaystyle{ \tfrac14\pi = \arctan\tfrac12 + \arctan\tfrac13 }[/math]. Analogous formulas can be developed for ϖ, including the following found by Gauss: [math]\displaystyle{ \tfrac12\varpi = 2 \operatorname{arcsl} \tfrac12 + \operatorname{arcsl} \tfrac7{23} }[/math], where [math]\displaystyle{ \operatorname{arcsl} }[/math] is the lemniscate arcsine.[17]

The lemniscate constant can be rapidly computed by the series[18][19]

[math]\displaystyle{ \varpi=2^{-1/2}\pi\left(\sum_{n\in\mathbb{Z}}e^{-\pi n^2}\right)^2=2^{1/4}\pi e^{-\pi/12} \left(\sum_{n\in\mathbb{Z}}(-1)^n e^{-\pi p_n}\right)^2 }[/math]

where [math]\displaystyle{ p_n=(3n^2-n)/2 }[/math] (for [math]\displaystyle{ n\ge 1 }[/math], these are the pentagonal numbers)

In a spirit similar to that of the Basel problem,

[math]\displaystyle{ \sum_{z\in\mathbb{Z}[i]\setminus\{0\}}\frac{1}{z^4}=G_4(i)=\frac{\varpi ^4}{15} }[/math]

where [math]\displaystyle{ \mathbb{Z}[i] }[/math] are the Gaussian integers and [math]\displaystyle{ G_4(\tau) }[/math] is the Eisenstein series of weight [math]\displaystyle{ 4 }[/math].[20]

Gauss's constant is given by the rapidly converging series

[math]\displaystyle{ G = \sqrt[4]{32}e^{-\frac{\pi}{3}}\left (\sum_{n = -\infty}^\infty (-1)^n e^{-2n\pi(3n+1)} \right )^2. }[/math]

The constant is also given by the infinite product

[math]\displaystyle{ G = \prod_{m = 1}^\infty \tanh^2 \left( \frac{\pi m}{2}\right). }[/math]

An analog of the Wallis product is:[21]

[math]\displaystyle{ G = \prod_{n=1}^{\infty} \left(\frac{4n-1}{4n} \cdot \frac{4n+2}{4n+1}\right) = \biggl(\frac{3}{4} \cdot \frac{6}{5}\biggr) \biggl(\frac{7}{8} \cdot \frac{10}{9}\biggr) \biggl(\frac{11}{12} \cdot \frac{14}{13}\biggr) \cdots }[/math]

Gauss' constant as a continued fraction is [0, 1, 5, 21, 3, 4, 14, ...]. (sequence A053002 in the OEIS)

Integrals

A geometric representation of [math]\displaystyle{ \varpi/2 }[/math] and [math]\displaystyle{ \varpi/\sqrt{2} }[/math]

ϖ is related to the area under the curve [math]\displaystyle{ x^4 + y^4 = 1 }[/math]. Defining [math]\displaystyle{ \pi_n \mathrel{:=} \Beta\bigl(\tfrac1n, \tfrac1n \bigr) }[/math], twice the area in the positive quadrant under the curve [math]\displaystyle{ x^n + y^n = 1 }[/math] is [math]\displaystyle{ 2 \int_0^1 \sqrt[n]{1 - x^n}\mathop{\mathrm{d}x} = \tfrac1n \pi_n. }[/math] In the quartic case, [math]\displaystyle{ \tfrac14 \pi_4 = \tfrac1\sqrt{2} \varpi. }[/math]

Gauss's constant appears in the evaluation of the integrals

[math]\displaystyle{ {\frac{1}{G}} = \int_0^{\frac{\pi}{2}}\sqrt{\sin(x)}\,dx=\int_0^{\frac{\pi}{2}}\sqrt{\cos(x)}\,dx }[/math]

[math]\displaystyle{ G = \int_0^{\infty}{\frac{dx}{\sqrt{\cosh(\pi x)}}} }[/math]

The first and second lemniscate constants are defined by integrals:

[math]\displaystyle{ L = \int_0^1\frac{dx}{\sqrt{1 - x^4}} }[/math]

[math]\displaystyle{ M = \int_0^1\frac{x^2 dx}{\sqrt{1 - x^4}} }[/math]

Circumference of an ellipse

Gauss's constant satisfies the equation[22]

[math]\displaystyle{ \frac{1}{G} = 2 \int_0^1\frac{x^2\, dx}{\sqrt{1 - x^4}} }[/math]

Euler discovered in 1738 that for the rectangular elastica (first and second lemniscate constants)[23][22]

[math]\displaystyle{ \textrm{arc}\ \textrm{length}\cdot\textrm{height} = L \cdot M = \int_0^1 \frac{\mathrm{d}x}{\sqrt{1 - x^4}} \cdot \int_0^1 \frac{x^2 \mathop{\mathrm{d}x}}{\sqrt{1 - x^4}} = \frac\varpi2 \cdot \frac\pi{2\varpi} = \frac\pi4 }[/math]

Now considering the circumference [math]\displaystyle{ C }[/math] of the ellipse with axes [math]\displaystyle{ \sqrt{2} }[/math] and [math]\displaystyle{ 1 }[/math], satisfying [math]\displaystyle{ 2x^2 + 4y^2 = 1 }[/math], Stirling noted that[24]

[math]\displaystyle{ \frac{C}{2} = \int_0^1\frac{dx}{\sqrt{1 - x^4}} + \int_0^1\frac{x^2\,dx}{\sqrt{1 - x^4}} }[/math]

Hence the full circumference is

[math]\displaystyle{ C = \frac{1}{G} + G \pi \approx 3.820197789\ldots }[/math]

This is also the arc length of the sine curve on half a period:[25]

[math]\displaystyle{ C = \int_0^\pi \sqrt{1+\cos^2(x)}\,dx }[/math]

See also

  • Lemniscate elliptic function

Notes

  1. ↑ although neither of these proofs was rigorous from the modern point of view.
  2. ↑ In particular, he proved that the beta function [math]\displaystyle{ \Beta (a,b) }[/math] is transcendental for all [math]\displaystyle{ a,b\in\mathbb{Q}\setminus\mathbb{Z} }[/math] such that [math]\displaystyle{ a+b\notin \mathbb{Z}_0^- }[/math]. The fact that [math]\displaystyle{ \varpi }[/math] is transcendental follows from [math]\displaystyle{ \varpi=\tfrac{1}{2}\Beta \left(\tfrac{1}{4},\tfrac{1}{2}\right) }[/math] and similarly for M and G from [math]\displaystyle{ \Beta \left(\tfrac{1}{2},\tfrac{3}{4}\right) }[/math]

References

  1. ↑ 1.0 1.1 Finch, Steven R. (18 August 2003) (in en). Mathematical Constants. Cambridge University Press. p. 420. ISBN 978-0-521-81805-6. https://www.google.com/books/edition/Mathematical_Constants/Pl5I2ZSI6uAC?hl=en. 
  2. ↑ Kobayashi, Hiroyuki; Takeuchi, Shingo (2019), "Applications of generalized trigonometric functions with two parameters", Communications on Pure & Applied Analysis 18 (3): 1509–1521, doi:10.3934/cpaa.2019072 
  3. ↑ Asai, Tetsuya (2007), Elliptic Gauss Sums and Hecke L-values at s=1 
  4. ↑ 4.0 4.1 Todd, John (January 1975). "The lemniscate constants". Communications of the ACM 18 (1): 14–19. doi:10.1145/360569.360580. https://dl.acm.org/doi/10.1145/360569.360580. 
  5. ↑ "A085565 - Oeis". http://oeis.org/A085565. 
  6. ↑ Carlson, B. C. (2010), "Elliptic Integrals", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F. et al., NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0-521-19225-5, http://dlmf.nist.gov/19.20.E2 
  7. ↑ "A064853 - Oeis". http://oeis.org/A064853. 
  8. ↑ "Lemniscate Constant". http://www.numberworld.org/digits/Lemniscate/. 
  9. ↑ Schneider, Theodor (1941). "Zur Theorie der Abelschen Funktionen und Integrale". Journal für die reine und angewandte Mathematik 183 (19): 110–128. doi:10.1515/crll.1941.183.110. https://www.deepdyve.com/lp/de-gruyter/zur-theorie-der-abelschen-funktionen-und-integrale-mn0U50bvkB. 
  10. ↑ G. V. Choodnovsky: Algebraic independence of constants connected with the functions of analysis, Notices of the AMS 22, 1975, p. A-486
  11. ↑ G. V. Chudnovsky: Contributions to The Theory of Transcendental Numbers, American Mathematical Society, 1984, p. 6
  12. ↑ Borwein, Jonathan M.; Borwein, Peter B. (1987). Pi and the AGM: A Study in Analytic Number Theory and Computational Complexity (First ed.). Wiley-Interscience. ISBN 0-471-83138-7.  p. 45
  13. ↑ Cox 1984, p. 277.
  14. ↑ Levin (2006)
  15. ↑ Hyde (2014) proves the validity of a more general Wallis-like formula for clover curves; here the special case of the lemniscate is slightly transformed, for clarity.
  16. ↑ Bottazzini & Gray (2013), p. 60
  17. ↑ Todd (1975)
  18. ↑ Cox 1984, p. 307, eq. 2.21 for the first equality.
  19. ↑ Berndt, Bruce C. (1998). Ramanujan's Notebooks Part V. Springer. ISBN 978-1-4612-7221-2.  p. 326
  20. ↑ Eymard, Pierre; Lafon, Jean-Pierre (1999) (in French). Autour du nombre Pi. HERMANN. ISBN 2705614435.  p. 224
  21. ↑ Hyde, Trevor (2014). "A Wallis product on clovers". The American Mathematical Monthly 121 (3): 237–243. doi:10.4169/amer.math.monthly.121.03.237. https://math.uchicago.edu/~tghyde/Hyde%20--%20A%20Wallis%20product%20on%20clovers.pdf. 
  22. ↑ 22.0 22.1 Cox 1984, p. 313.
  23. ↑ Levien (2008)
  24. ↑ Cox 1984, p. 312.
  25. ↑ Adlaj, Semjon (2012). "An Eloquent Formula for the Perimeter of an Ellipse". p. 1097. https://www.ams.org/notices/201208/rtx120801094p.pdf. "One might also observe that the length of the “sine” curve over half a period, that is, the length of the graph of the function sin(t) from the point where t = 0 to the point where t = π , is [math]\displaystyle{ \sqrt{2} l(1/\sqrt{2}) = L + M }[/math]." 

External links





Retrieved from "https://handwiki.org/wiki/index.php?title=Gauss%27s_constant&oldid=2879647"