Levi-Civita connection
In Riemannian or pseudo-Riemannian geometry (in particular the Lorentzian geometry of general relativity), the Levi-Civita connection is the unique affine connection on the tangent bundle of a manifold (i.e. affine connection) that preserves the (pseudo-)Riemannian metric and is torsion-free.
The fundamental theorem of Riemannian geometry states that there is a unique connection which satisfies these properties.
In the theory of Riemannian and pseudo-Riemannian manifolds the term covariant derivative is often used for the Levi-Civita connection. The components (structure coefficients) of this connection with respect to a system of local coordinates are called Christoffel symbols.
History
The Levi-Civita connection is named after Tullio Levi-Civita, although originally "discovered" by Elwin Bruno Christoffel. Levi-Civita,[1] along with Gregorio Ricci-Curbastro, used Christoffel's symbols[2] to define the notion of parallel transport and explore the relationship of parallel transport with the curvature, thus developing the modern notion of holonomy.[3]
In 1869, Christoffel discovered that the components of the intrinsic derivative of a vector field, upon changing the coordinate system, transform as the components of a contravariant vector. This discovery was the real beginning of tensor analysis.
In 1906, L. E. J. Brouwer was the first mathematician to consider the parallel transport of a vector for the case of a space of constant curvature.[4][5]
In 1917, Levi-Civita pointed out its importance for the case of a hypersurface immersed in a Euclidean space, i.e., for the case of a Riemannian manifold embedded in a "larger" ambient space.[1] He interpreted the intrinsic derivative in the case of an embedded surface as the tangential component of the usual derivative in the ambient affine space. The Levi-Civita notions of intrinsic derivative and parallel displacement of a vector along a curve make sense on an abstract Riemannian manifold, even though the original motivation relied on a specific embedding [math]\displaystyle{ M^n \subset \mathbf{R}^{n(n+1)/2}. }[/math]
In 1918, independently of Levi-Civita, Jan Arnoldus Schouten obtained analogous results.[6] In the same year, Hermann Weyl generalized Levi-Civita's results.[7][8]
Notation
- (M, g) denotes a Riemannian or pseudo-Riemannian manifold.
- TM is the tangent bundle of M.
- g is the Riemannian or pseudo-Riemannian metric of M.
- X, Y, Z are smooth vector fields on M, i. e. smooth sections of TM.
- [X, Y] is the Lie bracket of X and Y. It is again a smooth vector field.
The metric g can take up to two vectors or vector fields X, Y as arguments. In the former case the output is a number, the (pseudo-)inner product of X and Y. In the latter case, the inner product of Xp, Yp is taken at all points p on the manifold so that g(X, Y) defines a smooth function on M. Vector fields act (by definition) as differential operators on smooth functions. In local coordinates [math]\displaystyle{ (x_1,\ldots, x_n) }[/math], the action reads
- [math]\displaystyle{ X(f) = X^i\frac{\partial}{\partial x^i}f = X^i\partial_i f }[/math]
where Einstein's summation convention is used.
Formal definition
An affine connection [math]\displaystyle{ \nabla }[/math] is called a Levi-Civita connection if
- it preserves the metric, i.e., [math]\displaystyle{ \nabla g = 0 }[/math].
- it is torsion-free, i.e., for any vector fields [math]\displaystyle{ X }[/math] and [math]\displaystyle{ Y }[/math] we have [math]\displaystyle{ \nabla_X Y - \nabla_Y X = [X,Y] }[/math], where [math]\displaystyle{ [X, Y] }[/math] is the Lie bracket of the vector fields [math]\displaystyle{ X }[/math] and [math]\displaystyle{ Y }[/math].
Condition 1 above is sometimes referred to as compatibility with the metric, and condition 2 is sometimes called symmetry, cf. Do Carmo's text.[9]
Fundamental theorem of (pseudo) Riemannian Geometry
Theorem Every pseudo Riemannian manifold [math]\displaystyle{ (M,g) }[/math] has a unique Levi Civita connection [math]\displaystyle{ \nabla }[/math].
proof: If a Levi-Civita connection exists, it must be unique. To see this, unravel the definition of the action of a connection on tensors to find
- [math]\displaystyle{ X\bigl(g(Y,Z)\bigr) = (\nabla_X g)(Y, Z) + g(\nabla_X Y, Z) + g( Y, \nabla_X Z). }[/math]
Hence we can write condition 1 as
- [math]\displaystyle{ X\bigl(g(Y,Z)\bigr) = g(\nabla_X Y, Z) + g( Y, \nabla_X Z). }[/math]
By the symmetry of the metric tensor [math]\displaystyle{ g }[/math] we then find:
- [math]\displaystyle{ X \bigl(g(Y,Z)\bigr) + Y \bigl(g(Z,X)\bigr) - Z \bigl(g(Y,X)\bigr) = g(\nabla_X Y + \nabla_Y X, Z) + g(\nabla_X Z - \nabla_Z X, Y) + g(\nabla_Y Z - \nabla_Z Y, X). }[/math]
By condition 2, the right hand side is therefore equal to
- [math]\displaystyle{ 2g(\nabla_X Y, Z) - g([X,Y], Z) + g([X,Z], Y) + g([Y,Z], X), }[/math]
and we find the Koszul formula
- [math]\displaystyle{ g(\nabla_X Y, Z) = \tfrac{1}{2} \Big\{ X \bigl(g(Y,Z)\bigr) + Y \bigl(g(Z,X)\bigr) - Z \bigl(g(X,Y)\bigr) + g([X,Y],Z) - g([Y,Z], X) - g([X,Z], Y) \Big\}. }[/math]
Hence, if a Levi-Civita connection exists, it must be unique, because [math]\displaystyle{ Z }[/math] is arbitrary, [math]\displaystyle{ g }[/math] is non degenerate, and the right hand side does not depend on [math]\displaystyle{ \nabla }[/math].
To prove existence, note that for given vector field [math]\displaystyle{ X }[/math] and [math]\displaystyle{ Y }[/math], the right hand side of the Koszul expression is function-linear in the vector field [math]\displaystyle{ Z }[/math], not just real linear. Hence by the non degeneracy of [math]\displaystyle{ g }[/math], the right hand side uniquely defines some new vector field which we suggestively denote [math]\displaystyle{ \nabla_X Y }[/math] as in the left hand side. By substituting the Koszul formula, one now checks that for all vector fields [math]\displaystyle{ X, Y,Z }[/math], and all functions [math]\displaystyle{ f }[/math]
- [math]\displaystyle{ g(\nabla_X (Y_1 + Y_2), Z) = g(\nabla_X Y_1, Z) + g(\nabla_X Y_2 , Z) }[/math]
- [math]\displaystyle{ g(\nabla_X (f Y), Z) = X(f) g(Y, Z) + f g(\nabla_X Y,Z) }[/math]
- [math]\displaystyle{ g(\nabla_X Y, Z) + g(\nabla_X Z, Y) = X\bigl(g(Y,Z)\bigr) }[/math]
- [math]\displaystyle{ g(\nabla_X Y, Z) - g(\nabla_Y X, Z) = g([X,Y], Z). }[/math]
Hence the Koszul expression does, in fact, define a connection, and this connection is compatible with the metric and is torsion free, i.e. is a (hence the) Levi-Civita connection.
Note that with minor variations the same proof shows that there is a unique connection that is compatible with the metric and has prescribed torsion.
Christoffel symbols
Let [math]\displaystyle{ \nabla }[/math] be an affine connection on the tangent bundle. Choose local coordinates [math]\displaystyle{ x^1, \ldots, x^n }[/math] with coordinate basis vector fields [math]\displaystyle{ \partial_1, \ldots, \partial_n }[/math] and write [math]\displaystyle{ \nabla_j }[/math] for [math]\displaystyle{ \nabla_{\partial_j} }[/math]. The Christoffel symbols [math]\displaystyle{ \Gamma^l_{jk} }[/math] of [math]\displaystyle{ \nabla }[/math] with respect to these coordinates are defined as
- [math]\displaystyle{ \nabla_j\partial_k = \Gamma^l_{jk} \partial_l }[/math]
The Christoffel symbols conversely define the connection [math]\displaystyle{ \nabla }[/math] on the coordinate neighbourhood because
- [math]\displaystyle{ \begin{align} \nabla_X Y &= \nabla_{X^j\partial_j} (Y^k \partial_k) \\&= X^j\nabla_j(Y^k\partial_k) \\ &= X^j\bigl(\partial_j(Y^k)\partial_k + Y^k\nabla_j\partial_k\bigr) \\ &= X^j\bigl(\partial_j(Y^k)\partial_k + Y^k\Gamma^l_{jk}\partial_l\bigr) \\ &= X^j\bigl(\partial_j(Y^l) + Y^k\Gamma^l_{jk}\bigr)\partial_l \end{align} }[/math]
that is,
- [math]\displaystyle{ (\nabla_j Y)^l = \partial_jY^l + \Gamma^l_{jk} Y^k }[/math]
An affine connection [math]\displaystyle{ \nabla }[/math] is compatible with a metric iff
- [math]\displaystyle{ \partial_i \bigl(g(\partial_j, \partial_k) \bigr) = g(\nabla_i\partial_j, \partial_k) + g(\partial_j, \nabla_i\partial_k) = g(\Gamma^l_{ij}\partial_l, \partial_k) + g(\partial_j, \Gamma_{ik}^l\partial_l) }[/math]
i.e., if and only if
- [math]\displaystyle{ \partial_i g_{jk} = \Gamma^l_{ij}g_{lk} + \Gamma^l_{ik}g_{jl}. }[/math]
An affine connection ∇ is torsion free iff
- [math]\displaystyle{ \nabla_j\partial_k - \nabla_k \partial_j = (\Gamma^l_{jk} - \Gamma^l_{kj})\partial_l = [\partial_j, \partial_k]= 0. }[/math]
i.e., if and only if
- [math]\displaystyle{ \Gamma^l_{jk} = \Gamma^l_{kj} }[/math]
is symmetric in its lower two indices.
As one checks by taking for [math]\displaystyle{ X, Y, Z }[/math], coordinate vector fields [math]\displaystyle{ \partial_j, \partial_k, \partial_l }[/math] (or computes directly), the Koszul expression of the Levi-Civita connection derived above is equivalent to a definition of the Christoffel symbols in terms of the metric as
- [math]\displaystyle{ \Gamma^l_{jk} = \tfrac{1}{2} g^{lr} \left( \partial _k g_{rj} + \partial _j g_{rk} - \partial _r g_{jk} \right) }[/math]
where as usual [math]\displaystyle{ g^{ij} }[/math] are the coefficients of the dual metric tensor, i.e. the entries of the inverse of the matrix [math]\displaystyle{ g_{kl} }[/math].
Derivative along curve
The Levi-Civita connection (like any affine connection) also defines a derivative along curves, sometimes denoted by D.
Given a smooth curve γ on (M, g) and a vector field V along γ its derivative is defined by
- [math]\displaystyle{ D_tV=\nabla_{\dot\gamma(t)}V. }[/math]
Formally, D is the pullback connection γ*∇ on the pullback bundle γ*TM.
In particular, [math]\displaystyle{ \dot\gamma(t) }[/math] is a vector field along the curve γ itself. If [math]\displaystyle{ \nabla_{\dot{\gamma}(t)}\dot{\gamma}(t) }[/math] vanishes, the curve is called a geodesic of the covariant derivative. Formally, the condition can be restated as the vanishing of the pullback connection applied to [math]\displaystyle{ \dot\gamma }[/math]:
- [math]\displaystyle{ \left(\gamma^*\nabla\right) \dot{\gamma}\equiv 0. }[/math]
If the covariant derivative is the Levi-Civita connection of a certain metric, then the geodesics for the connection are precisely those geodesics of the metric that are parametrised proportionally to their arc length.
Parallel transport
In general, parallel transport along a curve with respect to a connection defines isomorphisms between the tangent spaces at the points of the curve. If the connection is a Levi-Civita connection, then these isomorphisms are orthogonal – that is, they preserve the inner products on the various tangent spaces.
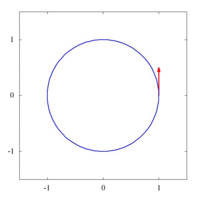
The images below show parallel transport of the Levi-Civita connection associated to two different Riemannian metrics on the plane, expressed in polar coordinates. The metric of left image corresponds to the standard Euclidean metric [math]\displaystyle{ ds^2 = dx^2 + dy^2 = dr^2 + r^2 d\theta^2 }[/math], while the metric on the right has standard form in polar coordinates (when [math]\displaystyle{ r = 1 }[/math]), and thus preserves the vector [math]\displaystyle{ {\partial \over \partial \theta} }[/math] tangent to the circle. This second metric has a singularity at the origin, as can be seen by expressing it in Cartesian coordinates:
- [math]\displaystyle{ dr = \frac{xdx + ydy}{\sqrt{x^2 + y^2}} }[/math]
- [math]\displaystyle{ d\theta = \frac{xdy - ydx}{x^2 + y^2} }[/math]
- [math]\displaystyle{ dr^2 + d\theta^2 = \frac{(xdx + ydy)^2}{x^2+y^2} + \frac{(xdy - ydx)^2}{(x^2+y^2)^2} }[/math]
Example: the unit sphere in R3
Let ⟨ , ⟩ be the usual scalar product on R3. Let S2 be the unit sphere in R3. The tangent space to S2 at a point m is naturally identified with the vector subspace of R3 consisting of all vectors orthogonal to m. It follows that a vector field Y on S2 can be seen as a map Y : S2 → R3, which satisfies [math]\displaystyle{ \bigl\langle Y(m), m\bigr\rangle = 0, \qquad \forall m\in \mathbf{S}^2. }[/math]
Denote as dmY the differential of the map Y at the point m. Then we have:
Lemma — The formula [math]\displaystyle{ \left(\nabla_X Y\right)(m) = d_mY(X(m)) + \langle X(m),Y(m)\rangle m }[/math] defines an affine connection on S2 with vanishing torsion.
It is straightforward to prove that ∇ satisfies the Leibniz identity and is C∞(S2) linear in the first variable. It is also a straightforward computation to show that this connection is torsion free. So all that needs to be proved here is that the formula above produces a vector field tangent to S2. That is, we need to prove that for all m in S2 [math]\displaystyle{ \bigl\langle\left(\nabla_X Y\right)(m),m\bigr\rangle = 0\qquad (1). }[/math] Consider the map f that sends every m in S2 to ⟨Y(m), m⟩, which is always 0. The map f is constant, hence its differential vanishes. In particular [math]\displaystyle{ d_mf(X) = \bigl\langle d_m Y(X),m\bigr\rangle + \bigl\langle Y(m), X(m)\bigr\rangle = 0. }[/math] The equation (1) above follows. Q.E.D.
In fact, this connection is the Levi-Civita connection for the metric on S2 inherited from R3. Indeed, one can check that this connection preserves the metric.
Behavior under conformal rescaling
If the metric [math]\displaystyle{ g }[/math] in a conformal class is replaced by the conformally rescaled metric of the same class [math]\displaystyle{ \hat g=e^{2\gamma}g }[/math], then the Levi-Civita connection transforms according to the rule[10] [math]\displaystyle{ \widehat\nabla_X Y = \nabla_XY + X(\gamma)Y + Y(\gamma)X - g(X,Y)\mathrm{grad}_g(\gamma). }[/math] where [math]\displaystyle{ \mathrm{grad}_g(\gamma) }[/math] is the gradient vector field of [math]\displaystyle{ \gamma }[/math] i.e. the vector field [math]\displaystyle{ g }[/math]-dual to [math]\displaystyle{ d\gamma }[/math], in local coordinates given by [math]\displaystyle{ g^{ik}(\partial_i \gamma)\partial_k }[/math]. Indeed, it is trivial to verify that [math]\displaystyle{ \widehat\nabla }[/math] is torsion-free. To verify metricity, assume that [math]\displaystyle{ g(Y,Y) }[/math] is constant. In that case, [math]\displaystyle{ \hat g(\widehat\nabla_XY,Y) = X(\gamma)\hat g(Y,Y) = \frac12 X(\hat g(Y,Y)). }[/math]
As an application, consider again the unit sphere, but this time under stereographic projection, so that the metric (in complex Fubini–Study coordinates [math]\displaystyle{ z,\bar z }[/math]) is: [math]\displaystyle{ g = \frac{4\,dz\,d\bar z}{(1+z\bar z)^2}. }[/math] This exhibits the metric of the sphere as conformally flat, with the Euclidean metric [math]\displaystyle{ dz\,d\bar z }[/math], with [math]\displaystyle{ \gamma = \ln(2)-\ln (1+z\bar z) }[/math]. We have [math]\displaystyle{ d\gamma = -(1+z\bar z)^{-1}(\bar z\, dz + z\,d{\bar z}) }[/math], and so [math]\displaystyle{ \widehat\nabla_{\partial_z}\partial_z = -\frac{2\bar z\partial_z}{1+z\bar z}. }[/math] With the Euclidean gradient [math]\displaystyle{ \mathrm{grad}_{Euc}(\gamma) = -(1+z\bar z)^{-1}(\bar z\partial_z + z\partial_{\bar z}) }[/math], we have [math]\displaystyle{ \widehat\nabla_{\partial_z}\partial_{\bar z} = 0. }[/math] These relations, together with their complex conjugates, define the Christoffel symbols for the two-sphere.
See also
- Weitzenböck connection
Notes
- ↑ 1.0 1.1 "Nozione di parallelismo in una varietà qualunque" (in it). Rendiconti del Circolo Matematico di Palermo 42: 173–205. 1917. doi:10.1007/BF03014898. https://zenodo.org/record/1428456.
- ↑ Christoffel, Elwin B. (1869). "Ueber die Transformation der homogenen Differentialausdrücke zweiten Grades". Journal für die reine und angewandte Mathematik 1869 (70): 46–70. doi:10.1515/crll.1869.70.46. http://gdz.sub.uni-goettingen.de/dms/load/img/?PPN=GDZPPN002153882&IDDOC=266356.
- ↑ See Spivak, Michael (1999). A Comprehensive introduction to differential geometry (Volume II). Publish or Perish Press. p. 238. ISBN 0-914098-71-3.
- ↑ "Het krachtveld der niet-Euclidische, negatief gekromde ruimten". Koninklijke Akademie van Wetenschappen. Verslagen 15: 75–94. 1906.
- ↑ "The force field of the non-Euclidean spaces with negative curvature". Koninklijke Akademie van Wetenschappen. Proceedings 9: 116–133. 1906. Bibcode: 1906KNAB....9..116B.
- ↑ "Die direkte Analysis zur neueren Relativiteitstheorie". Verhandelingen der Koninklijke Akademie van Wetenschappen te Amsterdam 12 (6): 95. 1918.
- ↑ "Gravitation und Elektrizitat". Sitzungsberichte Berliner Akademie: 465–480. 1918.
- ↑ "Reine Infinitesimal geometrie". Mathematische Zeitschrift 2 (3–4): 384–411. 1918. doi:10.1007/bf01199420. Bibcode: 1918MatZ....2..384W. https://zenodo.org/record/1447411.
- ↑ Carmo, Manfredo Perdigão do (1992). Riemannian geometry. Francis J. Flaherty. Boston: Birkhäuser. ISBN 0-8176-3490-8. OCLC 24667701. https://www.worldcat.org/oclc/24667701.
- ↑ Arthur Besse (1987). Einstein manifolds. Springer. p. 58.
References
- Boothby, William M. (1986). An introduction to differentiable manifolds and Riemannian geometry. Academic Press. ISBN 0-12-116052-1. https://archive.org/details/introductiontodi0000boot.
- Kobayashi, Shoshichi; Nomizu, Katsumi (1963). Foundations of differential geometry. John Wiley & Sons. ISBN 0-470-49647-9. See Volume I pag. 158
External links
- Hazewinkel, Michiel, ed. (2001), "Levi-Civita connection", Encyclopedia of Mathematics, Springer Science+Business Media B.V. / Kluwer Academic Publishers, ISBN 978-1-55608-010-4, https://www.encyclopediaofmath.org/index.php?title=p/l058230
- MathWorld: Levi-Civita Connection
- PlanetMath: Levi-Civita Connection
- Levi-Civita connection at the Manifold Atlas
 |